Simple ising model: Magnetic susceptibility derivation

[randomwalk]

I'm stuck on a question about deriving an expression for the magnetic susceptibility in terms of the variance of the magnetisation for a simple 2d square ising model.

I get the derivation of the specific heat, and I know am supposed to do something similar to get to the expression for susceptibility d<M>/dH. But how? Any help would be much appreciated.

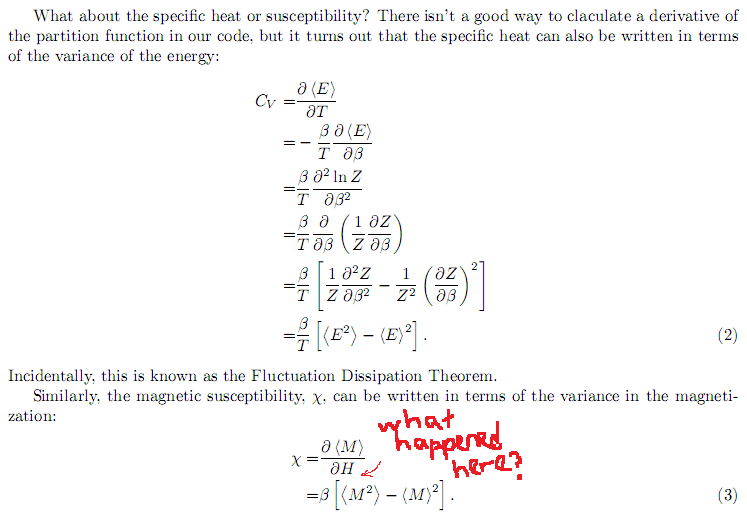
[beta is 1/KT]

 

[Mute]

Well, take a look at the partition function for the Ising Model and what the average of some quantity A is:

Z = \sum_{\{S_i\}}\exp\left[K\sum_{&lt;i,j&gt;}S_iS_j + \beta H \sum_i S_i \right]

\langle A \rangle = Z^{-1}\ \sum_{\{S_i\}}A\exp\left[K\sum_{&lt;i,j&gt;}S_iS_j + \beta H \sum_i S_i \right]

Let the magnetization be M = \sum_i S_i . Note that if you take a derivative of Z with respect to H it brings down the sum over the S_i, so you get the average of M times Z. If you take two derivatives you get the average of M^2 times Z (up to some betas). Compare to the derivative of the average of M with respect to H to get the relation your source claims.