- #1
michaelwoodco
- 28
- 0
Ok so I got in a HUGE argument with some people over on a car forum. They say that cars with longer wheelbases are harder to wheelie. I disagreed. I said it not only depends on the wheel base since that is an oversimplified way to look at it, it depends on where the center of gravity is in relation to the rear axle and the contact patch.
I believe you can calculate the amount of forward thrust a car can handle without doing a wheelie by looking at it this way:
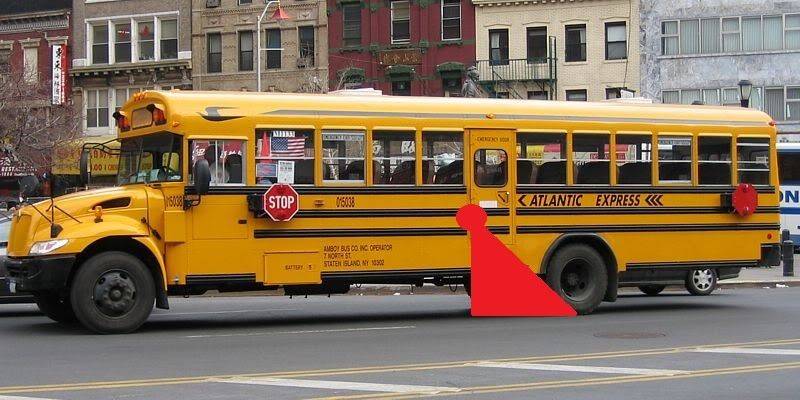
the red dot is it's center of gravity, pulling down, providing a torque on the axle. Whereas the horizontal line is the distance from the contact patch to a vertical line drawn through the COG. I believe that if you use a similar triangle it could enter a wheel stand when the bottom leg's value is great enough that the other vertical leg would have a value that exceeds the weight of the vehicle.
Anyways any comments, advice, or equations on this?
Thanks,
Michael Woodcock
I believe you can calculate the amount of forward thrust a car can handle without doing a wheelie by looking at it this way:
the red dot is it's center of gravity, pulling down, providing a torque on the axle. Whereas the horizontal line is the distance from the contact patch to a vertical line drawn through the COG. I believe that if you use a similar triangle it could enter a wheel stand when the bottom leg's value is great enough that the other vertical leg would have a value that exceeds the weight of the vehicle.
Anyways any comments, advice, or equations on this?
Thanks,
Michael Woodcock