Cart sliding along horizontal with spring connected

[ChiralSuperfields]

Homework Statement

Please see below

Relevant Equations

Please see below

For this problem,

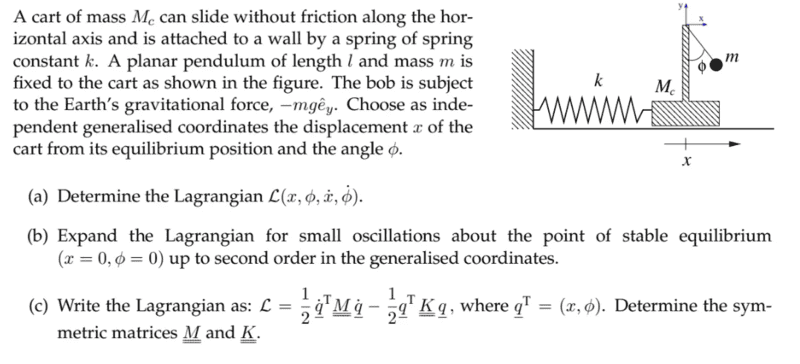
My working for (c) is


$\begin{aligned} & L=\frac{1}{2} M \dot{x}^2+\frac{1}{2} m\left(x^2+2 \dot{x} l \dot{\phi} \cos \phi+l^2 \dot{\phi}^2\right)+m g l \cos \phi-\frac{1}{2} k x^2 \\ \end{aligned}$
$L =\frac{1}{2} M \dot{x}^2+\frac{1}{2} m\left(\dot{x}^2+2 \dot{x} l \dot{\phi}-\dot{x} l \dot{\phi} {\phi}^2+l^2 \dot{\phi}^2\right)+m g l-\frac{m g l \phi^2}{2}-\frac{1}{2} k x^2$ using small angle approximation for cosine.

Then taking partial deratives for the Lagrange equation of phi and x.

I get the following equations

\begin{aligned}
& \left(m l-\frac{1}{2} m l \phi^2\right) \ddot{x}+m l^2 \ddot{\phi}=-m g l \phi \\
& (M+m) \ddot{x}+\left(m l-\frac{1}{2} m l \phi^2\right) \ddot{\phi}=-k x+m l \dot{\phi}^2 \phi \\
&
\end{aligned}


Then writing the equations in matrix form $M \ddot{x} = -kx$, I cannot find a symmetric matrix for K. Only for M.

Does anybody please agree that the problem has a mistake that it is impossible for find a symmetric matrix for K?

Thanks!

 

[haruspex]

First, I think you can throw away the mgl term. Just define the zero PE differently.

I don't understand why you differentiated. Part c does not involve second derivatives.

I can't see how the target equation can accommodate a $\dot\phi\phi^2$ term. It looks like the author did not keep the second order term there. I cannot think of a justification for that.

 

[ChiralSuperfields]

First, I think you can throw away the mgl term. Just define the zero PE differently.

I don't understand why you differentiated. Part c does not involve second derivatives.

I can't see how the target equation can accommodate a $\dot\phi\phi^2$ term. It looks like the author did not keep the second order term there. I cannot think of a justification for that.

Thank you for your reply @haruspex! T

So are you please saying that

\begin{aligned}
& (M+m) \ddot{x}+\left(m l-\frac{1}{2} m l \phi^2\right) \ddot{\phi}=-k x\\
&
\end{aligned}

?

It does seem to give a symmetric matrix for K. However, I don't like fudging that :(. I spent many hours to try to find a algebraic mistake I had made, however, there is certainly not mistake. The question must be wrong then I think since K is not a symmetric matrix without fudging the term.

In my working, I defined zero PE at the point where the string connects the pendulum to the cart tip.

This gives $x_m = x + l\sin\phi$, $y_m = -l\cos\phi$, $x_M = x$, $y_M = c$

Thanks!

 

[haruspex]

Thank you for your reply @haruspex!

So you are saying that I

\begin{aligned}
& (M+m) \ddot{x}+\left(m l-\frac{1}{2} m l \phi^2\right) \ddot{\phi}=-k x\\
&
\end{aligned}

?

Thanks!

No, I am saying that if you take your equation

$L =\frac{1}{2} M \dot{x}^2+\frac{1}{2} m\left(\dot{x}^2+2 \dot{x} l \dot{\phi}-\dot{x} l \dot{\phi} {\phi}^2+l^2 \dot{\phi}^2\right)+m g l-\frac{m g l \phi^2}{2}-\frac{1}{2} k x^2$

then throw away the $mgl$ (which is defensible) and the $\dot xl\dot\phi\phi^2$ term (which I do not see how to defend) then you can get straight to the form asked for without differentiation.

 

[ChiralSuperfields]

No, I am saying that if you take your equation

then throw away the $mgl$ (which is defensible) and the $\dot xl\dot\phi\phi^2$ term (which I do not see how to defend) then you can get straight to the form asked for without differentiation.

Thank you for your reply @haruspex!

That is interesting. I did not see that. The method I went about it was using the Euler-Lagrange equation to the get EOMs. However, using the Euler-Lagrange equation method, would it be the same sort of justification for removing that term I talked about (since it is really the same term, however, undergone some partial differentiation changing it form slightly).

Thanks!

 

[Orodruin]

I can't see how the target equation can accommodate a ϕ˙ϕ2 term. It looks like the author did not keep the second order term there. I cannot think of a justification for that.

It is not a second order term. It is multiplied by $\dot\phi$, making it third order.

@ChiralSuperfields As I said in another recent thread, if you want the linearized equations of motion you need to keep terms up to second order in the Lagrangian. This is not the same thing as always keeping two terms of cos(small number). In particular when the cosine is multiplied by something wich is not zeroth order. You have kept a term at third order, ruining the linear EoM.

 

[ChiralSuperfields]

It is not a second order term. It is multiplied by $\dot\phi$, making it third order.

@ChiralSuperfields As I said in another recent thread, if you want the linearized equations of motion you need to keep terms up to second order in the Lagrangian. This is not the same thing as always keeping two terms of cos(small number). In particular when the cosine is multiplied by something wich is not zeroth order. You have kept a term at third order, ruining the linear EoM.

Thank you for your reply @Orodruin!

Oh ok, I think I understand now. However, do you please know how $ϕ˙ϕ^2$ is third order? I've never seen anybody comment in a textbook about the order of a term multiplied by a another variable other than itself or constants which ain't variables.

Thanks!

 

[Orodruin]

Both $\phi$ and its derivatives are proportional to the amplitude. This makes that term third order in the amplitude.

 

[ChiralSuperfields]

Both $\phi$ and its derivatives are proportional to the amplitude. This makes that term third order in the amplitude.

Thank you for reply @Orodruin!

That is interesting that you are thinking in terms of the what the variables are composed of. Now I am interested in generalizing to any dependent variable that we don't know it is composed of so could be a function of any independent variable.

Do you please know how we could generalize this to any variable $x$ to any integer $m ≥ 0$ and $n ≥ 0$. i.e

Do you please know whether

$x^{(n)}x^{(m)}$ is of order $m + n$ where the brackets denote the nth and mth derivative respectively?

Thanks!

 

[Orodruin]

Do you please know whether

x(n)x(m) is of order m+n where the brackets denote the nth and mth derivative respectively?

It would be of second order. You do not get higher orders of the amplitude by differentiating. Just count the number of times you have a multiple of the variable - differentiated or not.

 

[ChiralSuperfields]

It would be of second order. You do not get higher orders of the amplitude by differentiating. Just count the number of times you have a multiple of the variable - differentiated or not.

Thank you for your reply @Orodruin!

Oh I think I see where I was getting confused because in ODE the order is the highest derivative in the ODE. However, here the order is you are referring to is the that of a Taylor polynomial, which is the number of times the variable (derivative or not) is multiplied to each other in a given term.

So if variables such as $x''$, $y^2$ and $z^3$ are multiplied together to form $x''y^2z^3$ then the term is of 1 + 2 + 3 = 6th order, is this please correct?

Thanks!

 

[haruspex]

Both $\phi$ and its derivatives are proportional to the amplitude.

Thanks, that's what I was missing.