Conservation of dot product with parallel transport

[fab13]

Hello, I have 2 questions regarding similar issues :

1*)

Why does one say that parallel transport preserves the value of dot product (scalar product) between the transported vector and the tangent vector ?

Is it due to the fact that angle between the tangent vector and transported vector is always the same during the operation of transport (which is the definition of parallel transport) ?

2*) From the following link http://www.physics.usu.edu/Wheeler/GenRel2013/Notes/Geodesics.pdf and more especially the following extract ;

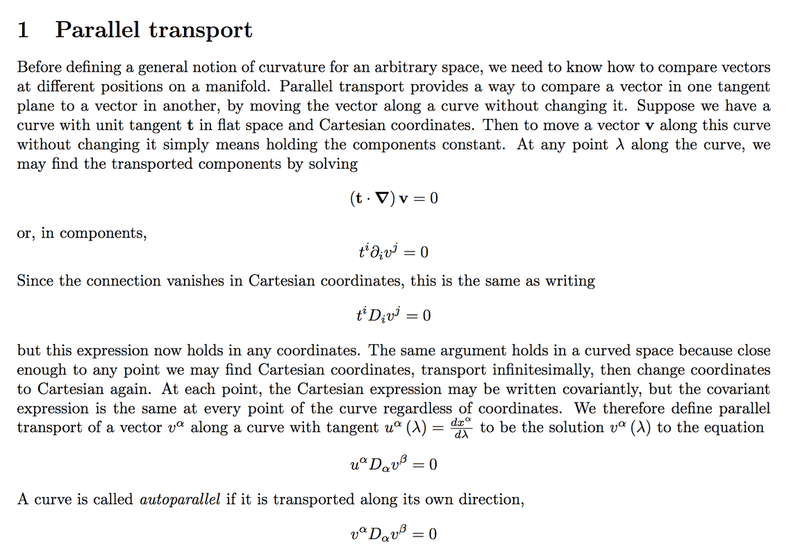
I don't understand the first relation, i.e : $$(\mathbf{t} \cdot \mathbf{\nabla})\,\mathbf{v}=0\quad\quad(1)$$

which actually is equal to : $$t^{i}\partial_{i}v^{j}=0\quad\quad(2)$$

How can we demonstrate the equation (1) above ?

Is there a link with my first question, i.e it relates to the conservation of dot product value between $$\mathbf{t}$$ tangent vector and $$\mathbf{v}$$ vector ?

Thanks for your help

 

[andrewkirk]

How can we demonstrate the equation (1) above ?

That is part of the definition of parallel transport, so it doesn't need to be demonstrated. If it doesn't hold then the vector field $\mathbf v$ is not a parallel transport.

What does need to be demonstrated is that a consequence of that definition is that the values of the dot product between the tangent and the transported vector are the same at any two points along the curve - what you describe as 'conservation of the dot product'. Integration is required to demonstrate that.

 

[fab13]

That is part of the definition of parallel transport, so it doesn't need to be demonstrated. If it doesn't hold then the vector field $\mathbf v$ is not a parallel transport.

What does need to be demonstrated is that a consequence of that definition is that the values of the dot product between the tangent and the transported vector are the same at any two points along the curve - what you describe as 'conservation of the dot product'. Integration is required to demonstrate that.

ok, I have found why equation (1) has this expression. Taking $\tau$ an affine parameter, we can write

$$ t^{i}\partial_{i}v^{j} = \dfrac{\text{d}x^{i}}{\text{d}\tau}\,\dfrac{\partial v^{j}}{\partial x^{i}} = \dfrac{\text{d} v^{j}}{\text{d}\tau}\quad\quad (2) $$

So in flat space (i.e Euclidean), the straight line parameterized by $\tau$ and on which the vector $\vec{v}=v^{i}\,\vec{e_{i}}$ is transported, makes vanish the expression $\dfrac{\text{d} v^{j}}{\text{d}\tau}$ because the components doesn't vary during the transport.

Now, I have to generalize this Euclidean example to Curved space and undertsand in this case the using of Covariant Derivative which replaces the expression above $(2)$ with :

$$\dfrac{D\,v^{j}}{\text{d}\tau}=\dfrac{\text{d}x^{i}}{\text{d}\tau}\,\nabla_{i} v^{j}=t^{i} \,\nabla_{i} v^{j}=0$$ with $t^{i}=\dfrac{\text{d}x^{i}}{\text{d}\tau}$

Thanks

 

[Orodruin]

I suggest starting by transforming the Euclidean two-dimensional case to polar coordinates.

 

[fab13]

What do you mean ? The using of polar coordinates in 2D classic space is still a flat space, geodesics are still straight lines, I should rather start by the surface of a 2D sphere, shoudn't I ?

 

[Orodruin]

What do you mean ? The using of polar coordinates in 2D classic space is still a flat space, geodesics are still straight lines, I should rather start by the surface of a 2D sphere, shoudn't I ?

You are aware that even a flat space have non-zero Christoffel symbols in curvilinear coordinates, no? You should start with polar coordinates and check the form of the resulting equations for the parallel transported vector components. The property you are talking about is not particular for a curved space.

Edit: In fact, this is exactly what the text you quoted does. Starts from a Cartesian coordinate system and then uses that the partial and covariant derivatives are the same in that system to rewrite it on a covariant form in an arbitrary system.

 

[fab13]

Orodruin,

as you said, I have computed the equations for parallel transport vector, I get :

$$D A^{\rho}=dA^{\rho}-\rho\,A^{\theta}\,\text{d}\theta$$
and
$$D A^{\theta}=dA^{\theta}+\dfrac{1}{\rho}(A^{\rho}\,\text{d}\theta+A^{\theta}\,\text{d}\rho)$$

By definition of parallel transport, I can write : $D A^{\rho}=D A^{\theta}=0$

What can I conclude about the property that I am looking for, i.e the conservation of dot product ?

Thanks

 

[Orodruin]

What can I conclude about the property that I am looking for, i.e the conservation of dot product ?

For that you need to take the directional derivative of the dot product of the parallel transported fields along the curve taking into account that the vector components satisfy the parallel transport equations.

 

[fab13]

For that you need to take the directional derivative of the dot product of the parallel transported fields along the curve taking into account that the vector components satisfy the parallel transport equations.

I didn't understand what you mean, do I have to start with :

$$\text{d}(\vec{A}\cdot\vec{n}) = \text{d}(A_{i}\dfrac{\text{d}y^{i}}{\text{d}\tau})=0$$

??

 

[fab13]

For that you need to take the directional derivative of the dot product of the parallel transported fields along the curve taking into account that the vector components satisfy the parallel transport equations.

Dear Orodruin,

could you help me about what you told me to do, i.e take the directional derivative of the dot product of the parallel and demonstrate the conservation.

Thanks