Linear first-order differential equation with an initial condition

[Lambda96]

Homework Statement

Show that the solution of a linear differential equation with boundary condition $f(0)=f_0$ has the following form $f(x)=f_0 exp\biggl( \int_{0}^{x}ds ~g(s) \biggr)+\int_{0}^{x}ds ~h(s)exp\biggl( \int_{s}^{x}dr ~g(r)\biggr)$

Relevant Equations

none

Hi,

unfortunately I have problems with the task d and e, the complete task is as follows:

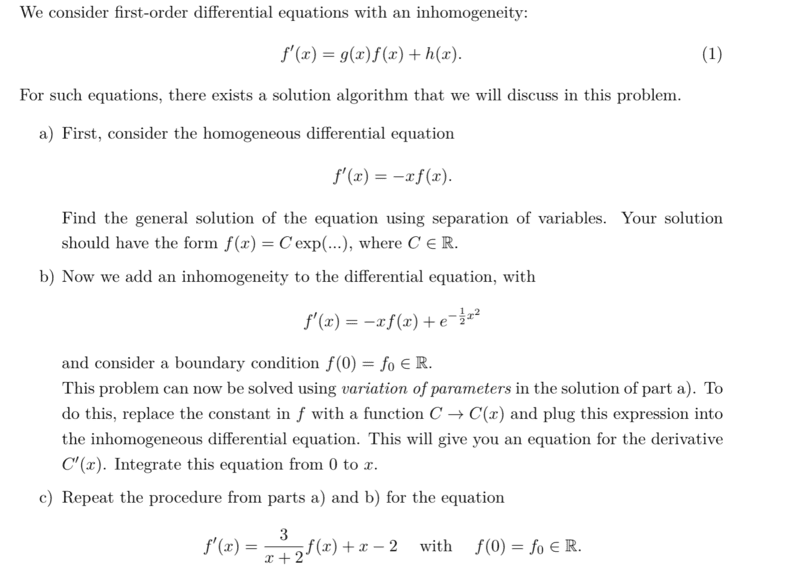
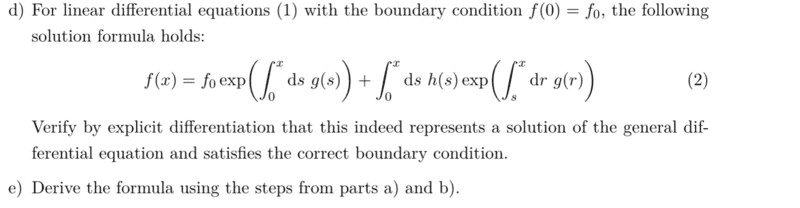
I tried to form the derivative of the equation $f(x)$, but unfortunately I have problems with the second part, which is why I only got the following.

$$\frac{d f(x)}{dx}=f_0 g(x) \ exp\biggl( \int_{0}^{x}ds \ g(s) \biggr)+?$$

I then tried another approach and first wanted to get rid of the integrals in the exp terms

$$f_0 exp\biggl( G(x)-G(0) \biggr)+\int_{0}^{x}ds \ h(s) exp \biggl( G(x)-G(s) \biggr) f(x)dx$$
$$f_0 e^{G(x)-G(0)}+e^{G(x)}\int_{0}^{x}ds \ h(s) e^{-G(s)}$$

Unfortunately, I now have problems again with the derivative for the second term using this method.

 

[pasmith]

You have an expression of the form $$\int_a^x f(x,t)\,dt$$ for which you can show from the limit definition that, for sufficiently smooth $f$, $$\frac{d}{dx} \int_a^x f(x,t)\,dt = \lim_{h \to 0} \frac 1h \left( \int_a^{x+h} f(x+h, t)\,dt - \int_a^x f(x,t)\,dt\right) = f(x,x) + \int_a^x \left.\frac{\partial f}{\partial x}\right|_{(x,t)}\,dt.$$

 

[Lambda96]

Thanks pasmith for your help , I tried to implement your tip but am not sure about the $f(x,x)$ term:

I have now calculated the following

$$\frac{d f(x)}{dx}=\frac{d}{dx}\biggl( f_0 \ exp\Bigl( \int_{0}^{x}ds \ g(s) \Bigr) +\int_{0}^{x}ds \ h(s) \ exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr) \biggr)$$

$$\frac{d f(x)}{dx}= f_0 \ exp\Bigl( \int_{0}^{x}ds \ g(s) \Bigr) g(x) + h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)+\int_{0}^{x}ds \ h(s) \frac{d}{dx} \ exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)$$

$$\frac{d f(x)}{dx}= f_0 \ exp\Bigl( \int_{0}^{x}ds \ g(s) \Bigr) g(x) + h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)+\int_{0}^{x}ds \ h(s) exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)g(x)$$

$$\frac{d f(x)}{dx}= f_0 \ exp\Bigl( \int_{0}^{x}ds \ g(s) \Bigr) g(x) + h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)+\int_{0}^{x}ds \ h(s) exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)g(x)$$

$$\frac{d f(x)}{dx}= f_0 \ exp\Bigl( \int_{0}^{x}ds \ g(s) \Bigr) g(x) +\int_{0}^{x}ds \ h(s) exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)g(x)+ h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)$$

$$\frac{d f(x)}{dx}=f(x)g(x)+ h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)$$

Now I'm just having trouble with the term $h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)$, which is equivalent to $f(x,x)$. I was just having trouble figuring out what the exp term should be. After all, the easiest way would be for the integral to go from x to x, which would make the exponent zero and thus $e^{0}=1$ and I would get $f(x)g(x)+h(x)$

 

[pasmith]

Now I'm just having trouble with the term $h(x)exp\Bigl( \int_{s}^{x}dr \ g(r) \Bigr)$, which is equivalent to $f(x,x)$. I was just having trouble figuring out what the exp term should be. After all, the easiest way would be for the integral to go from x to x, which would make the exponent zero and thus $e^{0}=1$ and I would get $f(x)g(x)+h(x)$

Yes. The integrand is a function of $x$ and $s$, so it is evaluated at $(x,s) = (x,x)$.

 

[vela]

I then tried another approach and first wanted to get rid of the integrals in the exp terms

$$f_0 \exp\biggl( G(x)-G(0) \biggr)+\int_{0}^{x}ds \ h(s) \exp \biggl( G(x)-G(s) \biggr) \,dx$$
$$f_0 e^{G(x)-G(0)} + e^{G(x)} \int_{0}^{x}ds \ h(s) e^{-G(s)}$$

Unfortunately, I now have problems again with the derivative for the second term using this method.

The second term is of the form $e^{G(x)} [J(x)-J(0)]$ where $J'(x) = h(x) \exp[-G(x)]$. Just apply the product rule.

 

[Lambda96]

Thanks pasmith and vela for your help