Trigonometry lighthouse boat problem

[Nate1]

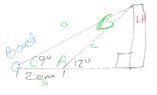
A boat is some distance away from a small lighthouse. The angle of elevation from the boat is 9 degrees. If the boat moves forward 20m, the angle is now 12. Assume the lighthouse is at a rightangle to the boat.

Calculate the distance from the boat to the top of the lighthouse before and after it moves
View attachment 8541

 

[Greg]

Hi Nate and welcome to MHB!

Any thoughts on how to begin?

 

[Nate1]

a/sinA = b/sinB = c/sinC

180 - 12 = 168

180 - (12+90) = 78
180 - (9+90) = 81
81 - 78 = 3 (top corner)

a/sin168 = 20/sin3
a = (20 x sin168)/sin3
a = 79.45

c/sin9 = 79.45/sin168
c = (79.45 x sin9)/sin168
c = 59.78

Correct?

View attachment 8542

 

[MarkFL]

I would let:

$$h$$ = the height of the lighthouse in meters

$$\theta_1$$ = the initial angle of inclination

$$\theta_2$$ = the final angle of inclination

$$x$$ = the final distance to the lighthouse in meters

Now, we may state:

$$\tan\left(\theta_1\right)=\frac{h}{x+20}$$

$$\tan\left(\theta_2\right)=\frac{h}{x}$$

Now, these equations imply:

$$h=x\tan\left(\theta_2\right)=(x+20)\tan\left(\theta_1\right)$$

Or:

$$x\tan\left(\theta_2\right)=(x+20)\tan\left(\theta_1\right)$$

Now, solve this for \(x\)...what do you get?

 

[Nate1]

x0.2126=x0.1584+3.1677
x0.0542=3.1677
x=58.47

 

[MarkFL]

x0.2126=x0.1584+3.1677
x0.0542=3.1677
x=58.47

I recommend solving the equation completely, then then plugging in the given values and rounding only at the very end.

$$x\tan\left(\theta_2\right)=(x+20)\tan\left(\theta_1\right)$$

$$x=\frac{20\tan\left(\theta_1\right)}{\tan\left(\theta_2\right)-\tan\left(\theta_1\right)}$$

Now plug in the given angles of inclination:

$$x=\frac{20\tan\left(9^{\circ}\right)}{\tan\left(12^{\circ}\right)-\tan\left(9^{\circ}\right)}\approx58.47452024794465$$

So, initially the boat was about 78.5 m from the lighthouse, and finally it was about 58.5 m from the lighthouse.

 

[Nate1]

So which methed is more accurate?

 

[MarkFL]

So which methed is more accurate?

When working problems involving numeric data, it is best to only round at the end, and not use rounded values in intermediary steps, as this can sometimes magnify rounding errors. Here it didn't make much difference, if any, but it is good practice to only round once, at the end. :)

 

[Nate1]

Sorry, by which method I meant the one you are using or the sin rule (seen above)

 

[Olinguito]

So, initially the boat was about 78.5 m from the lighthouse, and finally it was about 58.5 m from the lighthouse.

The problem is asking for the distances from the boat to the top of the lighthouse.

 

[MarkFL]

The problem is asking for the distances from the boat to the top of the lighthouse.

Oops...my bad. (Emo)

 

[MarkFL]

If we let \(d_1\) be the initial distance from the eye of the observer to the top of the lighthouse, and \(d_2\) be the final distance, and armed with the value of \(x\), we could state:

$$\cos\left(\theta_1\right)=\frac{x+20}{d_1}\implies d_1=(x+20)\sec\left(\theta_1\right)$$

$$\cos\left(\theta_2\right)=\frac{x}{d_2}\implies d_2=x\sec\left(\theta_2\right)$$

Now, it's just a matter of plugging in the data. :)