10 Math Things We All Learnt Wrong At School
Some of these could even lead to heavy debates within the scientific community, so maybe I should say: from my point of view. So before you get excited or even angry about what is to come, please keep in mind to take it with a big grain of salt and try to feel entertained, not schooled.
Table of Contents
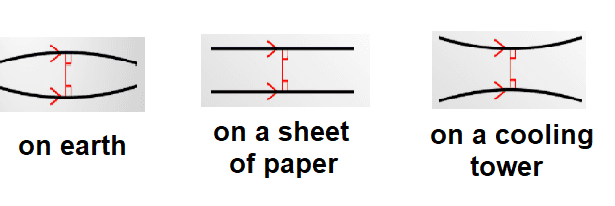
#10. The sum of all angles in a triangle is 180°
We all live on a globe, a giant ball. The angles of a triangle on this ball add up to a number greater than ##180°##.
And the amount by which the sum extends ##180°## isn’t even constant. It depends on the size of the triangle. The flat triangle with angle-sum ##180°## is the exception, not the norm. The real world is crooked.
#9. What is 360°?
The measuring of angles in degrees is at best confusing. Even the calculator on the computer allows three versions of a full angle: ##360°, 400°, 2\pi##. And whoever used the ##400°##? Anyway, ##2\pi## is what it should be: the ratio of the circumference of a circle of radius ##1## to its radius ##1##. It is how angles are used in mathematics: multiples of ##\pi##. Degrees should be treated like Roman numbers: a historical sidenote.
#8. Parallels do not intersect
Have you ever stood on a railroad track? Nobody would deny that the rails are in parallel. Do they intersect? Certainly not soon because locomotives normally do not derail. However, you will likely have looked to the horizon while standing on the rails. And – surprise – they do intersect at the horizon, or mathematically: at infinity. But infinity on a ball where we live isn’t at infinity. It is somewhere. We see that there are possible geometries, in which parallels do intersect.
#7. What is a tangent?
Yes, it is the derivative of ##y.## But what is meant by that? Obviously we have a function ##x \longmapsto y=y(x)## and a derivative $$y’=y'(x)=\dfrac{dy}{dx}=\left. \dfrac{d}{dx}\right|_{x=a}y(x)=y(a+h)-J(h)-r(h)=y'(a) $$ It now isn’t obvious at all what is meant: the function ##x\longmapsto y'(x)##, the value of the slope ##y'(a)##, or the linear map ##J,## the Jacobi matrix, the tangent in a way? The fact is, all of them, as needed according to the situation. I don’t say we should teach tangent bundles and sections, but a little bit more accuracy would smoothen the step to calculus at college.
#6. ##0.999999999… =0.\bar{9}= 1##
This isn’t a problem at school. It is a problem in understanding. Yes, the two numbers are indeed equal. They are only written in a different representation, such as ##\pi = 3.1415926… ## notes pi and everyone knows it is not ending with ##6## or ever. Nevertheless, we write ‘##=##’ and not ‘##\approx##’ because we mean the actual number, not its approximation.
\begin{align*}0.\bar{9}=0.999999999…&=\dfrac{9}{10}+\dfrac{9}{100}+\dfrac{9}{1000}+\ldots\\[10pt] &=\sum_{i=0}^\infty \dfrac{9}{10^{i+1}} =9\cdot\sum_{i=0}^\infty \dfrac{1}{10}\cdot\dfrac{1}{10^{i}}\stackrel{(*)}{=}\dfrac{9}{10}\cdot\lim_{n \to \infty}\sum_{i=0}^n \dfrac{1}{10^{i}}\\[10pt] &=\dfrac{9}{10}\cdot \lim_{n \to \infty}\dfrac{1-\left(\dfrac{1}{10}\right)^{i+1}}{1-\dfrac{1}{10}} \stackrel{(**)}{=} \dfrac{9}{10}\cdot \dfrac{1-0}{1-\dfrac{1}{10}}=\dfrac{9}{10}\cdot \dfrac{10}{9}=1\end{align*}
The crucial points are the limits. While the first one ##(*)## is simply a translation for ‘and so on’, i.e. the dots in ‘##0.999999999…##’ which shouldn’t be controversial, the misconception begins at the second one ##(**)##. A limit isn’t a process, it is a number! And ##0.\bar{9}## isn’t a process either, it is a number. Number ##1.##
#5. The rationals are numbers
They are not. They are equivalence classes. My favorite example is, that it makes a huge difference whether you carry home a pie from the bakery or ##12/12## pieces of a pie. The amount of pie and the prizes would be the same, but their appearance is not. Of course, we treat ##1=\frac{12}{12}## the same because we are interested in its value, however, they are only equal because ##1\cdot 12 = 12 \cdot 1.## It becomes clearer in its general form:
$$\dfrac{a}{b}\sim\dfrac{c}{d}\Longleftrightarrow a\cdot d= b\cdot c$$
‘##\sim##’ is strictly speaking an equivalence relation. It gathers many quotients under one name
$$1=\left\{1,\dfrac{2}{2},\dfrac{-3}{-3},\dfrac{12}{12},\ldots\right\}$$
and the same is true for all other quotients. We take them as the same and write ‘##=##’ instead of ‘##\sim##’ because we are only interested in their values. But ##1\neq \dfrac{12}{12}.## You can literally see that it is different: ##5## symbols instead of ##1.##
#4. Reducing an equation
This time I’m hopefully by teachers. The standard procedure is
$$ab=ac \;\Longrightarrow \;b=c$$
It is not only sloppy, it is even wrong sometimes, and belongs to the standard mistakes in class. If we all would take the time and write it down more carefully, this could avoid the mistake: \begin{align*}ab=ac \;\Longrightarrow \;ab-ac=0\;\Longrightarrow \;a\cdot (b-c)=0\;\Longrightarrow \;a=0\quad\text{ or }\quad b=c\end{align*}
The second possibility is all of a sudden evident. The advice behind it is simple: avoid divisions as long as you can. And if so, make sure you are allowed to. Which brings us to …
#3. You cannot divide by ##0##
This always sounded to me as if there was obscure mathematics police that forbids us to do so. Why? Well, there is a simple reason: ##0## hasn’t the least to do with multiplication and even less with division. The question to divide by ##0## doesn’t even arise! The neutral element for multiplication is ##1,## not ##0##. That belongs to addition. And there is only one way to combine the two, namely by the distributive law ##a\cdot(b+c)=a\cdot b+b\cdot c.## Let’s pretend there was a meaningful way to define ##m=a:0.## Then $$a=m\cdot 0 = m\cdot (1-1)= m\cdot 1 – m\cdot 1= m-m =0$$ So can we at least divide ##a=0## by ##0## and get ##m##? I’m afraid not. Have a look at $$m\cdot a= \dfrac{0}{0}\cdot a = \dfrac{0\cdot a}{0\cdot 1}=\dfrac{0}{0}=m\Longrightarrow a=1$$ or $$2m=(1+1)\cdot m=m+m=\dfrac{0}{0}+\dfrac{0}{0}=\dfrac{0+0}{0}=\dfrac{0}{0}=m$$ and we could only multiply ##m## by ##1##. So even if we define ##m=0/0## we wouldn’t get anything useful in the sense that it would be connected to any number we normally use for calculations.
#2. 1+1=2
This is funny because you currently read this text on a device that uses ##1+1=0.## If we switch off a light we use it because switching twice doesn’t change anything, i.e. equals zero. Our clocks measure hours from ##1## to ##12## and start again after that. So obviously ##12=0## and ##3\cdot 4 = 2\cdot 6 = 0.## See, it depends on the context. There are all kinds of number systems: binary for the computer, duodecimal for clocks, the Babylonians used sexagesimal, and the Mayas used cycles of ##1,872,000## days. You won’t convince your local grocery store that twice as much is zero, but you use it while you read those lines.
12= 0? 24=0? 60=0?
#1. A prime is only divisible by ##1## and itself
This is wrong. Well, yes and no. Strictly speaking, this definition describes irreducibility. And irreducibility is different from primality. A prime number is a number, that if it divides a product, then it has to divide one of the factors. $$7\,|\,28=2\cdot 14 \;\Longrightarrow \;7\,|\,2\text{ or }7\,|\,14$$ $$4\,|\,12=2\cdot 6\text{ but }4\,\nmid \,2 \text{ and } 4\,\nmid \,6$$ It is a bit more complicated, but it is the correct definition. However, irreducible integers are prime integers and vice versa which is why the correct definition is replaced at school by the more handy one. However, there are domains in which this is not the case. The standard example is ##\mathbb{Z}[\sqrt{-5}]## where $$6=2\cdot 3=(1+\sqrt{-5})\cdot (1-\sqrt{-5})$$ is a decomposition into irreducible factors that are not prime.
”
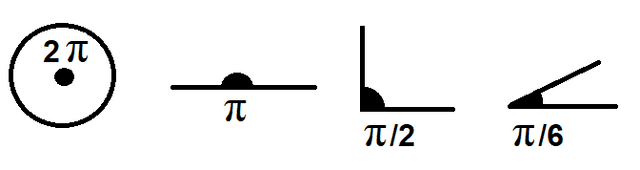
And note that because we live on the (non-Euclidean) curved surface of the Earth ‘curving something around a ball and calling it straight’ is what we do every day when we travel in a straight line.
”
For those interested in this sort of thing, read the first few chapters of the Feynman Lectures available free from Caltech.
Thanks
Bill
”
An interesting read, though I do take exception to those concepts of Parallel and Triangles in #8 and #10.
#8 is false because clearly the train tracks don’t meet, and #10 is false because a triangle is defined as having 3 straight sides and 3 angles, and those sides aren’t straight. You can’t curve something around a ball and call it straight! What you’re doing there is taking your reference plane as being perpendicular to gravity for drawing the triangle and then taking the reference as floating to measure the angles. With consistent definition, a triangles internal angles will add up to 180°.
At infinity a parallel pair of rails may appear to be meeting, but then with an infinitely powerful zoom one could see that they aren’t!
”
See:
[URL]https://en.wikipedia.org/wiki/Non-Euclidean_geometry[/URL]
”
Now this one is indeed devilish, because the key assumptions that make it so are “off the chalk board” and only appropriate to apply only under some highly specific circumstances that the vast majority of people who use math never encounter.
”
Exactly. As I mentioned we unconsciously make assumptions in math, physics, all sorts of areas all the time. They are so ingrained we do not even realise it. Yet situations crop up occasionally where we are incorrect in making those assumptions. When talking about math, it is quite easy for ‘lay’ people to get confused and say it must be wrong etc. Do an internet search on 1+2+3+4…… and you will see what I mean. This one was particularly active a few years ago and you see some laughable comments on it. The correct answer was given by Mathlodger, although I would not call it debunked:
[MEDIA=youtube]YuIIjLr6vUA[/MEDIA]
Not understanding this can lead to all sorts of sloppy thinking.
Thanks
Bill
All true. But even the concept of point (except when using Hilbert’s Axioms) is problematical. It is supposed to have a position but no size. Such, of course, do not exist. We all carry around assumptions we are not aware of. Generally, they cause no problems. But occasionally, they rear their ugly head and need to be challenged. An example is the good old 1+2+3+4…… = Infinity. But as we all know from studying complex analysis by the ‘trick’ of regularisation, it is -1/12. We normally take for granted that when talking about natural numbers, they are not considered a subset of the complex plane. However, in some calculations, such as the Casimir force, if we wish to avoid infinity, that is exactly what we must do. It is related to the epistemological position of Model Dependent Realism:
https://en.wikipedia.org/wiki/Model-dependent_realism
Thanks
Bill
”
“The sum of all angles in a triangle is 180 degrees”, is wrong … Needed, say “INTERNAL ANGLES”,
”
In its defense it does say “in a triangle”, not “outside a triangle”. :oldbiggrin:
”
I am a little confused but not worried as maybe I should.
”
I am a little confused too. I assumed your post #4 about angles of a triangle was trying to point out something wrong with the diagram in post #1, but I don’t see anything wrong. I may not be giving you enough credit.
”
The angles of the triangle being 180 degrees SPECIFICALLY tells of those as INTERIOR angles.
”
Yes. The marked interior angles of that triangle add up to about 300 degrees.
”
Although, I would summarize this by saying that there aren’t parallel lines in projective geometry, not that parallels intersect.
”
Yes, I saw this weakness, too. But I decided to live with it since a detour to projective geometry would have been off-topic and too long.
”
Sure, but why does projective geometry have to enter this?
”
It doesn’t. Of course the “myth” is not a myth, in Euclidean geometry of the plane the sum of the angles in a triangle do add up to two right angles. Nothing wrong about this.
I have one nitpick about the discussion of rationals. In the foundations of mathematics, what’s done is this:
[LIST=1]
[*]We start with the natural numbers, 0, 1, 2 … (some people start with 1 instead of 0).
[*]Then we can define the integers as equivalence classes of pairs of naturals: ##(a,b) \approx (c,d)## if ##a+d = b + c##. So ##8 – 12## is defined to be the equivalence class ##(8, 12)##.
[*]Then we can define the rationals as equivalence classes of pairs ##(n,m)## where ##(n,m) \approx (p,q)## if ##n \times q = m \times p##.
[*]Then we can define the reals as equivalence classes of convergent sequences of rationals.
[/LIST]
However, having developed the reals, we can then, for most purposes, just consider a real to be a “black box” with certain closure properties, and then consider rationals and integers and naturals as particular subsets of the reals. Then under this reinterpretation, an expression such as ##2/3## means the real number resulting from dividing the real number 2 by the real number 3. So ##2/3 = 4/6## is an equality.