A Principle Explanation of the “Mysteries” of Modern Physics
All undergraduate physics majors are shown how the counterintuitive aspects (“mysteries”) of time dilation and length contraction in special relativity (SR) follow from the light postulate, i.e., that everyone measures the same value for the speed of light c, regardless of their motion relative to the source (see this Insight, for example). And, we can understand the light postulate to follow from the principle of relativity, sometimes referred to as “no preferred reference frame” (NPRF). Simply put, if the speed of light from a source was only equal to ##c=\frac{1}{\sqrt{\epsilon_o \mu_o}}## (per Maxwell’s equations) for one particular velocity relative to the source, that would certainly constitute a preferred reference frame. Borrowing from Einstein [1], NPRF might be stated (see this Insight):
No one’s “sense experiences,” to include measurement outcomes, can provide a privileged perspective on the “real external world.”
While time dilation and length contraction follow “analytically” from the light postulate, there are those who do not consider the light postulate explanatory, since it does not provide “hypothetically constructed” mechanisms to “synthetically” account for time dilation and length contraction [2][3]. That is, the postulates of SR are “empirically discovered” principles offered without corresponding “constructive efforts.” In what follows, Einstein explains the difference between the two [4]:
We can distinguish various kinds of theories in physics. Most of them are constructive. They attempt to build up a picture of the more complex phenomena out of the materials of a relatively simple formal scheme from which they start out. Thus the kinetic theory of gases seeks to reduce mechanical, thermal, and diffusional processes to movements of molecules – i.e., to build them up out of the hypothesis of molecular motion. When we say that we have succeeded in understanding a group of natural processes, we invariably mean that a constructive theory has been found which covers the processes in question.
Along with this most important class of theories there exists a second, which I will call “principle-theories.” These employ the analytic, not the synthetic, method. The elements which form their basis and starting point are not hypothetically constructed but empirically discovered ones, general characteristics of natural processes, principles that give rise to mathematically formulated criteria which the separate processes or the theoretical representations of them have to satisfy. Thus the science of thermodynamics seeks by analytical means to deduce necessary conditions, which separate events have to satisfy, from the universally experienced fact that perpetual motion is impossible.
The advantages of the constructive theory are completeness, adaptability, and clearness, those of the principle theory are logical perfection and security of the foundations. The theory of relativity belongs to the latter class. In order to grasp its nature, one needs first of all to become acquainted with the principles on which it is based.
Here is why Einstein formulated special relativity as a principle theory [5, pp. 51-52]:
By and by I despaired of the possibility of discovering the true laws by means of constructive efforts based on known facts. The longer and the more despairingly I tried, the more I came to the conviction that only the discovery of a universal formal principle could lead us to assured results.
Despite the fact that “there is no mention in relativity of exactly how clocks slow, or why meter sticks shrink” (no “constructive efforts”), the “empirically discovered” principles of SR are so compelling that “physicists always seem so sure about the particular theory of Special Relativity, when so many others have been superseded in the meantime” [6].
As it turns out, we are in a similar position today with quantum mechanics (QM). For example, QM accurately predicts violations of the Clauser-Horne-Shimony-Holt (CHSH) inequality all the way to the Tsirelson bound for Bell state entanglement without providing a corresponding constructive account (see this Insight, for example). This prompted Lee Smolin to write [7, p. 227]:
So, my conclusion is that we need to back off from our models, postpone conjectures about constituents, and begin again by talking about principles.
Other physicists are also calling for a principal account of QM. Chris Fuchs writes [8, p. 285]:
Compare [quantum mechanics] to one of our other great physical theories, special relativity. One could make the statement of it in terms of some very crisp and clear physical principles: The speed of light is constant in all inertial frames, and the laws of physics are the same in all inertial frames. And it struck me that if we couldn’t take the structure of quantum theory and change it from this very overt mathematical speak — something that didn’t look to have much physical content at all, in a way that anyone could identify with some kind of physical principle — if we couldn’t turn that into something like this, then the debate would go on forever and ever. And it seemed like a worthwhile exercise to try to reduce the mathematical structure of quantum mechanics to some crisp physical statements.
That QM accurately predicts the violation of the CHSH inequality to the Tsirelson bound without spelling out any corresponding constructive account prompted Smolin to write [7, p. xvii]:
I hope to convince you that the conceptual problems and raging disagreements that have bedeviled quantum mechanics since its inception are unsolved and unsolvable, for the simple reason that the theory is wrong. It is highly successful, but incomplete.
Of course, this is precisely the complaint leveled by Einstein, Podolsky, and Rosen (EPR) in their famous paper, “Can Quantum-Mechanical Description of Physical Reality Be Considered Complete?” [9]. In an equally famous paper (published after Einstein’s death), John Bell showed how any naïve “completion” of QM via hidden variables could conflict with the predictions of QM for two entangled particles (e.g., see this Insight) [10]. As long as the particles couldn’t communicate faster than light and didn’t have access to information about measurement settings until they were actually being measured, the outcomes for entangled particles with hidden variables would have to obey the “Bell inequality” (the CHSH inequality is a variation thereof). There are two-particle entangled states (“Bell states“) in QM that can violate the Bell inequality (and therefore, the CHSH inequality) and these violations are now experimentally well-confirmed. This “mystery” is in large part responsible for “the conceptual problems and raging disagreements that have bedeviled quantum mechanics.”
However, contra Smolin, we recently showed that Bell state entanglement does not render QM “wrong” or “incomplete” by extending NPRF to include the measurement of another fundamental constant of nature, Planck’s constant h [11][12][30]. As Steven Weinberg points out, measuring an electron’s spin via Stern-Gerlach (SG) magnets constitutes the measurement of “a universal constant of nature, Planck’s constant” [13, p. 3] (Figure 1). So if NPRF applies equally here, everyone must measure the same value for Planck’s constant h, regardless of their SG magnet orientations relative to the source, which like the light postulate is an “empirically discovered” fact. By “relative to the source” of a pair of spin-entangled particles, I mean relative “to the vertical in the [symmetry] plane perpendicular to the line of flight of the particles” [14, p. 943] (Figure 2). Thus, different SG magnet orientations relative to the source constitute different “reference frames” in QM just as different velocities relative to the source constitute different “reference frames” in SR.
Just as NPRF and the speed of light c produce the “mysteries” of time dilation and length contraction in SR, we showed that NPRF and Planck’s constant h produce the “mysteries” of Bell state entanglement and the Tsirelson bound for QM. Generally speaking, Bell spin state entanglement results from the conservation of spin angular momentum, and conservation principles and gauge invariance follow from Wheeler’s “boundary of a boundary” principle, ##\partial\partial = 0## [15][16][17] (Figure 3), which with NPRF underwrite all of physics [17][18].
Specifically, when Alice and Bob make their SG spin measurements at the same angle in the plane of symmetry (same reference frame), conservation of spin angular momentum dictates that they obtain the same result (both +1 or both -1) for the spin triplet states (opposite results for the spin singlet state). This suggests (naively) that if Bob makes his SG spin measurement at angle ##\theta## with respect to Alice (different reference frames), then he should obtain ##\cos{(\theta)}## when Alice obtains +1 in accord with the conservation of spin angular momentum (Figure 4). But, Bob can only ever measure ##\pm 1## per NPRF, just like Alice, so the conservation principle is constrained to hold only on average per NPRF (Figures 5 & 6). Thus, Bell state entanglement and the Tsirelson bound are “mysteries” precisely because of “average-only” conservation, which is conservation per NPRF (Figure 7). So we see that the principle of NPRF reveals an underlying coherence between non-relativistic QM and SR (Figure 8) where others have perceived tension.
For example, in his paper “On the Incompatibility of Special Relativity and Quantum Mechanics” Marco Mamone-Capria’s argument is based on “The EPR correlations … as a simple example of quantum mechanical macroscopic effects with spacelike separation from their causes” [19]. He points out that in 1972 Dirac wrote [20, p. 11]:
The only theory which we can formulate at the present is a non-local one, and of course one is not satisfied with such a theory. I think one ought to say that the problem of reconciling quantum theory and relativity is not solved.
And, Bell also voiced concerns about the compatibility of SR and QM based on quantum entanglement [21, p. 172]:
For me then this is the real problem with quantum theory: the apparently essential conflict between any sharp formulation and fundamental relativity. That is to say, we have an apparent incompatibility, at the deepest level, between the two fundamental pillars of contemporary theory.
Of course, we know QM is not Lorentz invariant and so it deviates trivially from SR in that fashion. In order to get QM from Lorentz invariant quantum field theory one needs to make low energy approximations [22, p. 173]. But, the charge of incompatibility based on QM entanglement actually carries serious consequences, because we have experimental evidence confirming the violation of the CHSH inequality per QM entanglement. So, if the violation of the CHSH inequality is in any way inconsistent with SR, then SR is being challenged empirically. By analogy, we know Newtonian mechanics deviates from SR because it is not Lorentz invariant. As a consequence, Newtonian mechanics predicts a very different velocity addition rule, so suppose we found experimentally that velocities do add as predicted by Newtonian mechanics. That would not merely mean that Newtonian mechanics and SR are incompatible, that would mean Newtonian mechanics has been empirically verified while SR has been empirically refuted. So, if one believes the violation of the CHSH inequality is in any way inconsistent with SR, and one believes the experimental evidence is accurate, then one believes SR has been empirically refuted. Clearly that is not the case, so their reconciliation as regards the violation of the CHSH inequality must certainly obtain in some fashion and here we see how the principle of NPRF does the job.
Further, contrary to Smolin and EPR, Bell state entanglement does not mean that QM is “incomplete” or “wrong.” Rather, QM is as complete as possible per the principles of ##\partial\partial = 0## and NPRF.
Given this result, one immediately wonders if general relativity (GR) can be brought into the mix via NPRF and the gravitational constant G. Of course it can and the associated counterintuitve aspect (“mystery”) in GR is the contextuality of mass. We already showed how this might resolve the missing mass problem without having to invoke non-baryonic dark matter [23][24].
Specifically, I am pointing out the well-known result per GR that matter can simultaneously possess different values of mass when it is responsible for different combined spatiotemporal geometries. Here “reference frame” refers to each of the different spatiotemporal geometries associated with one and the same matter source. Tacitly assumed in this result is of course that G has the same value in each reference frame, which is consistent with NPRF as applied to c and h above. This spatiotemporal contextuality of mass is not present in Newtonian gravity where mass is an intrinsic property of matter. For example, when a Schwarzschild vacuum surrounds a spherical matter distribution the “proper mass” ##M_{p}## of the matter, as measured locally in the matter, can be different than the “dynamic mass” ##M## in the Schwarzschild metric responsible for orbital kinematics about the matter [25, p. 126]. This difference is attributed to binding energy and goes as ##dM_p = \left(1-\frac{2GM(r)}{c^2r}\right)^{-1/2} \: dM##. In another example, suppose a Schwarzschild vacuum surrounds a sphere of Friedmann-Lemaitre-Robertson-Walker (FLRW) dust, as used originally to model stellar collapse [15, pp. 851-853]. The dynamic mass ##M## of the surrounding Schwarzschild metric is related to the proper mass ##M_{p}## of the FLRW dust, as joined at FLRW radial coordinate ##\chi_o##, by
\begin{equation}
\frac{M_p}{M} = \frac{3(2\chi_o -\sin(2\chi_o))}{4 \sin ^3(\chi_o)} \label{massratio}
\end{equation}
where
\begin{equation}
ds^2 = -c^2d\tau^2 + a^2(\tau)\left(d\chi^2 + \sin^2\chi d\Omega^2 \right) \label{FLRWmetric}
\end{equation}
is the closed FLRW metric [26]. I should quickly point out that this may prima facie seem to constitute a violation of the equivalence principle, as understood to mean inertial mass equals gravitational mass, since inertial mass can’t be equal to two different values of gravitational mass. But, the equivalence principle says simply that spacetime is locally flat [27, pp. 68-69] and that is certainly not being violated here nor with any solution to Einstein’s equations.
Thus, contrary to what some believe about SR, QM, and GR collectively, these theories are comprehensive (not “incomplete” as claimed in [7] and [9]) and coherent (not “in conflict” as claimed in [19] and [20]). In order to appreciate the beauty of these theories collectively, one need only view them per the principles of ##\partial\partial = 0## and NPRF with their associated “mysteries” corresponding to c, h, and G, respectively. I close with this quote from Wolfgang Pauli [28, p. 33]:
‘Understanding’ nature surely means taking a close look at its connections, being certain of its inner workings. Such knowledge cannot be gained by understanding an isolated phenomenon or a single group of phenomena, even if one discovers some order in them. It comes from the recognition that a wealth of experiential facts are interconnected and can therefore be reduced to a common principle. In that case, certainty rests precisely on this wealth of facts. The danger of making mistakes is the smaller, the richer and more complex the phenomena are, and the simpler is the common principle to which they can all be brought back. … ‘Understanding’ probably means nothing more than having whatever ideas and concepts are needed to recognize that a great many different phenomena are part of a coherent whole.
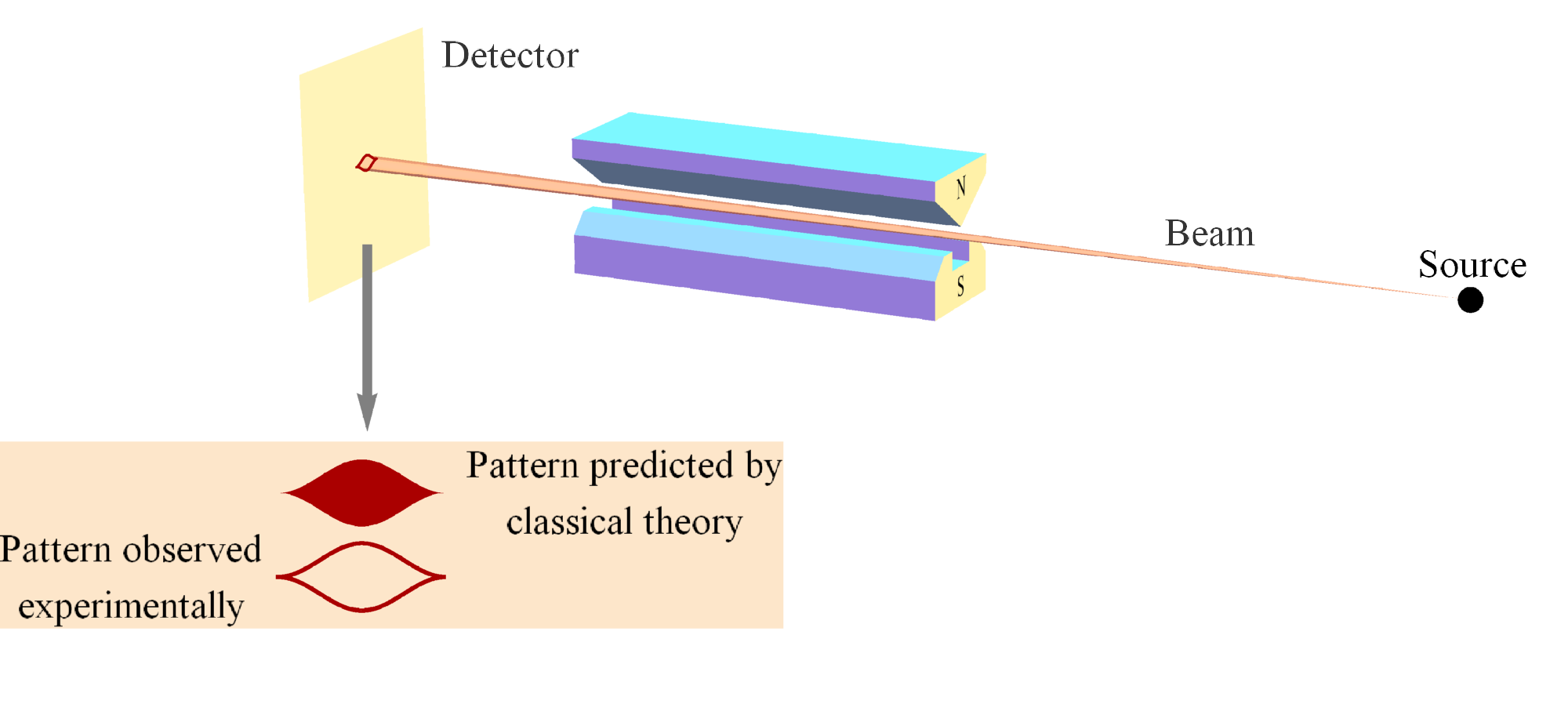
Figure 1. A Stern-Gerlach (SG) spin measurement showing the two possible outcomes, up (##+\frac{\hbar}{2}##) and down (##-\frac{\hbar}{2}##) or +1 and -1, for short. The important point to note here is that the classical analysis predicts all possible deflections, not just the two that are observed. The difference between the classical prediction and the quantum reality uniquely distinguishes the quantum joint distribution from the classical joint distribution for the Bell spin states [29].
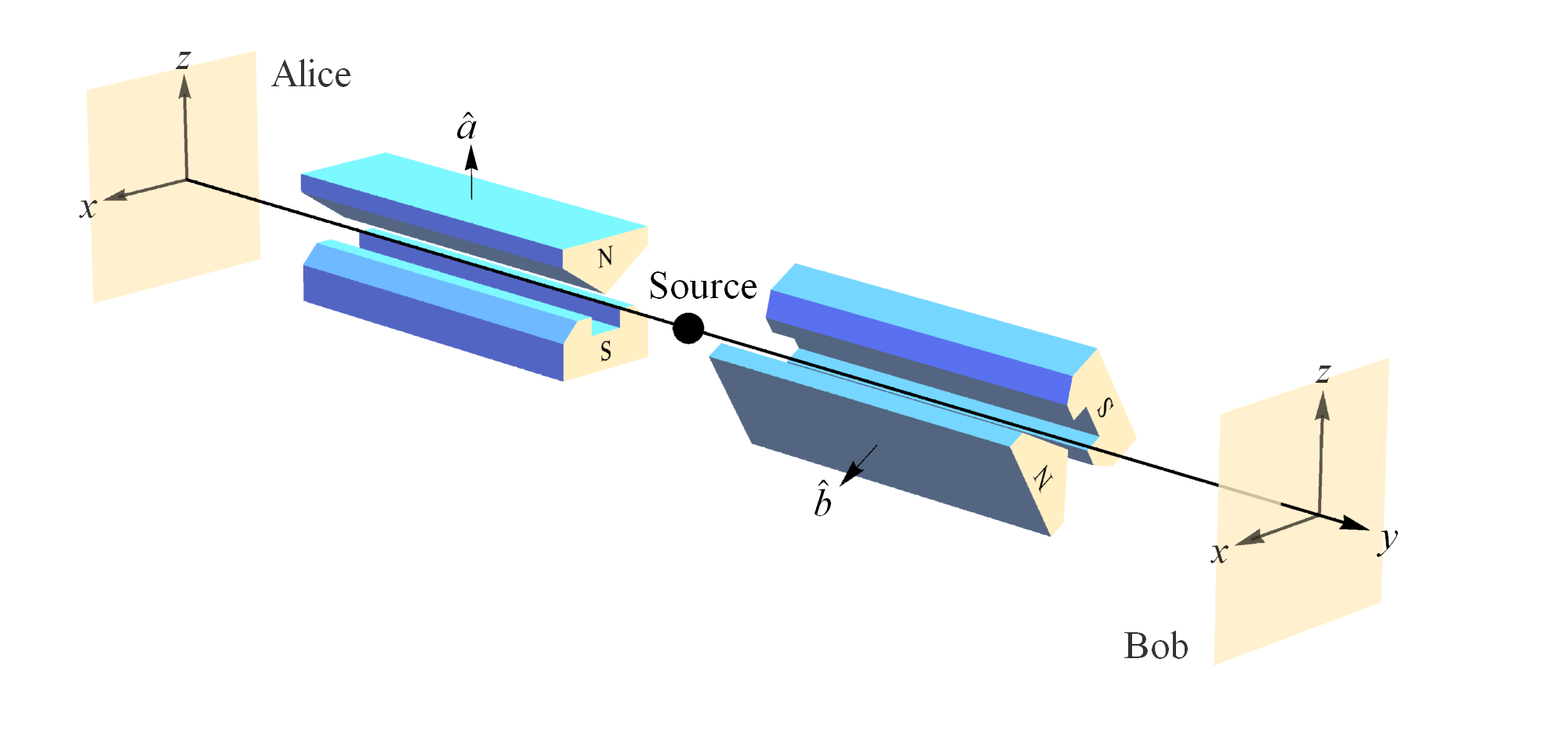
Figure 2. Alice and Bob making spin measurements on a pair of spin-entangled particles with their Stern-Gerlach (SG) magnets and detectors in the xz-plane. Here Alice and Bob’s SG magnets are not aligned so these measurements represent different reference frames.
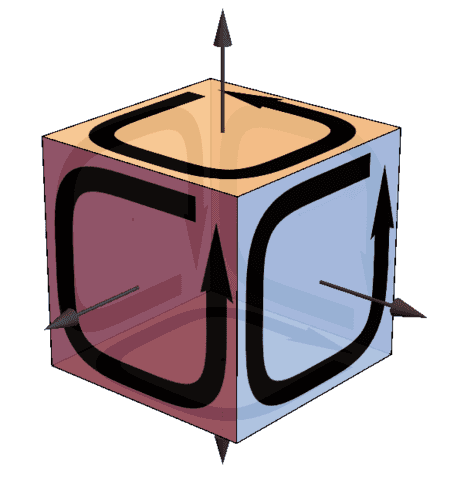
Figure 3. The boundary of a boundary is zero. The oriented plaquettes bound the cube and the directed edges bound the plaquettes. As you can see from the picture, every edge has oppositely oriented directions that cancel out. Thus, the boundaries of the plaguettes (the edges), which bound the cube, sum to zero.
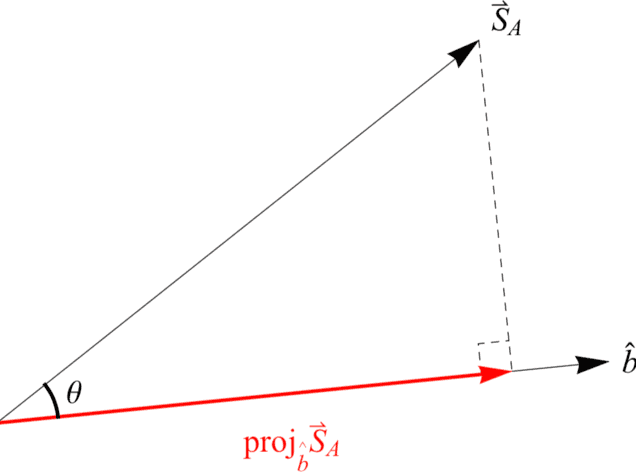
Figure 4. The angular momentum of Bob’s particle ##\vec{S}_B = \vec{S}_A## projected along his measurement direction ##\hat{b}##. This does not happen with spin angular momentum because Bob must always measure ##\pm 1##, no fractions, in accord with NPRF.
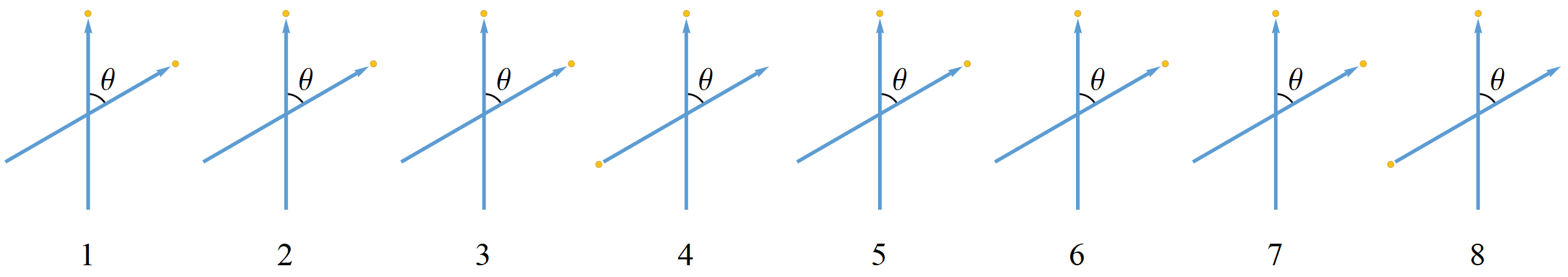
Figure 5. A spatiotemporal ensemble of 8 experimental trials for the spin triplet states showing Bob’s outcomes corresponding to Alice’s +1 outcome when ##\theta = 60^\circ##. Blue arrows depict SG magnet orientations and yellow dots depict the measurement outcomes. Spin angular momentum is not conserved in any given trial, because there are two different measurements being made, i.e., outcomes are in two different reference frames, but it is conserved on average for all 8 trials (six up outcomes and two down outcomes average to ##\cos{(60^\circ)}=\frac{1}{2}##).
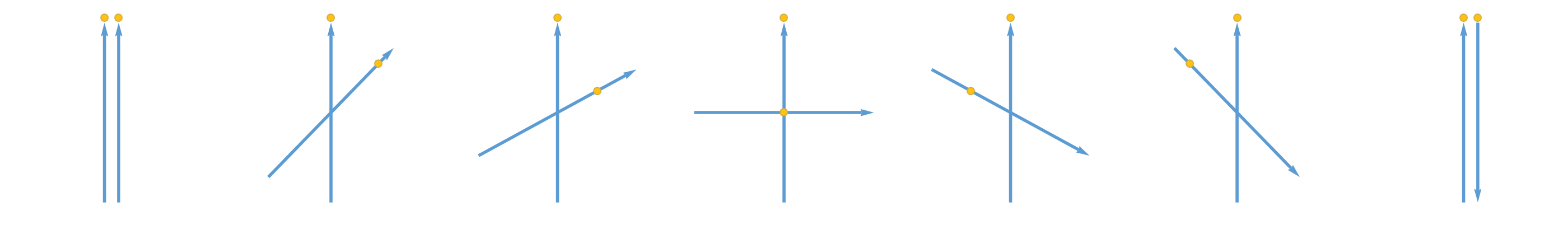
Figure 6. Average View for the Spin Triplet States. Reading from left to right, as Bob rotates his SG magnets relative to Alice’s SG magnets for her +1 outcome, the average value of his outcome varies from +1 (totally up, arrow tip) to 0 to -1 (totally down, arrow bottom). This obtains per conservation of spin angular momentum on average in accord with no preferred reference frame. Bob can say exactly the same about Alice’s outcomes as she rotates her SG magnets relative to his SG magnets for his +1 outcome. That is, their outcomes can only satisfy conservation of spin angular momentum on average in different reference frames, because they only measure ##\pm 1##, never a fractional result. Again, just as with the light postulate of special relativity, we see that no preferred reference frame leads to a counterintuitive result. Here it requires quantum outcomes ##\pm 1 \left(\frac{\hbar}{2}\right)## for all measurements leading to the “mystery” of “average-only” conservation.
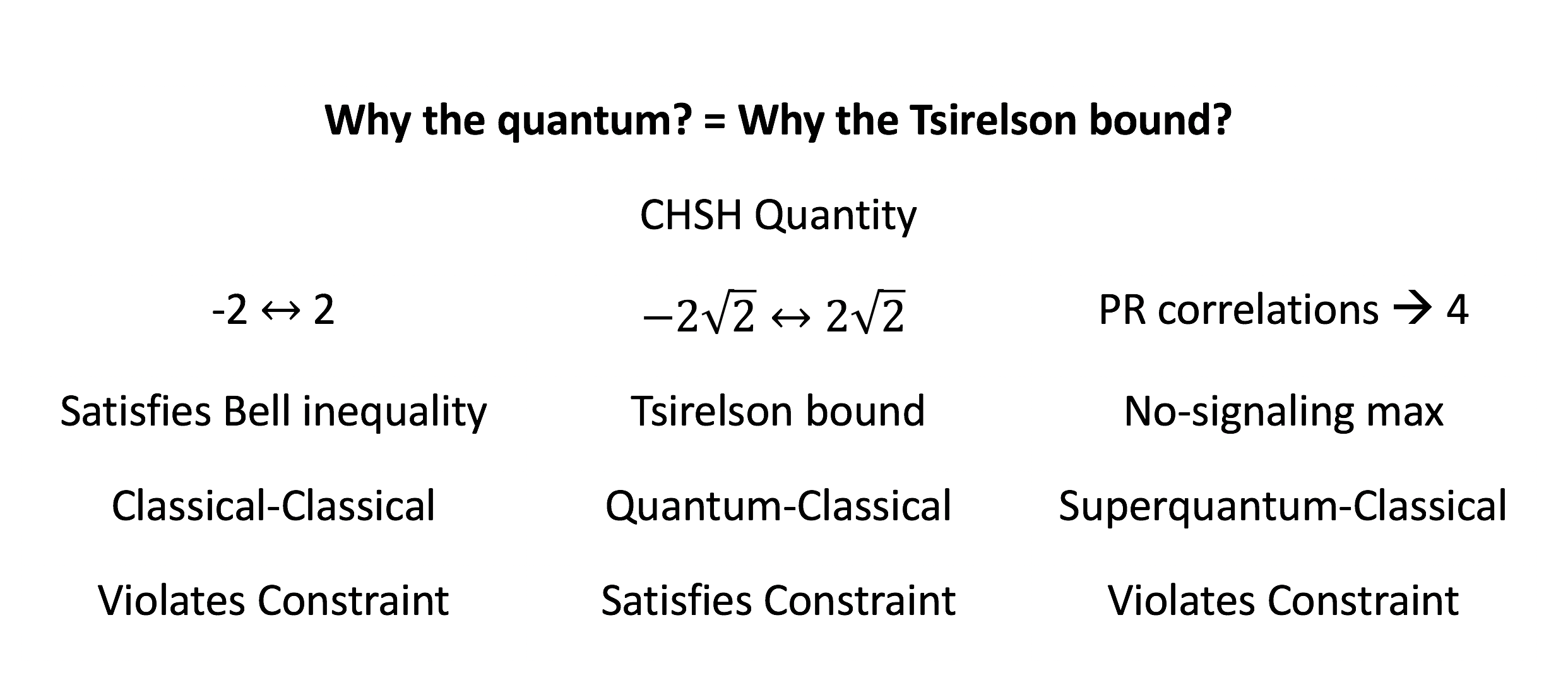
Figure 7. Bub’s version of Wheeler’s question “Why the quantum?” is “Why the Tsirelson bound?” The “constraint” is the principle of “conservation per no preferred reference frame”.
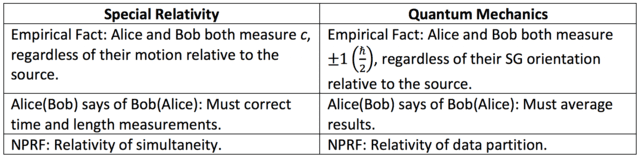
Figure 8. Comparing SR with QM according to no preferred reference frame (NPRF). Because Alice and Bob both measure the same speed of light c, regardless of their motion relative to the source per NPRF, Alice(Bob) may claim that Bob’s(Alice’s) length and time measurements are erroneous and need to be corrected (length contraction and time dilation). Likewise, because Alice and Bob both measure the same values for spin angular momentum ##\pm 1## ##\left(\frac{\hbar}{2}\right)##, regardless of their SG magnet orientation relative to the source per NPRF, Alice(Bob) may claim that Bob’s(Alice’s) individual ##\pm 1## values are erroneous and need to be corrected (averaged, Figures 5 & 6). In both cases, NPRF resolves the “mystery” it creates. In SR, the apparently inconsistent results can be reconciled via the relativity of simultaneity. That is, Alice and Bob each partition spacetime per their own equivalence relations (per their own reference frames), so that equivalence classes are their own surfaces of simultaneity and these partitions are equally valid per NPRF. This is completely analogous to QM, where the apparently inconsistent results per the Bell spin states arising because of NPRF can be reconciled by NPRF via the “relativity of data partition.” That is, Alice and Bob each partition the data per their own equivalence relations (per their own reference frames), so that equivalence classes are their own +1 and -1 data events and these partitions are equally valid.
References
- A. Einstein, Journal of the Franklin Institute 221(3), 349 (1936).
- H. Brown, Physical Relativity: Spacetime Structure from a Dynamical Perspective (OxfordUniversity Press, Oxford, UK, 2005).
- H. Brown, O. Pooley, in The Ontology of Spacetime, ed. by D. Dieks (Elsevier, Amsterdam, 2006), p. 67.
- A. Einstein, London Times pp. 53–54 (1919).
- A. Einstein, in Albert Einstein: Philosopher-Scientist, ed. by P.A. Schilpp (Open Court, La Salle,IL, USA, 1949), pp. 3–94.
- P. Mainwood. What Do Most People Misunderstand About Einstein’s Theory Of Relativity? (2018).
- L. Smolin, Einstein’s Unfinished Revolution: The Search for What Lies Beyond the Quantum (Penguin Press, New York, 2019).
- C. Fuchs, B. Stacey, in Quantum Theory: Informational Foundations and Foils, ed. by G. Chiribella, R. Spekkens (Springer, Dordrecht, 2016), pp. 283–305.
- A. Einstein, B. Podolsky, N. Rosen, Physical Review 47, 777 (1935).
- J. Bell, Physics 1, 195–200 (1964).
- W. Stuckey, M. Silberstein, T. McDevitt, I. Kohler, Entropy 21, 692 (2019). https://arxiv.org/abs/1807.09115
- W. Stuckey, M. Silberstein, T. McDevitt, T. Le, Scientific Reports 10, 15771 (2020). www.nature.com/articles/s41598-020-72817-7
- S. Weinberg. The Trouble with Quantum Mechanics (2017).
- N. Mermin, American Journal of Physics 49(10), 940 (1981).
- C. Misner, K. Thorne, J. Wheeler, Gravitation (W.H. Freeman, San Francisco, 1973).
- D. Wise, Classical and Quantum Gravity 23(17), 5129 (2006).
- A. Kheyfets, J. Wheeler, International Journal of Theoretical Physics 25, 573–580 (1986).
- M. Silberstein, W. Stuckey, Entropy 22, 551 (2020). https://www.mdpi.com/1099-4300/22/5/551/pdf
- M. Mamone-Capria, Journal for Foundations and Applications of Physics 8(2), 163 (2018). https://arxiv.org/pdf/1704.02587.pdf
- P. Dirac, in The Physicist’s Conception of Nature, ed. by J. Mehra (Reidel, Boston, 1973).
- J. Bell, Speakable and Unspeakable in Quantum Mechanics (Cambridge University Press, Cambridge, Massachusetts, 1987).
- A. Zee, Quantum Field Theory in a Nutshell (Princeton University Press, Princeton, 2003).
- W. Stuckey, T. McDevitt, A. Sten, M. Silberstein, International Journal of Modern Physics D 25(12), 1644004 (2016). http://arxiv.org/abs/1605.09229
- W. Stuckey, T. McDevitt, A. Sten, M. Silberstein, International Journal of Modern Physics D 27(14), 1847018 (2018). https://arxiv.org/abs/1509.09288
- R. Wald, General Relativity (University of Chicago Press, Chicago, 1984).
- W. Stuckey, American Journal of Physics 62(9), 788 (1994).
- S. Weinberg, Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity (John Wiley & Sons, New York, 1972).
- W. Heisenberg, Physics and Beyond: Encounters and Conversations (Harper & Row, New York, 1971).
- A. Garg, N. Mermin, Physical Review Letters 49, 901 (1982).
- M. Silberstein, W. Stuckey, T. McDevitt, Entropy 23(1), 114 (2021). https://www.mdpi.com/1099-4300/23/1/114/htm
PhD in general relativity (1987), researching foundations of physics since 1994. Coauthor of “Beyond the Dynamical Universe” (Oxford UP, 2018).









I got excited by all the talk about ontological contextual emergence. Re-reading clarified that you consider spacetime source elements as psi-epistemic and what is real are classical events. Is that correct? I also saw a line where you seemed to leave open that what is considered a “classical event” might be over-constrained. I will have to look for that quote.
(One lame thing about an e-book is you can’t mark up the margins etc. I would have marked that one)
I am still confused about how an entity can be psi-epistemic and ontological but maybe I finally start to understand the nuance of those terms.
and I am still curious where the Second Law fits in, if at all I guess, in the fundamental relational exchanges and the relative realism of the diachronic experience.
It also occurred to me while reading the chapter on emergence that non-linearity is a big feature of emergent phenomena (at least what I have read about them). And non-linear math is… not an easy or particularly useful place to start building the idea of math. So it’s not surprising human beings started with smooth differential manifolds, symmetry rules etc.
"
See Section 3.2.1 of this paper: https://www.mdpi.com/1099-4300/22/5/551/htm for the role of Newton's 2nd law. That's not in the book.
Ch 4 fig 4.20 re K. I want to get this question down because it seems clear re that diagram.
In RBW where “all contextual levels” interact… I buy the “boundary of a boundary is zero” math but I am struggling to imagine a single boundary that is really zero (an experiment with any real “spacetime source elements”) given the second law. You can artificially create one but only at the expense of displacing crucial entropy increasing interaction to some other level or boundary.
So for any real spacetime source element that boundary has be drawn such that entropy doesn’t cancel.
What I mean, is… isn’t that a cube with a temperature or entropy equivalent to “1 bit” or something like that. If it is real doesn’t it have to a spacetime “cost” vis a vis the AGC.
"
I don’t think of a spacetimesource element as “real”. It just represents the relational nature of quantum exchanges of energy-momentum.
I've been reading Part 3 of the book and it is very interesting indeed. I would love to explore the ideas further and check to make sure I have understood them. Is this thread the right place for that discussion, or would it be advisable to start a new one?
"
In a private conversation is best :-)
What is the name of the book again RUTA?
EDIT: Is this it – 'Beyond the Dynamical Universe: Unifying Block Universe Physics and Time as Experienced'?
"
Yes that’s it
We are getting nowhere. I certainly agree with the standard derivation of SR from the constancy of the speed of light in a vacuum. Please give me a reference for what you are talking about so I can educate myself, since obviously you refuse to discuss Bohmian Mechanics.
"
This article https://arxiv.org/pdf/2107.02089.pdf was just posted on another thread I've been following. You might be interested in reading Section 5.1 "De Broglie-Bohm theory"
We are getting nowhere. I certainly agree with the standard derivation of SR from the constancy of the speed of light in a vacuum. Please give me a reference for what you are talking about so I can educate myself, since obviously you refuse to discuss Bohmian Mechanics.
"
My Insight has nothing to do with the dBB interpretation nor have I ever published anything to do with dBB, so I don't know why you expected me to engage in a discussion of dBB. If you want to see how the relativity principle + h underwrites QM just as the relativity principle + c underwrites SR, see https://arxiv.org/abs/2106.12043 written for physics educators. It references our relevant publications if you're interested.
I am certainly happy that you are content to find an explanation of QM that satisfies you. However, gently, I will point out to you that in this lengthy discussion you have not been able to present a clear explanation of how allowing h to be variable solves any problem in understanding QM, … If physics is going to really explain how Nature works, it must be clear and understandable, not just the "hit-and-run" game of stating a nonobvious principle and then running away. If you are playing a game with me, Ruta, I do not like it much.
"
The relativity principle applied to the measurement of the speed of light c constitute the postulates of SR. As of now, 116 years after the publication of SR, we have nothing else to explain time dilation, length contraction, and the relativity of simultaneity. The relativity principle is not considered "nonobvious" in physics. On the contrary, it has been widely accepted since Galileo. What we showed in our publications (reviewed in this Insight) is that you can recover all of denumerable-dimensional QM with the very same relativity principle applied to the measurement of another fundamental constant of Nature, Planck's constant h. If you believe this principle account of QM lacks explanatory value, then you must also believe the postulates of SR lack explanatory value. You wouldn't be alone in that belief, but the vast majority of physicists disagree and all introductory physics textbooks introduce SR via its postulates.
In the double-slit experiment, a single photon traveling through a slit appears to be affected by a separate slit, through which it does not travel. Any interpretation must explain this apparent nonlocal mystery of Nature. The Standard Interpretation waves its hands and talks nonsense about how a particle can behave as a wave. Bohmian Mechanics does away with such nonsense.
There is a widespread bias against Bohmian mechanics, and it is usually based on ignorance and doubt. I hope you have thought deeply about the physics and are not merely expressing an unexamined belief.
"
In order to provide a "constructive" account of QM (as required for a proper "interpretation"), one will have to give up something cherished. That's what all the no-go theorems tell us. Since I love special relativity (I got my PhD in general relativity) and SR also lacks any constructive account, I have recently decided that I can live without a constructive account of QM as long as I can find a "principle" account of it as compelling as that for SR (relativity principle and light postulate). And, I have done so (explained in this Insight and others referenced therein). Indeed, this principle account of QM uses the same relativity principle applied to a different constant of Nature, h ("Planck postulate"). I'm content with that :-)
Thank you kindly for your clarification. I'm afraid I don't see the need for faster-than-light causal mechanisms in nonlocal pilot waves, as in it particle trajectories are deterministic, and which Bell supported, according to Making Sense of Quantum Mechanics, Jean Bricmont, Springer, 2016.
If such complaints are indeed common, perhaps you could be so kind as to throw just one citation my way. You would clearly be more likely to find one than I, since you know them to be common, but I don't (I'm not a professional physicist).
I would be grateful to see a criticism of Bohm's 1952 proposal that actually has some merit. Recall that all of physics turned against him, along with his former friend Robert Oppenheimer, when Joseph McCarthy found out that Bohm had briefly been a member of the Communist Party. In my opinion, political issues should not be mixed with physics.
"
There are lots of references for the non-locality given in the Wiki article https://en.wikipedia.org/wiki/De_Broglie–Bohm_theory
I am not aware that Bohmian Mechanics specifies a preferred intertial frame, the Ether. If you could provide a citation for this fact, especially in Bohm's 1952 paper, I would be very grateful. None of the rest of your comments appears to have any relationship to what I actually wrote. Quantum mechanics itself, to the best of my knowledge, does not "follow" from the existence of Planck's Constant, although it certainly makes use of it in the Schrödinger equation, which is the heart of QM. QM mostly "follows" the strange results seen in experiments involving the very tiny regime of electrons, photons, and even molecules. I do agree fully that SR follows from the constancy of c in all inertial frames.
"
dBB is non-local, i.e., it uses a faster-than-light causal mechanism (pilot wave). Thus, if you use dBB to explain Bell-inequality-violating QM correlations, you must pick a preferred frame to maintain definite causal ordering. It has nothing to do with the aether. This is a very common complaint against dBB, you can find it addressed in virtually every paper dealing with the dBB interpretation.
Putting SR aside for a moment, if we consider what could be "incomplete" about QM, there is one obvious candidate: the proposal in 1952 by David Bohm that the initial positions of the particles of an experiment be considered part of the definition of the experiment.
I think Einstein would have found Bohm's interpretation refreshing and in the spirit of a "completion" of QM, because it finally gives a deterministic basis in which a probability distribution is the emergent or computed result (rather than a mysterious axiom), just as the Maxwell-Boltzmann analysis based on molecular motion gives the temperature of a black body radiator as a probabilistic result, rather than as an experimental mystery.
"
The problem with Bohm is the use of a preferred frame. This looks a lot like the situation Einstein found himself in (quote from forthcoming in Am. J. Phys. by Moylan):
Even "Einstein was willing to sacrifice the greatest success of 19th century physics, Maxwell’s theory, seeking to replace it by one conforming to an emission theory of light, as the classical, Galilean kinematics demanded" before realizing that such an emission theory would not work (J. Norton, “Einstein’s Special Theory of Relativity and the Problems in the Electrodynamics ofMoving Bodies that Led him to it,” https://www.pitt.edu/~jdnorton/papers/companion.pdf). So, now that we know QM follows from the relativity principle applied to Planck's constant h, just like SR follows from the relativity applied to the speed of light c, (https://arxiv.org/abs/2106.12043), I have to believe Einstein would again defer to relativity principle :-)
(cited in our in our Scientific Reports paper and attached here). We reject the subjective nature of QBism, opting instead for one, self-consistent objective account of physical reality (represented by the block universe of shared, self-consistent classical information). And it makes perfect sense given that we're advocating for the fundamentality of principle explanation, i.e., principle explanation doesn't necessarily require a constructive counterpart. If you believe physics is in the business of identifying constraints on experience, as QBism claims, then the constraint "no preferred reference frame" is quite reasonable without a corresponding causal mechanism.
So, I am trying to get my head around the gauge-fixing part of the results from axioms. It seems relevant to the tension I am trying to describe. Just pretending for a second that there are all kinds of PO's not just people (or are people (with the ability to reflect upon and discuss the tension of consistency across TTO's somehow priveledged in the TTO graph vs. say rocks or muons) and being radically democratic with radical empiricism, say you have a couple of atomic PO's deep in the gravity well of the sun. This sun is cranking away with heat, pressure, gravity, geodesic stress – a regular hotbed of tension between said PO's so these two atomic PO's, say a couple of He atoms, are arguing long and loud. What happens next?[/quote]
POs are not necessarily human, they can be actual or hypothetical data collection devices, e.g., the probe that just landed on an asteroid or a hypothetical probe of some spacetime location inside a star.
"
I have the similar confusion with Alice and Bob for that matter. After all you are saying conservation only on average… so sometimes Bob get's the final h (Alice must simply agree) sometimes Alice get's it. Poor Bob. How is that represented in the TTO model?
"
Both Alice and Bob always measure h (##\pm \frac{\hbar}{2}## to be precise) for their spin measurements of the Bell spin state in question. Both are justified in saying the other is the one who has to average their outcomes to obtain the "correct" value of spin to satisfy conservation of spin angular momentum. Just like time dilation and length contraction where Alice(Bob) tells Bob(Alice) that his(her) clocks are slow and his(her) meter sticks are short, both are right. That is the "weird" consequence of conservation per NPRF, i.e., the relativity principle applied to the measurement of h.
I am hung up on how evolution, a dynamical view if ever there was, and the ultimate bully idea of dynamical time fits into the adynamical Lagragian description of things in your view?
"
Dynamical explanation in physics is still absolutely legit in our view, it's just not fundamental. In my opinion, anything that can be explained dynamically should be, since our experience is dynamical. I only resort to the more fundamental principle/adynamical/Lagrangian explanation as a last resort, e.g., in dealing with closed timelike curves, origin of the universe, delayed choice, etc. All this is covered in our book, as you'll see. Chapters 7 & 8 cover the reconciliation of our dynamical experience of time with adynamical or block universe physics. You can also read our Entropy paper. At 35 pages it's long, but still shorter than Chapters 7 & 8
I'm not sure about all aspects of the analogy between SR and QM that is described in the paper.
I agree that all observers in relativity having to measure ##c## for the speed of light, regardless of their state of motion, is analogous to all observers in QM having to measure ##\pm \hbar / 2## for spin, regardless of their choice of measurement direction. And I agree that the latter fact requires that, when analyzing conservation of angular momentum in QM, the best we can possibly do is the "average conservation" that the paper describes.
I'm not sure how length contraction or time dilation correspond to the spin "corrections" that have to be made to verify "average conservation", since length and time aren't conserved quantities and the contracted lengths and dilated times that a given observer assigns to objects in motion relative to him are not "corrections" applied to any calculation of conservation.
I'm wondering, though, if the latter issue could be addressed by looking at energy and momentum instead of time and length, since they are "corrected" by the same factors and they are conserved quantities. That would still leave as a difference between SR and QM the fact that the SR conservation laws are not average only.
"
The "conservation part" of the mystery of entanglement is not found in the mysteries of time dilation and length contraction. The fact we are pointing out in our Scientific Reports paper (as outlined in the ScienceX News article) is that all of these mysteries stem from the application of the relativity principle ("no preferred reference frame" NPRF) to a fundamental constant. And while this principle explanation of the mysteries is true (principle explanation = mathematical consequences of empirical facts), there are still no (consensus) constructive accounts. And these mysteries are very very old. So, what do we make of these facts?
As I suggest in the ScienceX News article, perhaps we don't always need an explanation based on a causal mechanism. That's where the ScienceX News article ends, but we go further in our Scientific Reports paper. There we suggest that principle explanation is just as valid as constructive explanation when physics is viewed per Mermin's QBism (cited therein):
In other words, physics provides constraints on experience. If that's the way you view physics, then a constraint such as NPRF is very reasonable. That's where the Scientific Reports paper ends, but we go further in our Entropy paper. In that paper, we provide a mathematical model of consciousness whereby all of physics follows from two axioms (empirical principles), one of which is NPRF. And that shows how the mysteries of physics are related to the hard and combination problems of consciousness, and how neutral monism resolves them all. The bottom line: the physics we have is beautifully coherent and comprehensive (although not finished, since we still need quantum gravity of course).
I had heard of CHSH but there was a lot in that answer I didn't understand at all. In fact I hadn't even heard of "superquantum correlations". This paper was a pretty good intro to the idea… in case it's valuable to anyone else.
Maybe those variables are hidden by the 5D aliens so we won't realize there is no such thing as time and will keep trying to make lots of important decisions (that's a joke, in case it's not clear).
https://arxiv.org/abs/1205.1162
Classical, quantum and superquantum correlations
GianCarlo Ghirardi, Raffaele Romano
"
You might also like to read this Insight which explains an "unreasonable consequence" of superquantum correlations as presented in Bub and Bub's book.
I'm not surprised you haven't heard of superquantum correlations. I started working in foundations in 1994 and didn't hear about superquantum correlations until 2018. We published a book on foundations of physics in 2018 and Jeff Bub asked us to give a talk on it for his seminar. After a few hours of discussion we were walking from the restaurant to our car when Bub said, "What I really want to know is, Why don't we observe superquantum correlations? They don't violate no-superluminal-signaling, so why are they not found in Nature?" Silberstein and I didn't know what he was talking about. Answering his question (they violate "conservation per NPRF") is what ultimately led to this Insight.
I've seen some statements in for example, Misner and Thorne's textbook "Gravitation", to the effect that self-interactions of a gravitational field cannot be an independent source of gravitational effects, that gravitational energy cannot be localized, and other details that don't seem to be consistent with Deur's approach. They look like no go theorems inconsistent with his approach but that may simply over read what they really mean.
"
The bolded phrase is correct. I wrote up a series of Insights articles on this very topic:
https://www.physicsforums.com/insights/does-gravity-gravitate/
The short version is, the answer to the title question (does gravity gravitate?) can be either yes or no, depending on how you interpret it. The things MTW is talking about are part of the interpretation of the question that leads to a no answer. But the way Deur is using nonlinear effects is part of the interpretation of the question that leads to a yes answer. Both interpretations are valid; they just show that the question itself as originally stated is ambiguous, and the ambiguity can be resolved into two different questions that have different answers.
there is debate over whether the cosmological constant amounts to violation of conservation of mass-energy as the amount of dark energy increases when the volume of the universe does, or whether that is really a trade off between gravitational potential energy of some sort and dark energy
"
That debate isn't just over the dark energy case, although that's the case Sean Carroll chose to use to illustrate the issue in his blog post about "energy is not conserved". The debate applies to any spacetime that is not stationary, including the many non-stationary spacetimes with no cosmological constant (such as all of the FRW spacetimes with no cosmological constant).
So while I agree that not having to have a cosmological constant is a nice feature, I don't think Deur's model "solves" any general problem about "mass-energy not being conserved in GR". The same problem, if you think it's a problem, is there in FRW spacetime with no cosmological constant, which is basically where Deur's model would end up as a model of the universe as a whole (modulo some corrections due to the other effects in his model, that also don't affect the "conservation" issue as far as I can see).
"
This is good for a quantum gravity theory because in a quantum gravity theory you'd like to have everything arise locally and no global effects like the cosmological constant.
"
The cosmological constant in GR is not "global"; it's just non-dynamical–it's an extra effective energy density that is just there regardless of anything else that happens. In quantum field theory it just corresponds to a nonzero vacuum expectation value of energy, which is a perfectly good local quantity.
The last sentence leads to what I think is a better way to describe what problem is solved by not having to have a cosmological constant. All attempts to estimate from QFT what a nonzero vacuum expectation value of energy should be, if there is one, come up with answers 120 or so orders of magnitude larger than the observed value they are supposed to be explaining. Deur's model makes that problem go away, which is certainly nice, but again, I don't think "solving a problem with mass-energy not being conserved" is a good way to describe why it's nice; "eliminating the problem of the huge mismatch between theoretical and experimental values of the cosmological constant" would be better.