How Does an Airplane Wing Work? A Primer on Lift
Many people ask how an airplane wing works, and several answers are commonly given. You might have even seen vigorous arguments between proponents of competing theories, such as Bernoulli’s principle and Newton’s laws. So who is correct? First, we must discuss what Bernoulli’s equation means.
Table of Contents
Bernoulli’s equation
First, what is Bernoulli’s principle? Bernoulli’s principle (or Bernoulli’s equation) is essentially a statement of conservation of energy for a moving fluid that relates the velocity of a fluid to its pressure. Its most common form is
[tex]p + \dfrac{1}{2}\rho v^2 + \rho gz = \mathrm{constant} = p_{\mathrm{t}}.[/tex]
Here, ##p## is the static pressure, ##p_{\mathrm{t}}## is the total pressure, ##\rho## is the density, ##v## is the velocity, ##g## is the acceleration due to gravity, and ##z## is the height. The static pressure essentially represents a form of potential energy per unit volume. The total pressure represents the total energy per unit volume in the system. The term ##^1/_2 \rho v^2## is called the dynamic pressure and is the kinetic energy per unit volume.
This expression is only valid under a set of very limited circumstances, specifically for steady, incompressible, inviscid flow (or along a streamline). There are various other more complicated forms, but this serves to illustrate the physics just fine. Often, this equation is used to compare the pressure and velocity at two different points. In the case of an airfoil where the change in ##z## is very small and can be ignored, this is often written as
[tex]p_1 + \dfrac{1}{2}\rho v_1^2 = p_2 + \dfrac{1}{2} \rho v_2^2.[/tex]
You could rearrange this instead to say
[tex]p_2 = p_1 + \dfrac{1}{2}\rho ( v_1^2 – v_2^2 ).[/tex]
In other words, if ##v_2 > v_1##, then ##p_2 < p_1## and vice versa. There is therefore an inverse relationship between velocity and pressure, which shouldn’t be surprising in light of the energy conservation nature of the equations.
Many of the explanations of lift use this principle to explain how the air moves faster over the top of the airfoil, therefore it has a lower pressure than the bottom, resulting in a net force upward. While this is not, in general, incorrect, it does lend itself to several fallacies. With this in mind, a few incorrect explanations for lift must next be discussed.
Common lift fallacies
Equal transit time
One of the most common myths associated with lift on an airfoil is that of the “equal transit time” theory. In this common explanation, someone may claim that the length of the path over the top of the airfoil is longer, so the air over the top must speed up to travel to the end of the airfoil at the same time as the air on the bottom. The problem with this explanation is that nothing forces the air on the top and bottom to traverse the airfoil in the same amount of time. The air over the top generally travels much faster than that on the bottom and leaves the airfoil well before the air underneath. This fact is illustrated in the following video. For a more detailed breakdown of this issue, see this NASA article.
Venturi effect
Another common explanation for why air might move faster over the top is by comparing the flow over an airfoil to that through a Venturi nozzle. The idea is that you can draw a horizontal line above the airfoil in the free stream representing a streamline, and the resulting shape looks somewhat like a Venturi tube with a constriction in the middle created by the airfoil. Conservation of mass would then imply the air speeds up, resulting in a low pressure over the top.
The first problem here is that this does not correctly predict the velocity observed over the top of an airfoil. An airfoil is not a Venturi tube and there is no magic surface above it that artificially constricts the flow. If you arbitrarily choose a horizontal streamline above (or below) an airfoil, the answer you get will change depending on which streamline you pick. Further, that answer will only give some average velocity in that section of your “tube” and will likely only be very slightly faster than the free stream, especially if you pick your arbitrary surface far away from the airfoil.
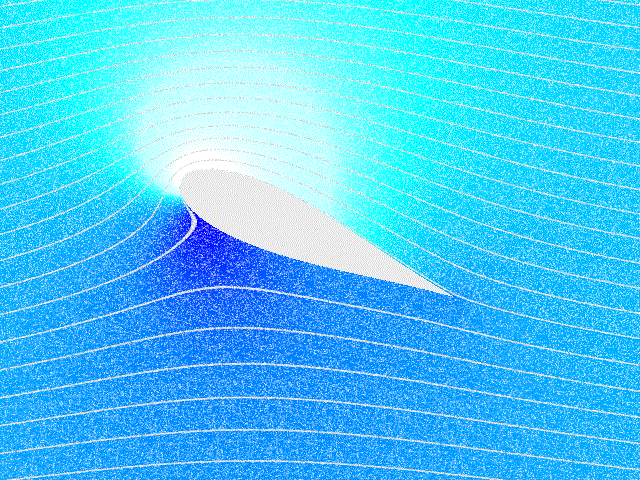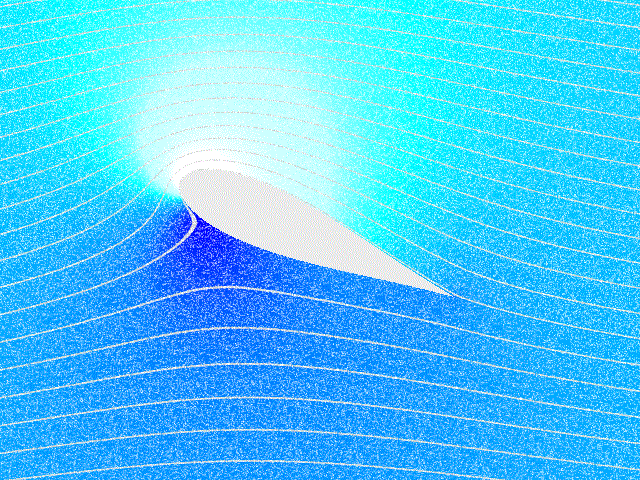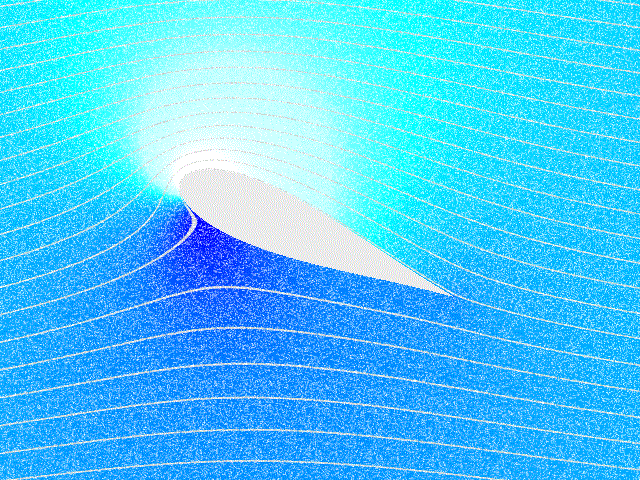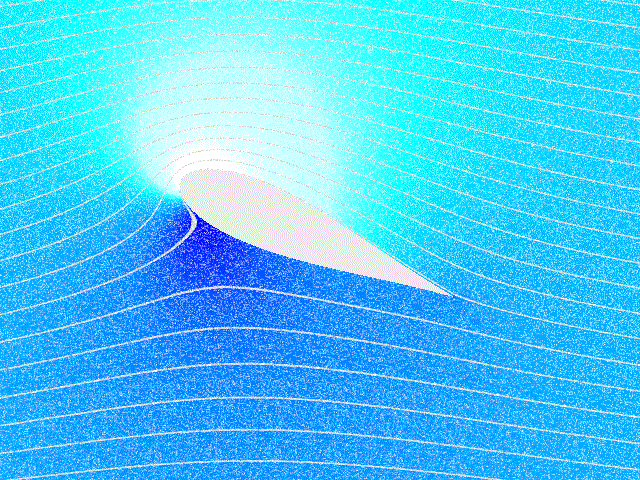
Second, this ignores the effect of the bottom surface, which would naturally constrict the flow below as well using the same logic. This would lead to a lower than ambient pressure on the bottom of the airfoil as well, leading to either negative or positive lift depending on the shape. In reality, it is often (but not always) the case that the pressure below a wing is higher than the ambient pressure. For one example, see the following image, where darker blue means higher pressure.
The Venturi theory could not predict such a case even though it does occur.
Now, for all that is wrong with the usual Venturi theory, there is one circumstance where it would work. By definition, streamlines in a flow field have no mass flow crossing them. Therefore, if you pick two arbitrary streamlines in any steady flow field, they form what is called a stream tube through which mass is conserved. In this case, the Venturi effect would apply (in the sense of average velocity). So, for a wing, if you happen to know the streamlines (or function) beforehand, then you can simply select the streamline at the surface of the airfoil and one streamline above it somewhere and it will give you the correct average velocity, and as the upper streamline you choose gets asymptotically closer to the surface, your average velocity will approach the exact velocity near the surface. Of course, all of the streamlines are generally curved, even a great distance away from the airfoil, and this approach requires prior knowledge of the flow field to work. The only way to obtain the required information about the flow field to calculate streamlines is to take a different approach.
In essence, the Venturi effect cannot explain or calculate lift except in the specific case where you already know the flow field by other means and can calculate the streamlines, and in that case, since you already know the flow field, doing any sort of analysis based on Venturi is redundant and not useful. For more on the problems with the Venturi theory, as it pertains to lift, NASA has another article to browse.
The skipping stone theory
One other common misconception can be described as the skipping stone theory. The general idea is that an airfoil moves over a layer of air in much the same way a stone can move over the surface of a pond and skip rather than sink. While this idea does get one thing correct: namely that lift can be described as a reaction force to the deflection of air, it completely ignores the effect of the upper surface, which plays an important role. For more on this theory, see yet another NASA article on the topic.
So where does lift come from?
The simple answer: Newton
This brings us back to our original question: where does lift come from? The simplest answer is that an airfoil (or any lift-generating shape) bends the airflow downward. This is a change in momentum, which, per Newton’s laws, requires a force to be exerted on the air by the airfoil. The equal and opposite force to this is lift.
The practical answer: Bernoulli
However, before you, Newton fans declare total victory, keep in mind that Bernoulli’s equation is also correct when explaining lift. If we start off with the assumption that the air does move faster over the top of the airfoil than the bottom, then Bernoulli’s equation does predict a pressure difference and then a net force. This fact is extraordinarily practical because, unlike the downwash produced by an airfoil, it is quite easy to measure the velocity over an airfoil and convert that to pressure to calculate lift or to just measure the pressure directly.
A quick note on viscosity: Some astute observers may point out that real air flows are viscous, so Bernoulli’s equation should not apply. While the former is true, Bernoulli’s equation is still very useful due to a phenomenon called the boundary layer. The effect of viscosity is confined to a very small region very near the surface of an object, and in the direction normal to the surface, the pressure is constant. Therefore, if one uses Bernoulli’s equation to predict the pressure distribution just outside of the boundary layer where viscosity is not important, they are effectively calculating the surface pressure distribution as well and therefore can calculate the lift.
Why does the air move faster over the top, and for that matter, how do we know how much downwash is produced?
It turns out, these two questions are related, and also why lift is incorrectly explained so often. It also turns out that the answer to this question is extraordinarily complicated. The simplest answer is that inviscid theory predicts one stagnation point at the front where the air impacts the tip of the airfoil, and one at the back at some arbitrary point that would result in no lift and no flow deflection. However, real life is not inviscid. Airfoils take advantage of this fact by incorporating design features that are specially designed to cause the stagnation point on the downstream end of the airfoil to occur at the trailing edge. This is usually done by making the trailing edge effectively sharp, but can also be achieved through other means (see: flatback airfoil). Since that trailing edge stagnation point has now been “artificially” fixed, the airflow must accelerate or decelerate in various places to ensure that the conservation laws (conservation of mass, momentum, and energy) are satisfied. This results in a much faster velocity over the top of the airfoil and a deflection of the flow downward.
So, in the end, Newton fans: you are correct! Bernoulli fans: you are also correct (provided you didn’t invoke equal transit time or Venturi)!
PhD – Fluid mechanics, nonlinear dynamical systems, hydrodynamic stability.
Swimming, grilling, beer, movies, watching most sports, playing a few, and a dash of video games








Leave a Reply
Want to join the discussion?Feel free to contribute!