Blockworld and Its Foundational Implications: The Relativity of Simultaneity and Blockworld

 

In part 1 of this 5-part Insights series, I introduced two consequences of the second postulate of special relativity (SR): time dilation (“moving clocks run slow”) and length contraction (“moving objects shrink”). Since moving clocks run slow, if you and observers at rest with respect to you (hereafter simply “you”) see me moving, you should see me aging more slowly than you. But motion is relative, so I (and observers at rest with respect to me) say you’re the ones moving and therefore we should see you aging more slowly than us. Likewise, since moving objects shrink, I must say your meter sticks are shorter than one meter while you must say my meter sticks are shorter than one meter. Here in part 2 of this series, I will explain how the relativity of simultaneity (RoS) resolves these apparent contradictions, but at a cost called “blockworld.” According to blockworld (aka “block universe”), the past and future are just as ‘real’ as the present. I will do this by telling a story about twin boys who see triplet girls moving relative to them at high speed (Figure 1).

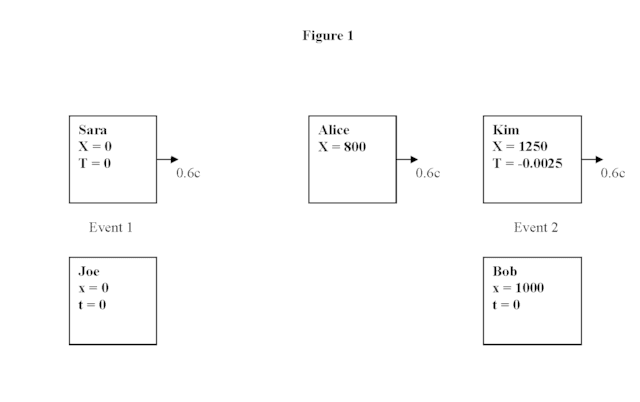
The adaptation I make to the DeWitt/Mermin version of this story[1] is to consider only local observations. I do this to emphasize that the blockworld (BW) implication of the RoS is not an illusion resulting from the finite speed of light. In order to keep our observations local I introduce three new characters – Bob, Alice and Kim – in addition to Joe and Sara of the DeWitt/Mermin version. The boys, Bob and Joe, are at rest with respect to each other and their clocks are synchronized (let them be twins). Likewise, the girls are at rest with respect to each other and their clocks are synchronized (let them be triplets). Joe and Bob see the girls moving in the positive x direction at 0.6c (Figure 1). The girls, therefore, see the boys moving in the opposite direction at 0.6c (Figure 3). Who is actually moving?

The answer to this question is central to the BW perspective. According to the first postulate of SR, there is no way to discern absolute, uniform motion (the laws of physics are the same in all inertial frames of reference), so either perspective is equally valid. The girls are correct in saying it is the boys who are moving, and vice-versa. This is equivalent to saying there is no preferred, inertial frame of reference in the spacetime of SR. Now we compare some observations and their consequences.

Joe is located at x = 0 (lower case coordinates are the boys’) and Bob is at x = 1000km. When Joe passes Sara their clocks read T = t = 0 (upper case coordinates are the girls’) and Sara is located at X = 0, so X = x = 0 when T = t = 0. To find Kim’s coordinates for when Bob passes her (x = 1000km, t = 0), SR gives (Figure 1):

\begin{equation} T = \gamma \left(t \, – \frac{vx}{c^2} \right) = 1.25 \left(0\,  – \frac{0.6c(1000)}{c^2} \right) = -0.0025\mbox{s}   \label{girlsT2} \end{equation}
\begin{equation} X = \gamma \left(x \, – vt \right) = 1.25 \left(1000 \, – 0.6c(0) \right) = 1250\mbox{km}   \label{girlsX2} \end{equation}

where c = 300,000km/s and

\begin{equation} \gamma = \frac{1}{\sqrt{1 \, – \frac{v^2}{c^2}}} = \frac{1}{\sqrt{1 \, – \frac{(0.6c)^2}{c^2}}}  = 1.25   \label{gamma} \end{equation}

The girls and boys agree with all the clock readings, so what’s the problem?

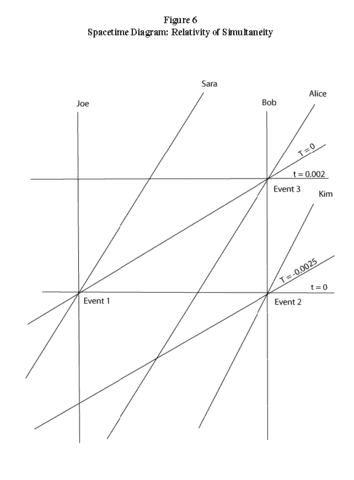
Let’s translate these clock readings to ages (in exaggerated fashion). Let T = t = 0 equate to an age of 20 years and let 0.0025s equate to 2.5 years from the temporal origin. Then 20 year-old Sara kisses 20 year-old Joe (Event 1) and 17.5 year-old Kim kisses 20 year-old Bob (Event 2). Since the boys believe Events 1 & 2 are simultaneous (Figures 1 & 6), they conclude that Kim is 2.5 years younger than her sister, Sara, so the girls are not triplets. Further, the girls’ claim that Kim and Sara are 1250km apart is not consistent with the boys’ claim that they’re only 1000km apart (again, this difference in spatial separation is called length contraction). Who’s right?

Neither of their frames is preferred, so the girls and the boys are correct! Whether or not space-like separated events (Figure 2) are simultaneous is relative to the frame of reference. So, what is the consequence of this “relativity of simultaneity?”

RoS renders the view known as “presentism” highly suspect. Presentism is the belief that everyone shares a unique, ‘real’ present while their common past states no longer exist and their common future states are yet to exist. Per presentism, everyone could agree with a statement such as, “Sam is surfing in California while Jonathan is shoveling snow in New York.” If Sam is 25 years old, the 24 year-old version of Sam no longer exists, i.e., is no longer ‘real’, and the 26 year-old version will not exist, i.e., will not be ‘real’, for another year. There is a sense that we share in a ‘real’ present moment with everything else in the universe and this attribute of ‘realness’ moves along all worldlines synchronously. [If you’re not familiar with the notion of a “worldline,” see the Tabletop Explainer video https://www.youtube.com/watch?v=gVGSK1Mb3Po.] In fact, what we mean by “the universe” is vague unless everyone agrees on a spatial surface of simultaneity in spacetime. But, as we continue with our example it should become clear that the RoS is contrary to this commonsense notion of presentism. Let us continue.

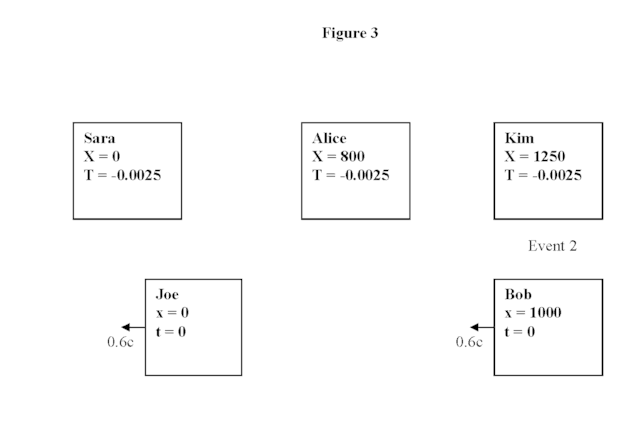
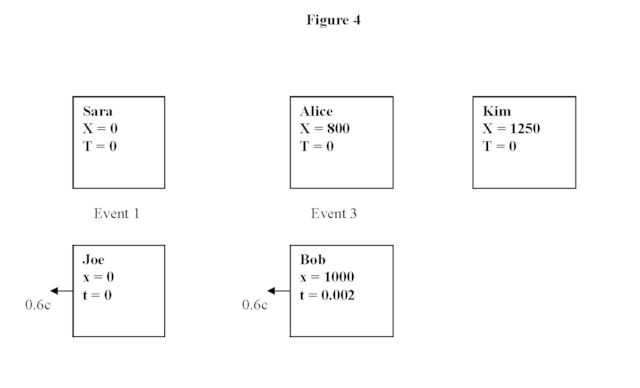
Since Kim’s clock reads T = -0.0025s at Event 2, the girls say Bob passed Kim 0.0025s before Joe got to Sara (Figure 3). The event simultaneous with Event 1, per the girls, is Bob passing Alice (Event 3, Figure 4). All agree that Alice’s clock reads T = 0 when Bob is there, but they also agree that Bob’s clock reads t = 0.002s when he is at Alice’s position.

\begin{equation} T = \gamma \left(t \, – \frac{vx}{c^2} \right) = 1.25 \left(0.002 \, – \frac{0.6c(1000)}{c^2} \right) = 0   \label{girlsT3} \end{equation}
\begin{equation} X = \gamma \left(x \, – vt \right) = 1.25 \left(1000 \, – 0.6c(0.002) \right) = 800\mbox{km}   \label{girlsX3} \end{equation}

That is, per the girls, Bob was 450km away from Alice at T = -0.0025s and moving at 0.6c. Since it took him 0.0025s to get from Kim to Alice, i.e., $\frac{450}{0.6c} = 0.0025\mbox{s}$, Alice’s clock reads T = 0 when Bob passes her. Here’s how the girls tell the story.

Sara is at X = 0, Alice is at X = 800km and Kim is at X = 1250km. The boys are not 1000km apart, as they claim, but rather only 800km apart (again, length contraction). The girls know this since Bob was at Alice’s position (X = 800km, T = 0) simultaneous with Joe being at Sara’s position (X = 0, T = 0). Thus, the girls say the boys’ distances are exaggerated, while the boys say the same about the girls’ distances (recall above the boys measure the distance between Sara and Kim to be 1000km, not 1250km as the girls claim). So, per the girls, Bob passed Kim (Event 2) before Joe got to Sara (Event 1). Notice also that while the girls aged 0.0025s (Bob at Kim at T = -0.0025s and Bob at Alice at T = 0), Bob only aged 0.002s; the girls see the boys aging more slowly than themselves. But, since the boys say it’s the girls who are moving, it must be the case that the boys see the girls aging more slowly. This will be verified below via observations of Sara. Now for the million dollar question, who shares the attribute of ‘realness’, i.e., where is the spatial frame of ‘realness’ which defines “the universe” at any given time?

Well, unless we can touch things which are not ‘real’, Joe and Sara are ‘co-real’ at Event 1, and Bob and Kim are ‘co-real’ at Event 2 (Figure 6). But, Joe and Bob are ‘co-real’ at Events 1 & 2 (t = 0 for both) so we see that all four characters involved in Events 1 & 2 are ‘co-real’. This means Sara shares ‘realness’ at T = 0 with Kim at T = -0.0025s, and Sara shares ‘realness’ at T = 0 with Kim at T = 0. Thus, Kim at T = 0 shares ‘realness’ with Kim at T = -0.0025s, so the RoS implies the past is as ‘real’ as the present. Now for more on the boys’ perspective.

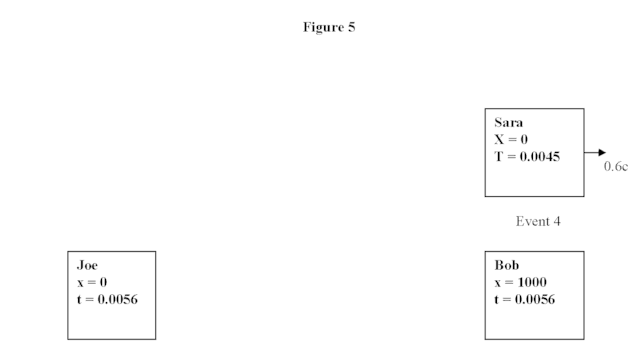
Joe is at x = 0 and Bob is at x = 1000km. Sara and Kim passed the boys (Events 1 & 2) simultaneously (t = 0), so as stated supra, Joe and Bob do not believe Sara and Kim are 1250km apart, but only 1000km apart (length contraction). Alice passed Bob 0.002s later, so she must be (0.6c)(0.002s) = 360km behind Kim (not 450km as the girls claim – more length contraction). As for how fast the girls are aging as viewed by the boys, Sara, Joe and Bob were all 20 years-old (T = t = 0) when Sara kissed Joe. Sara is 1000km away from Bob at t = 0 and is moving at 0.6c, so she arrives at Bob at $t = \frac{1000}{0.6c}= 0.0056\mbox{s}$. What are the girls’ coordinates for this event?

\begin{equation} T = \gamma \left(t \, – \frac{vx}{c^2} \right) = 1.25 \left(0.0056 \, – \frac{0.6c(1000)}{c^2} \right) = 0.0045\mbox{s}   \label{girlsT4} \end{equation}
\begin{equation} X = \gamma \left(x \, – vt \right) = 1.25 \left(1000 \, – 0.6c(0.0056) \right) = 0   \label{girlsX4} \end{equation}

Notice first that Sara’s spatial position is calculated to be X = 0, as must be the case. Second, notice that she has aged only 4.5 years (exaggerated time) between the time she kissed Joe (Event 1) and the time she kissed Bob (Event 4, Figure 5), while the boys aged 5.6 years between these events. Thus, as promised above, the boys see the girls aging more slowly than themselves. Finally, notice that at Event 3 Alice shares ‘realness’ with Bob while she shares ‘realness’ with Sara (both T = 0, Figures 4 & 6). However, at T = 0 Sara also shares ‘realness’ with Joe (both at Event 1). Thus, Bob at t = 0 shares ‘realness’ with Bob at t = 0.002s, so the RoS implies the future is as ‘real’ as the present.

In conclusion, the boys say the girls’ clocks run slow and the girls’ meter sticks are short, while the girls say exactly the same thing about the boys’ clocks and meter sticks. These seemingly incompatible facts can hold because the boys and girls disagree as to which events are simultaneous – Events 1 & 2 are simultaneous for the boys, Events 1 & 3 are simultaneous for the girls (Figure 6). This relativity of simultaneity implies the past, present and future must co-exist, i.e., reality is blockworld. You may like to watch this excerpt from Nova’s program, “The Fabric of the Cosmos: The Illusion of Time” https://www.youtube.com/watch?v=vrqmMoI0wks. However, clearly we have dynamical experience and the blockworld model of spacetime maps to that dynamical experience, so it cannot possibly be the case that the blockworld model of spacetime contradicts our dynamical experience of time. As it turns out the offending assumption in the above argument in that of the transitivity of ‘realness’, at least as it concerns dynamical experience. See this Insight for details. Nonetheless, the blockworld model of spacetime does invoke constraints on that dynamical experience that are spatiotemporal as opposed to “dynamical,” as we will see in this series.

In the next (third) part of this Insights series, I will (conceptually) introduce general relativity and bring BW to bear on general relativistic cosmology to ‘explain’ what puzzles so many people about big bang cosmology, i.e., the origin of the universe.

The girls are triplets and the boys are twins. 20 year-old Joe kisses 20 year-old Sara and 20 year-old Bob kisses 17.5 year-old Kim (exaggerated times). The boys and girls agree on those facts, so Events 1 & 2 occur at the same time for the boys and they conclude the girls are not the same age. Also, the boys measure the distance between Sara and Kim as 1000km, not 1250km as the girls measure it, so the boys say the girls’ distances are length contracted.

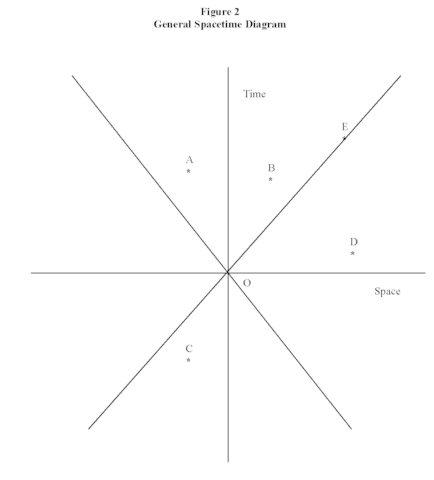
If you’re unfamiliar with a spacetime diagram, watch the 3-minute video https://www.youtube.com/watch?v=gVGSK1Mb3Po. Events A, B, and C are time-like related to the origin O. Event D is space-like related to O and Event E is light-like related to O.

The girls are triplets and 20 year-old Joe kisses 20 year-old Sara (Event 1) and 20 year-old Bob kisses 17.5 year-old Kim (Event 2). The boys and girls agree on those facts, so the girls don’t believe Events 1 & 2 occur at the same time, i.e., Event 2 occurred 2.5 years before Event 1.

The girls are triplets and 20 year-old Joe kisses 20 year-old Sara (Event 1) and 20 year-old Alice kisses 22 year-old Bob (Event 3). The boys and girls agree on those facts, so the girls say Events 1 & 3 occur at the same time and the boys are not the same age. Since 17.5 year-old Kim kissed 20 year-old Bob (Event 2), the girls aged 2.5 years between Events 2 & 3 while Bob only aged 2 years, so the girls say the boys are aging more slowly than they are. Also, the girls measure the distance between Joe and Bob as 800km, not 1000km as the boys measure it, so the girls say the boys’ distances are length contracted.

The boys are twins and 20 year-old Joe kisses 20 year-old Sara (Event 1) and 25.6 year-old Bob kisses 24.5 year-old Sara (Event 4). The boys and girls agree on those facts, so the boys aged 5.6 years between Events 1 & 4 while the girls only aged 4.5 years. Therefore, the boys say the girls are aging more slowly than they are.

Events 1 & 2 are simultaneous for the boys (both occurring at t = 0) while Events 1 & 3 are simultaneous for the girls (both happening at T = 0).

1. R. DeWitt, Worldviews: An Introduction to the History and Philosophy of Science (Blackwell Publishing, United Kingdom, 2004), 213-219. DeWitt says his example “owes much to” N.D. Mermin, Space and Time in Special Relativity (McGraw-Hill, New York, 1968).

Note: Comments for this post have been disabled. Please show your feedback by using the star rating at the top of the article and or contacting the author personally.