Emergence From the Superpoint
Show Complete Series
Part 1: It Was 20 Years Ago Today — the M-theory Conjecture
Part 2: Homotopy Lie-n Algebras in Supergravity
Part 3: Emergence from the Superpoint
Part 4: Why supersymmetry? Because of Deligne’s theorem
Part 5: 11d Gravity from just the Torsion Constraint
Part 6: Spectral Standard Model and String Compactifications
Part 7: Super p-Brane Theory emerging from Super Homotopy Theory
In the previous article we saw that the generalization of super-Lie algebras to homotopy super-Lie n-algebras (super L-infinity algebras) has been found, decades back, to be at the heart of supergravity and M-theory — somewhat secretly so, in the dual guise of “FDA”s.
Lie algebras are infinitesimal symmetries.
Super Lie algebras are infinitesimal supersymmetries.
Lie n-algebras are infinitesimal higher symmetries-of-symmetries.
Super-Lie n-algebras are infinitesimal higher supersymmetries-of-supersymnetries.
Here in this article, we explore the landscape of super Lie n-algebras. We do so by starting at the root, the atom of supergeometry, the single superpoint, regarded as the simplest non-vanishing super Lie algebra. By looking at it from the perspective of homotopy theory, we find that there emerges out of it a bouquet of super-Lie n-algebra extensions. This bouquet, purely Lie n-theoretic as it is, turns out to be the system of super-spacetimes and of all the super p-branes propagating on these, as they appear in string/M-theory. The diagram looks as follows, with each extension corresponding to a super-spacetime or to a super p-brane in string/M-theory (below I explain how this works and what it means — all the ingredients are kf if not presented in this super Lie n-algebraic form):
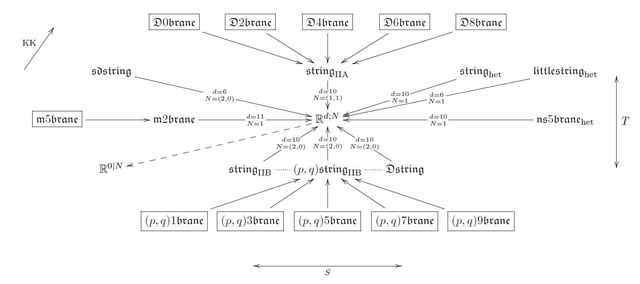
While being a purely mathematical object in super Lie n-algebra theory, this system is congruent to the famous cartoon map of M-theory (Witten 97):

But as opposed to that cartoon, the super Lie n-algebraic brane bouquet has a mathematical existence. Homotopy Lie theorists of the 21st century, classifying super Lie n-algebras, would eventually have found this diagram themselves, had string theory not fallen into the 20th century to scoop them.
If time permits, then I will discuss in the following articles here how from that diagram that looks like M-theory, one actually gets what is known about M-theory… and maybe a bit more. But today I focus just on how the diagram itself appears.
To that end, we start from nothing but the superpoint.
In the beginning there is supergeometry, with its atom of space, the superpoint.
I’ll talk another day about why that is so. The curious or impatient reader finds the mathematical details behind this curious statement in these notes, other readers are invited to enjoy the fact that this is all that I am going to assume. The rest follows. For more details on the supergeometric concepts that I will mention in the following, you could also check the nLab entry on super-Cartan geometry.
So we have in our hands a tiny little gem called the superpoint. Let’s have a closer look at it.
From the fundamental point of view of homotopy theory, every single object has a potentially rich inner structure, whose unraveling is called its Postnikov-Whitehead tower. Rich as it is, and complicated as it may seem to the uninitiated, the beautiful fact about it is that it is a god-given fundamental phenomenon which just is, we are not to worry but just to watch it do its thing.
And here is what it does. Very roughly, the Whitehead tower of any higher Lie theoretic object works like this: check which 2-cocycles the object carries, and form the Lie extension by those 2-cocycles. This should sound familiar. But it is just the first step in a higher pattern. Next, we are to check which 3-cocycles that extension itself carries and form the extension by the 3-cocycle. It is a simple but maybe not widely enough known fact that higher (n+1)-cocycles classify n-algebra extensions (namely their homotopy fibers) just as the familiar 2-cocycle classify plain algebra extensions. So for n=3 the extension we obtain is now a Lie 2-algebra. Then next we check for 4-cocyles on this Lie 2-algebra and extend by these… this yield a Lie 3-algebra. On that, we check for 5-cocycles and extend by them to obtain a Lie 4-algebra. And so forth. The rough idea should be clear, more details may be found in (Sati-Schreiber-Stasheff 08).
Back to the superpoint. Regarded as an abelian super Lie algebra, simplistic as that is, it happens to carry a non-trivial 2-cocycle. Namely when we denote by “theta” the single fermionic coordinate function on the superpoint, then this cocycle is the one given by the left-invariant super-2-form
d theta /\ d theta .
If theta were bosonic then this would vanish, but since it is fermionic this is indeed non-vanishing. And so the miracle begins.
What is the extension classified by this 2-cocycle? This is pretty trivial, but still worthwhile to make explicit: this extension is the N=1 d=1-dimensional supersymmetry algebra. The worldline of the superparticle.
Next, consider the Cartesian product of the superpoint with itself, the “square superpoint”. This has two odd coordinate functions on it, say theta1 and theta2. Accordingly, it has a 3-dimensional space of 2-cocycles, spanned by these left-invariant 2-forms:
d theta1 /\ d theta1
d theta1 /\ d theta2
d theta2 /\ d theta2
Extending by these three cocycles gives a bosonic-3-dimensional extension of the square superpoint. Which one could this be? Here a slightly more substantial miracle happens: that extension turns out to be N=1, d=2+1 super-Minkowski spacetime, with its supersymmetry super Lie algebra structure. (This follows by inspection of the nature of real spin representations in 2+1-dimensions, the details are spelled out here.)
We proceed to inspect the square superpoint in homotopy theory. After we have extended by the 2-cocycles, we are to look for 3-cocycles on the resulting extension. There are a few generic 3-cocycles, but there is one exceptional 3-cocycle on 2+1-dimensional super-Minkowski spacetime, which exists due to an instance of a Fierz identity. This 3-cocycle is best known in the literature as the curvature of the WZW term of the Green-Schwarz superstring in 3d.
In the physics literature, this cocycle is discovered as being the relevant structure to ensure a certain consistency condition of Green-Schwarz sigma-models known as kappa-symmetry. This is important, but for the moment we ignore all physics hindsight and let the pure abstract substance reveal itself.
What it reveals next is a super Lie 2-algebra extension of 3d super-Minkowski spacetime, namely the homotopy fiber of that 3-cocycle. This has accordingly been called the superstring super Lie 2-algebra or similar. (A gentle exposition of how this works is in Baez-Huerta 10).
So far we have seen 2+1-dimensional super-Minkowski spacetime together with the superstring in this dimension grow out of the superpoint. Given that structure so far, it is natural to ask which subgroup of the supergroup of linear symmetries of 3d super-spacetime preserves this, i.e.the subgroup the stabilizes the supersymmetry Lie bracket as well as the cocycle. This subgroup turns out to be… the spin-cover of the Lorentz group, Spin(2,1). (John Huerta has noticed a neat quick proof of this fact, interested readers may find it here.)
Hence Lorentzian geometry — relativity — emerges out of just the superpoint. At least in 3d so far… but let’s keep watching what the process produces next.
In fact Spin(2,1) sits in two different inequivalent ways inside all linear symmetries of 2+1-dimensional super-Minkowski spacetime, due to the two inequivalent chiral spin representations. Hence it is natural to combine both, and form the Cartesian fiber product of 2+1-dimensional super-Minkowski spacetime with itself, over ordinary 3d spacetime, and regarded as a product of Spin(2,1)-representations for the two different choices.
The result of that fibered Cartesian product construction is, unsurprisingly now, type N=(1,1) d=2+1 super Minowski spacetime. And this happens to carry yet one more interesting 2-cocycle, one that combines the fermionic variables of different chirality. Hence we get another extension by another copy of the real line. What is that extension? This is N=1 d=3+1 super-Minkowski spacetime, the geometry on which 4d minimal supergravity is modeled.
This might be a place to stop and explore. But let’s keep going to see where the homotopy Lie theory takes us.
On this N=1 4d super-Minkowski spacetime there is no interesting 3-cocycle, so the next step up in the “Whitehead tower”, nothing happens in this case. Then next we are to look out for a 4-cocycle. Such does happen to exist. It is known as the curvature of the WZW term for the Green-Schwarz super 2-brane in 4d.
These “non-critical” Green-Schwarz super p-branes in dimensions 3 and 4 have been known since long ago, but are still little explored. For some references suggesting that they deserve more attention than one might think, see the references here and here. (They are shadows of the G2-compactification of M-theory from 11d down to 4d that we are going to find below.)
So we keep going. Next, we form q-fold Cartesian products of the superpoint with itself for various q, and extend by exceptional cocycles existing on these. Something special happens next for q = 16. The 16-fold superpoint carries a space spanned by 10 2-cocycles. Extending by these make N=1 d=9+1 super-Minkowski spacetime appear. Just as N=1 d=4 super-Minkowski, this happens to carry an exceptional 3-cocycle. That 3-cocycle is known in the literature as the curvature of the WZW term of the heterotic Green-Schwarz superstring.
And as before in 4d, one finds that the joint stabilizer group of the supersymmetry and of that 3-cocycle in 10d is the Spin-cover of the Lorentz group in that dimension, Spin(9,1). Moreover, it again acts in two inequivalent ways, and so a fibered Cartesian product makes N=1 d=9+1 super-spacetime become N=(1,1) and N=(2,0). On these, we hunt again for 3-cocycles, and indeed we find them: one corresponding to the type IIA superstring, and one corresponding to the type IIB superstring.
Now on N=(1,1) d=9+1 superspacetime there also appears again one special new 2-cocycle. This corresponds to the D-0-brane. Extending by this (making the D0-branes condense) yields hence another extension of 10d spacetime by one more real dimension: we get the N=1 d=10+1 super-Minkowski spacetime on which 11-dimensional supergravity is modeled.
One finds that this 11-dimensional spacetime does not carry an interesting 3-cocycle, so here the next step in the tower doesn’t do anything and no string appears in 11d. But then one finds… an exceptional 4-cocycle, just as there was in 4d. This is known as the curvature of the WZW term of the Green-Schwarz M2-brane propagating in 11-dimensional spacetime.
Back in the old days, the collection of these statements, so far, was the content of the old brane scan, which may be depicted like so (taken from Duff 87):
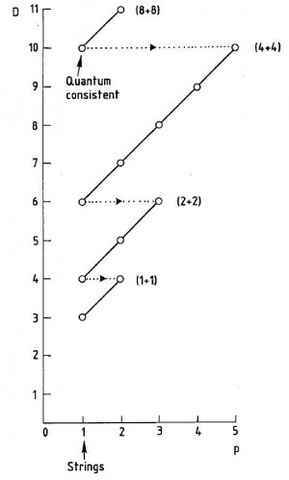
This old brane scan did indeed end at this stage. It classified the possible super-string and super-membranes in various dimensions, as well as for instance the NS-5-brane, by super Lie algebra cohomology, but it did not see the D-branes, and not the M5-brane: there are no cocycles corresponding to the D-branes on 10-dimensional super-Minkowski spacetime, and no cocycle corresponding to the M5-brane on 11-dimensional super-Minkowski spacetime.
Here the super-L-infinity theory comes to the rescue. By that story of the Whitehead tower, we know that before looking for further cocycles, we are first to form the extension classified by the previous cocycles. Now for the 3- and 4-cocycles in 10d and 11d, these extensions do not exist in the realm of super-Lie algebras anymore. Instead, these extensions are super Lie 2-algebras and super Lie-3 algebras, respectively: the superstring Lie 2-algebra and the M2-brane super Lie 3-algebra, as discussed above. In order to proceed with the brane scan, one has to climb from Lie theory to homotopy Lie theory / L-infinity theory / Lie n-theory. Whichever name you prefer.
And indeed, on the super-membrane Lie 3-algebra one does find that there is more, there is an interesting… 7-cocycle. Looking back,it is remarkable that this 7-cocycle was also found, also in slight disguise, already back in that remarkable article (D’Auria-Fre 82). Before its time had come. That 7-cocycle was much later understood, in (BLNPST 97), to indeed be the curvature of the WZW term for the Green-Schwarz-type sigma model of the M5-brane in 11d.
And similarly, one finds cocycles in all degrees p+2, for all the D-p-branes of type II string theory, not on super-Minkowski spacetime, but on its extension by the type II superstring super Lie 2-algebras.
Drawing out the sequence of iterative extensions that I am talking about here, one finds a tree — or bouquet — of extensions that turns out to reflect the full spacetime and p-brane contents of string/M-theory. It contains in it the old brane scan, as the level-1 branches after bare spacetime itself, but then it expands to include branes with higher gauge fields (vector and tensor-multiplet fields) on their worldvolume at the second level, to form a bouquet of branes.
We may naturally display this as follows. Since any extension maps down to what it is extending, there is an arrow to be drawn to a superpoint from the super-Minkowski spacetime that extends it; then an arrow to that spacetime from any super Lie (p+1)-algebra named after a super p-brane propagating on that spacetimeM and finally an arrow to that super Lie (p+1)-algebra from any super Lie (p2+1)-algebra named after the super p2-brane on which the given super p-brane may end.
Proceeding this way, the part of the brane bouquet that branches into 10d and 11d spacetimes look as follows:
This, and many other aspects mentioned here, is discussed in some detail in
- Domenico Fiorenza, Hisham Sati, Urs Schreiber,
Super Lie n-algebra extensions, higher WZW models, and super p-branes with tensor multiplet fields
International Journal of Geometric Methods in Modern Physics, Volume 12, Issue 02 (2015)
(arXiv:1308.5264, DOI: 10.1142/S0219887815500188, nLab)
That article also contains a comprehensive collection of pointers to the literature discussing which piece of the above bouquet was found where.
On a speculative note, observe that the “branching level” 0, 1, and 2 at which the p-branes appear in the brane bouqet as iterative L-infinity extensions match the level number at which their charges appear in the fundamental representation of E11. Maybe that’s a coincidence. Or maybe there is more to it. Such aspects of U-duality I will talk about more in another article.
But the iterative mathematical pattern here neatly and clearly matches a key aspect of the physics: those p-branes on which no other p-branes end and which have no gauge fields on their worldvolumes (the string and the membrane) come from cocycles on spacetime itself. But those branes on which other branes may end and which carry (higher) gauge fields on their worldvolume — the D-branes and the M5 — these come from “second-order” cocycles on those super Lie (p+1)-algebra extensions of super-Minkowski spacetime that were classified previously by the (p+2)-cocycles of the p-branes that may end on them.
This is not a coincidence. Indeed, this is an incarnation of the fact that in higher geometry the concepts of sigma-model and of gauge theory unify: a gauge theory is equivalently a sigma-model whose target space is not just a manifold, but a suitable smooth stack. And a higher gauge theory (for higher form fields) is a sigma model with a target of a suitable higher smooth stack. But just as Lie algebras are nothing but the infinitesimal version of Lie groups, so homotopy Lie n-algebras are the infinitesimal incarnation of higher smooth stacks.
The implication of this is that a Lie n-algebra extension of spacetime may itself be regarded as the infinitesimal version of a higher stacky spacetime which mixes aspects of sigma-models and of gauge theories: a map from a worldvolume into such a stacky extension of spacetime is equivalently a pair of two fields: first an ordinary sigma-model field for the ordinary underlying spacetime, and second a higher gauge field which is twisted in some way by the first map. (This is the content of the last section of the above article. Lecture notes that expand on this point are here.)
This is a phenomenon that keeps string theorists busy these days. And it all originates in the classification of super-Lie n-algebras. It all grows out of the single seed that is the superpoint, planted into the substance of geometric homotopy theory.
I am a researcher in the department Algebra, Geometry and Mathematical Physics of the Institute of Mathematics at the Czech Academy of the Sciences (CAS) in Prague.
Presently I am on leave at the Max Planck Institute for Mathematics in Bonn.


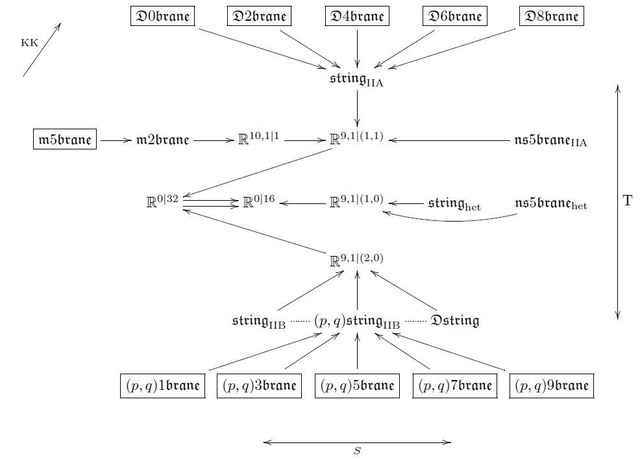






We now finally have an article on the topic of "Emergence from the superpoint" on the arXiv:John Huerta and Urs Schreiber:"M-Theory from the superpoint"arxiv.org/abs/1702.01774ncatlab.org/schreiber/show/M-Theory+from+the+SuperpointAbstract: The "brane scan" classifies consistent Green–Schwarz strings and membranes in terms of the invariant cocycles on super-Minkowski spacetimes. The "brane bouquet" generalizes this by consecutively forming the invariant higher central extensions induced by these cocycles, which yields the complete brane content of string/M-theory, including the D-branes and the M5-brane, as well as the various duality relations between these. This raises the question whether the super-Minkowski spacetimes themselves arise as maximal invariant central extensions. Here we prove that they do. Starting from the simplest possible super-Minkowski spacetime, the superpoint, which has no Lorentz structure and no spinorial structure, we give a systematic process of consecutive maximal invariant central extensions, and show that it discovers the super-Minkowski spacetimes that contain superstrings, culminating in the 10- and 11-dimensional super-Minkowski spacetimes of string/M-theory and leading directly to the brane bouquet.
Regarding higher spin gauge theory, what I find curious is that this connects to another old appearance of L-infinity algebras in string theory that sometimes seems not to get due attention. Namely first of all, higher spin gauge theory is expected to be the tensionless limit of bosonic string field theory (http://ncatlab.org/nlab/show/higher+spin+gauge+theory#ReferencesRelationToStringTheory). But, second, Zwiebach's seminal work from the 1990 (http://ncatlab.org/nlab/show/string+field+theory#ReferencesHomotopyAlgebra) shows that the genus-0 n-point functions of closed bosonic strings form an L-infinity algebra, even before passing to the tensionless limit. (Hence as the tension does go to zero one should expect, up to some technical subtleties, a kind of contraction limit that takes this tensionful string field L-infinity algebra to a higher spin gauge theory.)
Right, the bouquet might not end there. I don't presently know if and how it continues. But that's related to the speculative remark I made above, observing that for low values the branching level of the brane bouquet matches the level decomposition of the fundamental representation of E11 (http://ncatlab.org/nlab/show/E11#FundamentalRepresentationAndBraneCharges): for level 0 (spacetime), level 1 (M2-branes) and 2 (M5-branes). Should that be more than a coincidence, then it might point to the existence of a further 10-cocycle on the M5-brane super Lie 6-algebra corresponding to the "dual graviton" since that is what sits at level 3 in the E11 story.
In what sense does the brane bouquet "end" at the point where story ends? Does it end because people haven't explored it further? If so, exploring it further might reveal further treasures! Or does it end for some clear mathematical reason? I often hear people speak of 11-dimensional supergravity as the highest-dimensional supergravity theory that doesn't contain fields of spin > 2. But I've never been very happy with that, since the rationale seems to be that fields of spin > 2 aren't renormalizable… yet nobody has fully settled the issues of renormalizability for various supergravity theories: people seem to keep discovering unexpected cancellations. If the "ending" of the brane bouquet is defined by the requirement of no spins > 2, it would be at least mathematically interesting to drop that requirement and see what comes next – maybe some hidden treasure? On a vaguely related note, I hear some physicists are getting interested in higher-spin fields; in fact there will be a workshop on them in Singapore:http://www.ntu.edu.sg/ias/upcomingevents/HSGT/But I don't know anything about this.
Thanks! Fixed now.
Very nice article. Here are some typos, in case you want to publish it in a book someday:" It is known as the curvature of the WZW for the Green-Schwarz super 2-brane in 4d." – it seems there should be some noun after "WZW"."On a speculative note, observe that the “branching level” 0, 1, and 2 at which the p-branes appear in the brane bouqte" – should be "bouquet"."already back in that remarkable artice" – should be "article".