Examples of Prequantum Field Theories III: Chern-Simons-type Theories

 

 

After having constructed gauge fields and higher gauge fields in the previous article by systems of $L_\infty$-algebroid-valued differential forms on simplex bundles, we now use the same method to construct prequantum field theories — in the sense discussed at Higher Prequantum Geometry I, II, III, IV, V — of higher Chern-Simons type with such higher gauge fields.

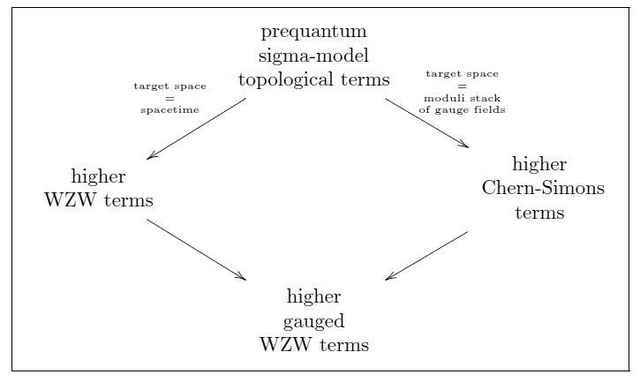
We begin with a few general words on how to obtain prequantum field theories of sigma-model type from “higher topological terms”. Chern-Simons-type prequantum field theory is one example of this, the Wess-Zumino-Witten type field theories that concern us in the next article in the series are another.

The impatient reader might want to first skip to the last section of this page here, which lists some example systems that we obtain, and then come back to read through how they are constructed.

---

 

A sigma-model is a field theory, whose field bundle (as in Higher Prequantum Geometry II) is of the simple form
$$
\Sigma \times X \stackrel{p_1}{\longrightarrow} \Sigma
$$
for some space $X$. This means that in this case field configurations, which by definition are sections of the field bundle, are equivalently maps of the form
$$
\phi : \Sigma \longrightarrow X
\,.
$$
One naturally thinks of such a map as being a $\Sigma$-shaped trajectory of a $p$-dimensional object (a $p$-brane) on the space $X$. Hence $X$ is called the target space of the model. Specifically, if this models
$\Sigma$-shaped trajectories of $p$-dimensional relativistic branes, then $X$ is the target spacetime. There are also famous examples of sigma-models where $X$ is a more abstract space, usually some moduli space of certain scalar fields of a field theory that is itself defined on spacetime. Historically the first sigma-models were of this kind. In fact, in the first examples $X$ was a linear space. For emphasis that this is not assumed one sometimes speaks of non-linear sigma models for the sigma-models that we consider here. In fact, we consider examples where $X$ is not even a manifold, but a smooth infinity-groupoid, a higher moduli stack.

Given a target space $X$, then every $(p+1)$-form $A_{p+1} \in \Omega^{p+1}(X)$ on $X$ induces a local Lagrangian for sigma-model field theories with target $X$: we may simply pull back that form to the jet bundle $J^\infty_\Sigma(\Sigma \times X)$ and project out its horizontal component. Lagrangians that arise this way are known as topological terms.

The archetypical example of a sigma-model with topological term is that for describing the electron propagating in a spacetime and subject to the background forces of gravity and of electromagnetism. In this case $p = 0$ (for a point particle, hence a “”0-brane””), $\Sigma$ is the interval $[0,1]$ or the circle $S^1$, regarded as the abstract “worldline” of an electron. Target space $X$ is a spacetime manifold equipped with a pseudo-Riemannian metric $g$ (modelling the background field of gravity) and with a vector potential 1-form $A\in \Omega^1(X)$ whose differential is the Faraday tensor $F = d A$ (modelling the electromagnetic background field). The local Lagrangian is
$$
L = L_{\mathrm{kin}} + \underset{L_{\mathrm{int}}}{\underbrace{q (A_\Sigma)_H}} \;\; \in \Omega^{p+1}_H(J^\infty_\Sigma(\Sigma \times X))
\,,
$$
where $L_{\mathrm{kin}}$ is the standard kinetic Lagrangian for (relativistic) point particles, $q$ is some constant, the electric charge of the electron, and $(A_\Sigma)_H$ is the horizontal component of the pullback of $A$ to the jet bundle. The variation of $L_{\mathrm{int}}$ yields the Lorentz force that the charged electron experiences.

Now, as in the discussion in Higher Prequantum Geometry I, in general, the Faraday tensor $F$ is not globally exact, and hence in general there does not exist a globally defined such 1-form on the jet bundle. But via the sigma-model construction, the prequantization of the worldline field theory of the electron on its jet bundle is naturally induced by a Dirac charge quantization of its background electromagnetic field on target spacetime: given

a circle-principal connection on target spacetime for the given field strength Faraday tensor $F$  (hence with local “vector potential” 1-forms $\{A_i\}$ with respect to some cover $\{U_i \to X\}$), then the horizontal projection $(\nabla_\Sigma)_H$ of the pullback of the whole circle-bundle with connection to the jet bundle constitutes a prequantum field theory in the sense of Higher Prequantum Field Theory III . Similarly, the background electromagnetic field $\nabla$ also serves to prequantize the covariant phase space of the electron, according to Higher Prequantum Field Theory IV. This is related to the familiar statement that in the presence of a magnetic background field the spatial coordinates of the electron no longer  Poisson-commute with each other.
This prequantization of sigma-models via $(p+1)$-form connections on target space works generally:  we obtain examples of prequantum field theories of sigma-model type by adding to a globally defined kinetic Lagrangian form a prequantum topological term given by the pullback of a $(p+1)$-form connection on target space. The pullback of that target  $(p+1)$-form connection to target space serves to prequantize the entire field theory in all codimensions:

While sigma-models with topological terms are just a special class among all variational field theories, in the context of higher differential geometry this class is considerably larger than in traditional differential geometry. Namely, we may regard any of the moduli stacks $\mathbf{A}_{\mathrm{conn}}$ of gauge fields that we discuss in Examples for Prequantum Field Theories I and Examples for Prequantum Field Theories II as target space, i.e. we may consider higher stacky field bundles
of the form
$$
\Sigma \times\mathbf{A}_{\mathrm{conn}}
\stackrel{p_1}{\longrightarrow}
\Sigma
\,.
$$
Everything goes through as before, in particular a field configuration now is a map $\Sigma \longrightarrow \mathbf{A}_{\mathrm{conn}}$ from worldvolume/spacetime $\Sigma$ to this moduli stack. But by the nature of $\mathbf{A}_{\mathrm{conn}}$, such maps now  are equivalent to gauge fields on $\Sigma$. These are, of course, the field configurations of gauge theories. Hence, in higher differential geometry, the concepts of sigma-model field theories and of gauge field theories are unified.

In particular, both concepts may mix. Indeed, we find below that higher-dimensional Wess-Zumino-Witten-type models generally are “higher gauged”, this means that their field configurations are a pair consisting of a map $\phi : \Sigma \to X$ to some target spacetime $X$, together with a $\phi$-twisted higher gauge field on $\Sigma$.

Examples of a (higher) gauged WZW-type sigma model are the Green-Schwarz-type sigma-models of those super $p$-branes on which other branes may end. This includes the D-branes and the M5-brane. The former are gauged by a 1-form gauge field (the “Chan-Paton gauge field”) while the latter is gauged by a 2-form gauge field. We say more about these examples in the next article.

---

 

We may construct examples of prequantized topological terms from functionality of the Lie integration process that already gave the (higher) gauge fields themselves in Examples of Prequantum Field Theories II. There we saw that a $(p+2)$-cocycle on  an $L_\infty$-algebroid is a homomorphism of $L_\infty$-algebroids of the form
$$
\mu : \mathfrak{a} \longrightarrow \mathbf{B}^{p+2}\mathbb{R}
\,.
$$
Moreover, the $\exp(-)$-construction which sends $L_\infty$-algebroids to simplicial presheaves representing universal higher moduli stacks of $\mathfrak{a}$-valued gauge fields is clearly functorial,  hence it sends this cocycle to a morphism of simplicial presheaves of the form
$$
\exp(\mu) : \exp(\mathfrak{a}) \longrightarrow \mathbf{B}^{p+2}\mathbb{R}
\,.
$$
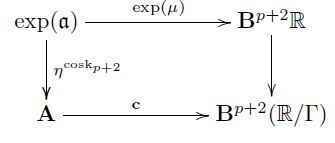
One finds that this descends to the $(p+2)$-coskeleton $\mathbf{A} := \mathrm{cosk}_{p+2}\exp(\mathfrak{a})$  after quotienting out the subgroup $\Gamma \hookrightarrow \mathbb{R}$ of periods of $\mu$ (just as in the prequantization of the global action functional in Higher Prequantum Geometry III):

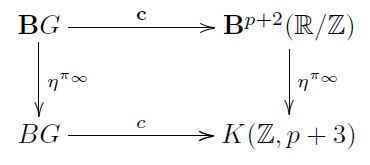
To get a feeling for what the resulting morphism $\mathbf{c}$ is, consider the case that $\mathbf{A} = \mathbf{B}G$ for some group $G$. There is a geometric realization operation $\pi_\infty$ which sends smooth infinity-groupoids to plain homotopy types (homotopy types of topological spaces). Under this operation a map $\mathbf{c}$ as above becomes a map $c$ of the form

where $B G$ is the traditional classifying space of a (simplicial) topological group $G$, and where $K(\mathbb{Z},p+3) = B^{p+3}\mathbb{Z}$ is the Eilenberg-MacLane space that classifies integral cohomology in degree $(p+3)$. What $B G$ classifies are $G$-principal bundles, and hence for each space $\Sigma$ the map $c$ turns into a characteristic class of equivalence classes of $G$-principal bundles:
$$
c_\Sigma : G \mathrm{Bund}(\Sigma)_\sim \longrightarrow H^{p+3}(\Sigma,\mathbb{Z})
\,.
$$
Hence $c$ itself is a universal characteristic class. Accordingly, $\mathbf{c}$ is a refinement of $c$ that knows about gauge transformations: it sends smooth $G$-bundles with smooth gauge transformations and gauge-of-gauge transformations between these to integral cocycles and coboundaries and coboundaries-between-coboundaries between these.

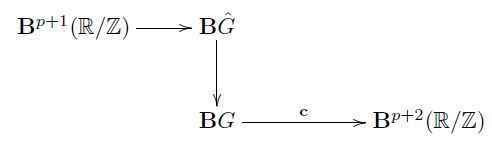
Equivalently, we may think of $\mathbf{c}$ as classifying a $(p+1)$-gerbe on the universal moduli stack of $G$-principal bundles. This is equivalently its homotopy fiber (in direct analogy with the infinitesimal  version of this statement Examples for Prequantum Field Theories II) fitting into a long homotopy fiber sequence of the form

Yet another equivalent perspective is that this defines an infinity-group extension $\hat G$ of the infinity-group $G$ by the infinity-group $\mathbf{B}^p (\mathbb{R}/\mathbb{Z})$.

So far all this is without connection data, so far these are just higher instanton sectors without any actual gauge fields inhabiting these instanton sectors. We now add connection data to the situation.

---

For $\mathfrak{g}$ a semisimple Lie algebra with Killing form invariant polynomial $\langle -,-\rangle$,  classical 3-dimensional Chern-Simons theory  has as fields the space of $\mathfrak{g}$-valued differential 1-forms $A$, and the Lagrangian is the Chern-Simons 3-form
$$
L_{\mathrm{CS}}(A) = \mathrm{CS}(A) := \langle A \wedge d A \rangle – \tfrac{1}{3} \langle A \wedge [A\wedge A]\rangle
\,.
$$
This Chern-Simons form is characterized by two properties: for vanishing curvature it reduces to the value of the 3-cocycle
$\langle -,[-,-]\rangle$ on the connection 1-form $A$, and its differential is the value of the invariant polynomial $\langle -,-\rangle$ on  the curvature 2-form $F_A$.

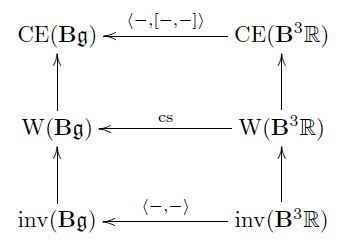
There is a slick way to express this in terms of the dg-algebraic description from Examples for Prequantum Field Theories II: there is an element $\mathrm{cs} \in \mathrm{W}(\mathbf{B}\mathfrak{g})$, which in terms of the chosen basis $\{t^a\}$ for $\wedge^1\mathfrak{g}^\ast$ is given by
$$
\mathrm{cs} : k_{a b} (d_W t^a)\wedge t^b – \tfrac{1}{3} k_{a a’} C^{a’}{}_{b c} t^a \wedge t^b \wedge t^c
\,.
$$
Hence equivalently this is a dg-homomorphism of the form
$$
\mathrm{W}(\mathbf{B}\mathfrak{g})
\stackrel{\mathrm{cs}}{\longleftarrow}
\mathrm{W}(\mathbf{B}^3\mathbb{R})
$$
and for $A \in \Omega^1(\Sigma,\mathfrak{g}) = \{ \; \Omega^\bullet(\Sigma) \longleftarrow \mathrm{W}(\mathbf{B}\mathfrak{g}) \; \}$ then the Chern-Simons form of $A$ is the composite
$$
\Omega^\bullet(\Sigma)
\stackrel{A}{\longleftarrow}
\mathrm{W}(\mathbf{B}\mathfrak{g})
\stackrel{\mathrm{cs}_3}{\longleftarrow}
:
\mathrm{CS}(A)
\,.
$$
Now, the two characterizing properties satisfied by the Chern-Simons equivalently mean in terms
of dg-algebra that the map $\mathrm{cs}$ makes the following two squares commute:

This shows how to prequantize 3d Chern-Simons theory in codimension 3: the vertical sequences appearing here are just the Lie algebraic data to which we apply differential Lie integration, as in Examples of Prequantum Field Theories II, to obtain the moduli stacks of $G$-connections and of 3-form connections, respectively. Moreover,  by the discussion above and using that $\langle -,[-,-]\rangle$ represents an integral cohomology class on $G$ we get a map
$$
(\mathbf{c}_2)_{\mathrm{conn}}
:=
\exp(\mathrm{cs})
\;:\;
\mathbf{B}G_{\mathrm{conn}}
\longrightarrow
\mathbf{B}^3(\mathbb{R}/\mathbb{Z})_{\mathrm{conn}}
\,.
$$
This is the background 3-connection which induces prequantum Chern-Simons field theory by the general procedure indicated above.

Notice that this map is a refinement of the traditional Chern-Weil homomorphism. This allows for instance to prequantize the Green-Schwarz anomaly cancellation condition  heterotic strings: the higher moduli stack of GS-anomaly free gauge fields is the homotopy fiber product of the prequantum Chern-Simons Lagrangians for the simple groups $\mathrm{Spin}$
and $\mathrm{SU}$ [Sati-Schreiber-Stasheff 12].

 

---

 

This higher Lie theoretic formulation of prequantum 3-Chern-Simons theory now immediately generalizes to produce higher (and lower) dimensional prequantum $L_\infty$-algebroid Chern-Simons theories.

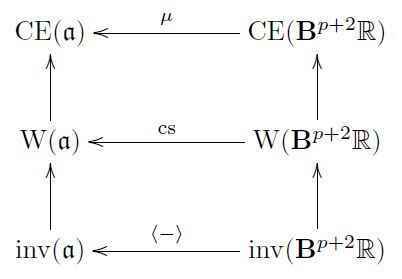
For $\mathfrak{a}$ any $L_\infty$-algebroid, we say a $(p+2)$-cocycle $\mu$ on $\mathfrak{a}$ is in transgression with an invariant polynomial $\langle -\rangle$ on $\mathfrak{a}$ if there is an element $\mathrm{cs} \in W(\mathfrak{a})$ (a Chern-Simons element) such that $d_W \mathrm{cs} = \langle -\rangle$ and such that $\mathrm{cs}|_{\mathrm{CE}} = \mu$. Equivalently this means that $\mathrm{cs}$ fits into a diagram  of dg-algebras of the form:

Applying $\exp(-)$ to this, this induces maps of smooth moduli stacks of the form
$$
\mathbf{c}_{\mathrm{conn}} : \mathbf{A}_{\mathrm{conn}} \longrightarrow \mathbf{B}^{p+2}(\mathbb{R}/\Gamma)_{\mathrm{conn}}
\,.
$$
This gives a prequantum Chern-Simons-type field theory whose field configurations locally are  $\mathfrak{a}$-valued differential forms, and whose Lagrangian is locally the Chern-Simons element $\mathrm{cs}$ evaluated on these forms.

---

 

For instance if $(\mathfrak{a},\omega)$ is a symplectic Lie p-algebroid, then we obtain the prequantization of $(p+1)$-dimensional AKSZ-type field theories [Fiorenza-Rogers-Schreiber 11]. For $p = 1$ this  subsumes the topological string A- and B-model [Alexandrov-Kontsevich-Scharz-Zaboronsky 95]. Generally, the prequantum moduli stack of fields for 2-dimensional prequantum AKSZ theory is a differential refinement of the symplectic groupoid of a given Poisson manifold [Bongers 14].  The Poisson manifold canonically defines a boundary condition for the corresponding prequantum 2d Poisson-Chern-Simons theory, and the higher geometric boundary quantization of this 2d prequantum theory reproduces ordinary Kostant-Souriau geometric quantization of the symplectic leafs [Nuiten 13]. This is a non-perturbative improvement of the perturbative algebraic deformation quantization of the Poisson manifold as the boundary of the perturbative 2d AKSZ field theory due to [Cattaneo-Felder 99].

Generally one expects to find non-topological non-perturbative $p$-dimensional quantum field theories arising this way as the higher geometric boundary quantization of  $(p+1)$-dimensional prequantum Chern-Simons type field theories.

For instance for $(\mathbf{B}^3 \mathbb{R}, \omega)$ the line Lie 3-algebra equipped with its canonical binary invariant polynomial, the corresponding prequantum Chern-Simons type field theory is 7-dimensional abelian cup-product Chern-Simons theory [Fiorenza-Sati-Schreiber 12]. This has been argued to induce on its boundary the conformal 6-dimensional field theory of a self-dual 2-form field [Witten 96, Hopkins-Singer 02]. This 7-dimensional Chern-Simons theory is one summand in the Chern-Simons term of 11-dimensional supergravity compactified on a 4-sphere. The AdS7/CFT6 correspondence predicts that this carries on its boundary the refinement of the self-dual 2-form to a 6-dimensional superconformal field theory. There are also nonabelian summands in this 7d Chern-Simons term. For instance for $(\mathbf{B}\mathfrak{string}_{\mathfrak{g}},\langle -,-,-,-\rangle)$ the string Lie 2-algebra equipped with its canonical degree-4 invariant polynomial, then the resulting prequantum field theory is 7-dimensional Chern-Simons field theory on String 2-connection fields [Fiorenza-Sati-Schreiber 12].

For more exposition of prequantum Chern-Simons-type field theories see also [Fiorenza-Sati-Schreiber 13].