A Geometrical View of Time Dilation and the Twin Paradox

Based on the number of questions we receive on the topic at Physics Forums, there is a lot of confusion in the general public about how time dilation works and the resolution of the twin paradox. More often than not, the confusion is based upon misunderstandings about how time dilation works and an unawareness of the relativity of simultaneity and how time is treated within special relativity. The aim of this Insight is to make an attempt at explaining these issues from a geometrical viewpoint and to see that we have an analogous effect in the geometry which we are used to, although we do not find it strange.

A geometrical analogue

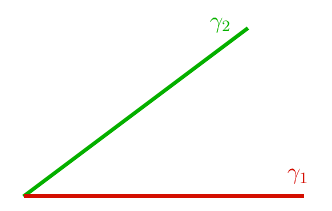
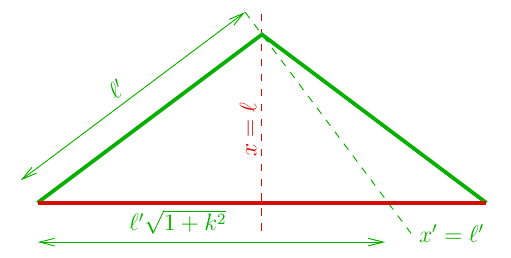
Consider the following two lines in normal two-dimensional space:

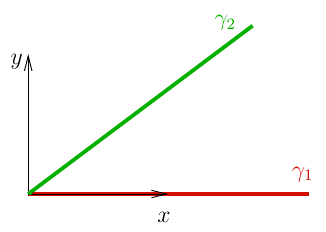
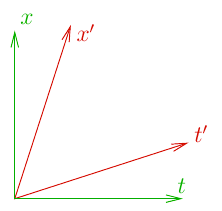
In order to describe the lines, we can introduce a coordinate system $S$:

In this coordinate system, we may use the coordinate $x$ to parametrise the lines, we find that

$$y_1(x) = 0, \quad y_2(x) = kx,$$

where $k$ is some constant. The lengths of each line between $x = 0$ and $x = x_0$ are simply given by Pythagoras’ theorem $\ell^2 = \Delta x^2 + \Delta y^2$ and we find

$$\ell_1 = x_0, \quad \ell_2 = x_0\sqrt{1+k^2}.$$

Consequently we find that the ratio between these two lengths is $\ell_2/\ell_1 = \sqrt{1+k^2}$. This is shown in the following figure:

However, there is nothing special about the coordinate system $S$. In particular, the length of the lines between any two given points on the lines does not depend on the coordinate system chosen and we could just as well have chosen to introduce the coordinate system $S’$ according to:

In this new coordinate system, we can parametrise the lines using the $x’$ coordinate

$$y_1′(x’) = -kx’, \quad y_2′(x’) = 0.$$

For the distance between the points corresponding to $x’ = 0$ and $x’=x_0’$, Pythagoras’ theorem now results in

$$\ell_1′ = x_0′ \sqrt{1+k^2}, \quad \ell_2′ = x_0’$$

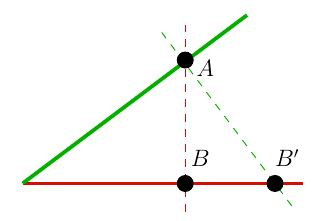
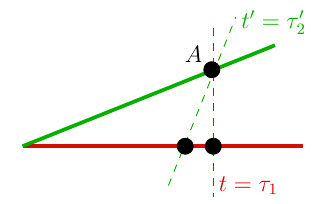
and so the line $\gamma_1$ is longer than $\gamma_2$ for the same difference in the parameter $x_0’$, unlike in the case when the lines were parametrised by $x$, where we obtained the opposite result. Yet there is nothing strange going on here. If we select a point on $\gamma_2$ and draw the lines which have the same $x$ and $x’$ values as that point, it becomes clear that the points on $\gamma_1$ which share the same parameter value differs depending on whether we used $x$ or $x’$ for the parametrisation. We should therefore not be surprised to find that the length to these two different points is different. Let us call this effect the “relativity of the same $x$-coordinate”. Naturally, this effect does not affect the actual length of any of the lines, but only what lengths are being compared. This is illustrated in the following figure:

The points $B$ and $B’$ have the same $x$ and $x’$ coordinate as $A$, respectively, yet correspond to different points on the curve $\gamma_1$ and therefore the distance from the intersection along this curve is different.

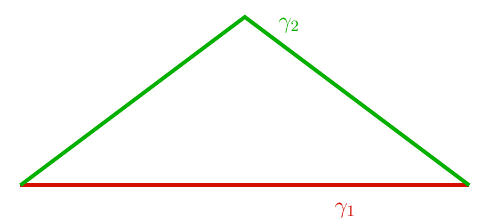
Curves intersecting at two points

Now consider the following two curves:

Just from the figure, it should be clear that $\gamma_2$ is longer and we can verify this by introducing the coordinate system $S$ as above and computing the lengths of each of the curves, with the length of $\gamma_2$ being split into two equal contributions, one from each line segment.

We find that

$$\ell_1 = 2\ell, \quad \ell_2 = 2\ell \sqrt{1+k^2}.$$

We could also compute the length of the curves by looking at the $S’$ coordinate system:

By our previous discussion, the length of $\gamma_2$ up to the half-way point is given by

$$\ell_2’/2 = \ell’,$$

while the length of $\gamma_1$ to the same $x’$ coordinate is given by

$$\ell_1’/2 = \ell’ \sqrt{1+k^2}.$$

If we were not careful about the relativity of the same $x$-coordinate, we might do the error of thinking that $\ell_1’/2$ would be half the length of the curve $\gamma_1$. However, since we are aware of this, we know better than that and realise that if we multiplied the result by two, we would obtain a result that was too large.

Application to relativity

So how does this connect to relativity and time dilation? In its foundation, relativity is a theory about the geometry of space-time, which may be described using a coordinate system with four coordinates $(t,x,y,z)$, where the first coordinate is the time coordinate and the last three are spatial coordinates. It is important to realise that, just as in the case we just described, these are just coordinated that, by themselves, do not have a direct physical interpretation. For the sake of brevity, let us consider only one spatial coordinate $x$ as the generalisation is straightforward.

In relativistic space-time, geometry works a bit differently as compared to the geometry with which we are used to working with. In particular, this manifests itself by a change in Pythagoras’ theorem, which now comes with a minus sign

$$c^2 \tau^2 = c^2 \Delta t^2 – \Delta x^2$$

as a direct consequence of the speed of light is equal in all frames. Here, $\tau$ is the proper time along a straight line in space-time with $\Delta t$ being the difference between the endpoint time-coordinates and $\Delta x$ being the difference between the endpoint space-coordinates. This also changes what kind of coordinate transformations we can do in order for the proper time to be the same regardless of the coordinates. Instead of rotating both coordinate axes in the same direction as happens for rotations, the coordinate axes are rotated by the same angle in opposite directions.

In relativity, the proper time defined above is the only time that will be measured by any observer. A curve in space-time is called a world line and will generally describe the position of the observer as a function of time. The proper time of a world line, i.e., the “length” of the world line with the modified Pythagorean theorem, is the time a clock following that world line will measure, the time coordinate $t$ has no particular meaning other than that it happens to numerically be the same as the time measured by an observer for which the spatial coordinates are not changing. This is completely analogous with our previous example, where the value of the $x$-coordinate was equal to the length of a line for which the $y$-coordinate was not changing.

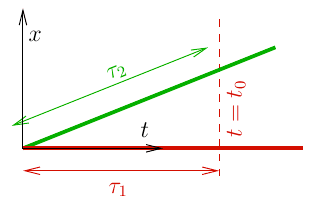
We can now do the exact same exercise as we did use regular geometry. Consider the following two world lines described in the coordinate system $S$:

The first world line $\gamma_1$ has a constant $x$-component while the second world line describes an observer travelling with velocity $v$. The world lines may be parametrised as

$$x_1(t) = 0, \quad x_2(t) = vt.$$

We can now compute the proper time from the time coordinate taking values $t=0$ to $t=t_0$ for both world lines using the modified Pythagorean theorem and find that

$$\tau_1 = t_0, \quad \tau_2 = t_0\sqrt{1-\frac{v^2}{c^2}}$$

or, equivalently,

$$\frac{\tau_1}{\tau_2} = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}} \equiv \gamma,$$

where $\gamma$ is the famous gamma-factor of special relativity. In other words, the proper time elapsed for an observer at rest between two time coordinates will differ from the proper time elapsed for a moving observer between the same time coordinates by a factor $\gamma$. This is the essence of time-dilation but, just as for the example we started with, it crucially depends on the coordinate system.

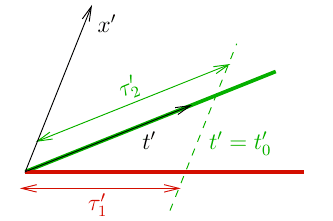
Taking a different coordinate system $S’$ where $\gamma_2$ describes a world line with constant $x’$

the exact same argumentation will now lead us to conclude

$$\frac{\tau_1′}{\tau_2′} = \sqrt{1-\frac{v^2}{c^2}} = \frac{1}{\gamma}.$$

This is the exact opposite relation as that we found in the coordinate system $S$, just as was the case in our first example! The resolution of this apparent mismatch is also completely analogous. We should not be surprised to find a different relation between the times elapsed between different $t$ coordinates and that elapsed between different $t’$ coordinates simply because the point on the curve $\gamma_1$ with the same $t$ coordinate as the point $A$ on $\gamma_2$ will not be the same as the point on $\gamma_1$ with the same $t’$ coordinate as $A$:

This effect is called the “relativity of simultaneity” and simply states that two events which are simultaneous in one set of coordinates are generally not simultaneous in a different set of coordinates (simultaneous simply refers to having the same time-coordinate). As such, there is really nothing strange about the proper times to $B$ and $C$ along with $\gamma_1$ being different since they are simply not the same point.

The twin paradox

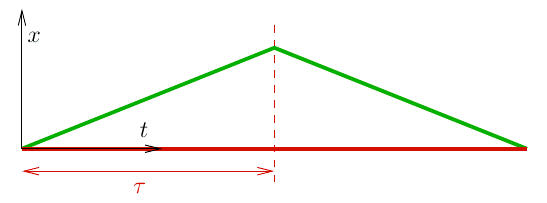
The twin paradox is the analogue of our second example with two curves starting and ending at the same points. In the case of two twins, where one leaves to travel to a faraway land and later returns, the world lines in the coordinate system $S$ would look like:

Just applying the modified Pythagorean theorem it becomes clear that the proper times along the world lines are given by

$$\tau_1 = 2\tau, \quad \tau_2 = 2\tau \sqrt{1-\frac{v^2}{c^2}} \quad \Longrightarrow \quad \frac{\tau_1}{\tau_2} = \gamma$$

and therefore more proper time will pass for the twin staying behind, i.e., the red curve. Going to the coordinate system $S’$, we can compute the relation between the proper time $\tau’$ taken for the travelling twin to reach the turnaround point and the proper time elapsed for the staying twin during the same $t’$ interval as

$$\frac{\tau_1′}2 = \tau’ \sqrt{1-\frac{v^2}{c^2}}, \quad \frac{\tau_2′}2 = \tau’.$$

However, just as in our example above, the relativity of simultaneity tells us that $\tau_1’$ is not equal to half the proper time elapsed for the staying twin until the reunion:

Instead, the change of geometry indicates that we are not counting part of the world line if we make this assumption and therefore underestimate the elapsed proper time. If taken properly into account, we would find that the time elapsed for the staying twin is longer also as computed by the travelling observer. This resolves the twin paradox and shows that it only appears due to not taking the relativity of simultaneity properly into account.

Click for Forum Comments