Geometry of Side-side-angle (SSA) and Impossible Triangles

What is the ambiguous case?

In high school geometry, the idea of proofs is often first introduced to American students. A common task is to use a basic set of rules to prove two triangles are congruent, which is a fancy way of saying they have the same side lengths and the same angles. Phrases like side-angle-side (SAS) and angle-angle-side (AAS) might ring a bell. This topic is very polarizing and quickly separates students into those who say “I love geometry!” and “I miss algebra!”. Simply put though, all of these acronyms are just descriptions of the information we are given about a triangle. Note that the order of this information is very important! SAS and SSA both contain one angle and two sides, but both of them won’t prove two triangles are congruent.

Side-Side-Angle Tringles

There is one problematic case of side-side-angle (SSA) or put another way, angle-side-side (I’ll pause here and let you chuckle at the joke every geometry teacher in the country makes at this moment).  Some teachers will correctly note that this situation is not enough to show two triangles are congruent and leave it at that, but there is more that can be explored here. When you are given an SSA triangle, this is called the ambiguous case because it could result in 0, 1, or 2 triangles. This post will focus on demonstrating that no solutions exist.

Step-by-step method of solving

1. Draw the triangle and label all the given information. It doesn’t need to be to scale at all! It also doesn’t matter how you orient the triangle as long as side A is across from angle A, side b is across from angle B, and side c is across from angle C. Just draw and label.
2. Use the Law of Sines to solve for the angle across from the second side. Which one is the second side? Well in SSA you have two sides. One of the angles will pair with a side, like angle A and side a, or angle B and side b. There will be an unused side that isn’t paired. We are going to try to find that angle.

Let’s walk through an example.

Side-Side-Angle Triangle Example

$$B = 55 ^{\circ}, b=8.99,a=26.22$$

Following the steps above, let’s draw this triangle!

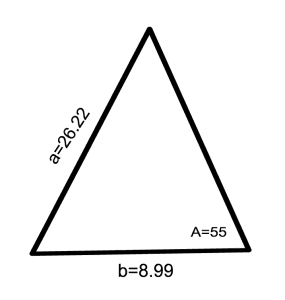
Notice that angle A is in the bottom right corner. Often students and teachers like to put angle A at the top. It doesn’t matter as long as the sides go across from the angles! You could draw the triangle like this as well. It won’t change the answer.

 

The Law of Sines states that $$\displaystyle \frac{\sin(A)}{a}=\frac{\sin(B)}{b}=\frac{\sin(C)}{c}$$ As mentioned in step 2, we are going to try to find angle B, because it is the angle opposite the second side. (We can’t find angle C or side c right now because we don’t have any information on that side. Try to solve for either of those to convince yourself if that seems unclear.)

$$\begin{align*}
\frac{\sin(55^{\circ})}{26.22}&=\frac{\sin(B)}{8.99} \\
\sin(B) &= \frac{26.22\sin(55^{\circ})}{8.99} \\
\sin(B) &\approx 2.389
\end{align*}$$

Uh oh! We have a problem! Remember that the range of $\sin(x)$ is $[-1,1]$, meaning the output of this function has to stay between these two values. When we get an answer like the one above, it is impossible to find an angle to make the statement true and we have an impossible triangle.

Try putting $\sin^{-1}(2.389)$ into your calculator to check and you’ll get an error message.

Key Points

Draw a triangle and label your given information. Do not worry at all if the triangle is to scale. Use the Law of Sines to find the second angle that connects to the second side you are given and simplify. When you get $\sin(A), \sin(B), \sin(C)$ is outside of $[-1,1]$, you can confidently answer that this triangle does not exist. If it does fall within that range, then you should move on to deciding if 1 or 2 triangles are possible.

That topic will be covered in another blog post soon.