How Can We Jump When the Ground Does No Work?

It is relatively common on Physics Forums to see arguments that are effectively similar to the following:

When we jump off the ground, the ground does not move. Because of this, the force from the ground on us does zero total work. Since the force does no work, we cannot gain any kinetic energy. We therefore cannot jump off the ground.

Now, the conclusion here is false. The world high jump record is 2.45 meters, definitely larger than zero. So where did the energy come from? This Insight seeks to clarify this in a fairly accessible way.

An idealized example

Before jumping off into bipedal mammals competing in the high jump, let us look at an idealized example. This example will help us understand what is going on a little better.

A mass $m$ has an ideal spring of length $\ell$ and spring constant $k$ attached to it. The mass and spring are pressed against a fixed wall such that the spring is compressed by a distance $D$, see the figure below.

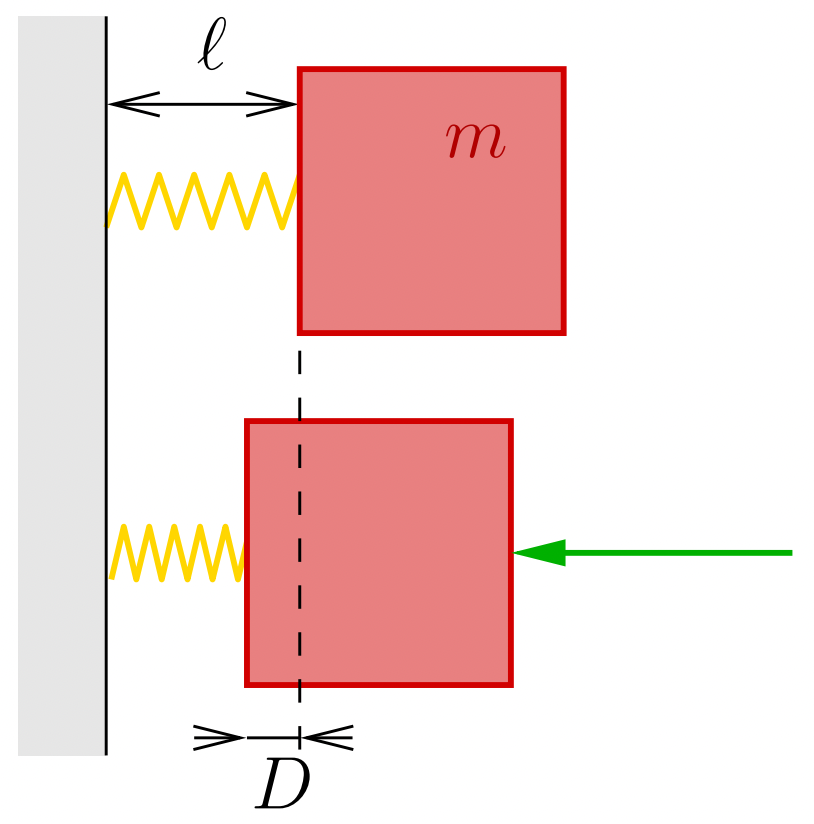
A mass $m$ is attached to a spring of length $\ell$ next to a wall. When pressed towards the wall, the spring compresses by a distance $D$.

In other words, the mass of the spring is zero, and the force at its ends is given by Hooke’s law. All of this occurs in a horizontal plane, meaning that we do not need to deal with gravity.

Once released, the spring pushes the mass away from the wall. Similar to the jump off the ground, the wall provides no work. By the same reasoning as in our example argument, the mass cannot move away from the wall.

Where is the energy?

So where does the energy come from? Because the spring will certainly launch the mass away from the wall. To answer this, let us first look at the process of compressing the spring. In particular, we consider a small segment of the spring between the coordinates $x_0$ and $x_0 + \Delta x$ when the spring is relaxed. The compressing force pushing on its ends is $F = -\kappa \epsilon$ by Hooke’s law (see the figure below). Here $\epsilon$ is the strain and $\kappa = k \ell$.

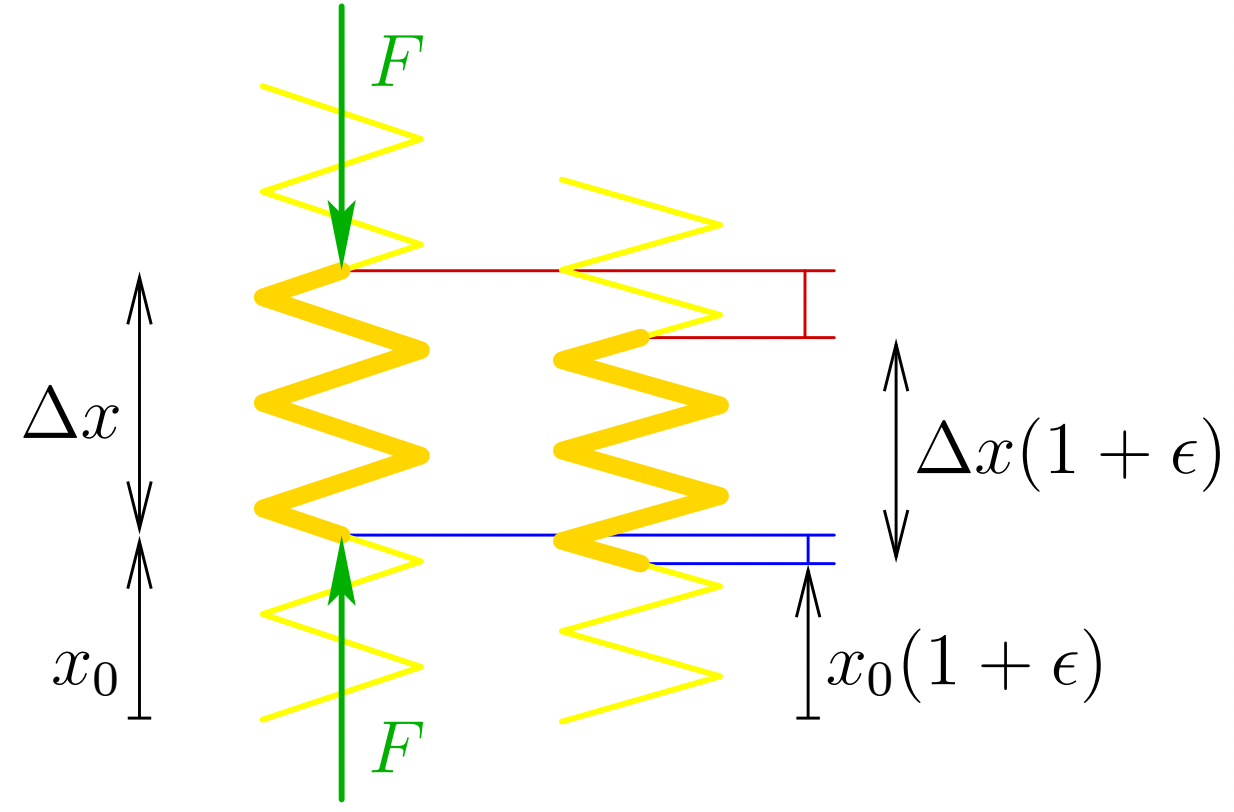
To compress a spring segment $\Delta x$, forces equal in magnitude but opposite in direction act on both ends. The displacement at the top of the segment (red) is larger than that at the bottom (blue). Therefore, the upper force does positive work of a larger magnitude than the negative work of the lower force.

Changing the strain by $d\epsilon$, the lower end of the string segment moves by $x_0 d\epsilon$ and the upper by $(x_0+\Delta x)d\epsilon$. The total work done on the segment becomes $$dW = F x_0 d\epsilon – F (x_0+\Delta x) d\epsilon = \kappa \epsilon \Delta x \, d\epsilon.$$ Integrating this from no strain to a strain $\epsilon_0$ leads to $$W = \kappa \Delta x\int_0^{\epsilon_0} \epsilon\,d\epsilon = \frac{\kappa\epsilon_0^2}{2} \Delta x.$$ This is the total energy stored in the spring segment at strain $\epsilon_0$.

That the total energy stored in the spring is $$W = \frac{kd^2}{2}$$, where $d$ is the compression of the spring and $k = \kappa/\ell$ is the spring constant, follows directly from the above.

Energy flux

The discussion above suggests the idea that energy can enter or exit an object and remain as internal energy. This occurs through forces acting on the object performing work. A force $\vec F$ acting on an object over a displacement $d\vec r$ will do a total work of $\vec F \cdot d\vec r$. In the example above, $x_0 d\epsilon$ replaces $\vec dr$ for the lower end as this is the lower end’s displacement and we work in one dimension. Similarly, we have a directed one-dimensional force $F$ instead of the three-dimensional vector $\vec F$.

The quantity $F x_0 d\epsilon = – \kappa \epsilon x_0 d\epsilon$ is, therefore, a measure of the amount of energy flowing upward through the spring at position $x_0$ when the strain changes by $d\epsilon$. When $\epsilon$ is negative, i.e., when the spring is compressed, energy will flow upward if $d\epsilon$ is positive. In other words, when the spring is compressing energy flows left in the spring and right when it is decompressing.

Launching the mass

The spring will decompress during the launch of the mass. The internal energy stored in the spring then flows from the spring into the mass. Denoting the compression of the spring $D(t)$, we find that $$D(t) = D_0 \cos(\omega t)$$ with $\omega^2 = \kappa/m\ell$ during the launch, where the initial compression is $D_0$.

The launch time interval is $0 \leq t \leq \pi/2\omega$. The strain $\epsilon$ is related to $D$ as $\epsilon = -D/\ell$. We therefore obtain $$\frac{d\epsilon}{dt} = -\frac{D'(t)}{\ell} = \frac{D_0\omega \sin(\omega t)}{\ell}.$$

Consequently, the energy flowing up through the spring at position $x$ is $$\frac{dW}{dt} = -\kappa \epsilon x\, d\epsilon = \kappa \frac{D_0\cos(\omega t)}{\ell} x \frac{D_0\omega \sin(\omega t)}{\ell} = \frac{\kappa D_0^2}{2\ell^2} \omega x\sin(2\omega t).$$

Naturally, this grows linearly with $x$. As all energy released from the spring flows into the mass, the energy flow gets larger the closer to the mass we get.

Relation to the jumper

A jumper’s legs are by no means an ideal spring. However, the discussion above does give some insight into the issue presented in the beginning:

• The upper body will receive net work from the legs much like the mass received net work from the spring during launch.
• The net work from the ground is zero.
• The energy is provided from internal energy stored in the jumper’s muscles. Just as the energy here was provided from internal energy stored in the spring.

Some differences are also notable:

• Unlike the spring, the jumper’s lower body will have non-zero kinetic energy at the end.
• Energy will also be lost in the form of heat as the efficiency of conversion of internal energy to macroscopic kinetic energy is not 100%.

While the ground does not do work on the jumper, the jumper’s momentum is provided by the force from the ground. This momentum is distributed throughout the jumper’s body by internal forces.