A Poor Man’s CMB Primer: The Birth of a Cosmic Background Radiation

 

The early universe was hot. So hot that nuclei boiled. The great thermal energy of the universe overwhelmed the confining efforts of the nuclear and electromagnetic forces, and droves of fundamental particles—quarks, gluons, leptons, photons—bounced and jostled in a tightly coupled plasma. The universe, however, has a built-in cooling mechanism: expansion. Matter particles dilute and photon wavelengths stretch as the universe expands in a process called redshift. Soon, protons and neutrons condense out of the plasma as the strong force overcomes the waning thermal wanderings of quarks and gluons. Over the next ten minutes, these nucleons join to form nuclei of deuterium, helium, and other light elements. The protons and nuclei, however, are naked—it’s still too hot for electrons to join them in the formation of neutral atoms. The universe will remain in this ionized state for the next few hundred thousand years.

The thing about ionized plasma is that photons have a hard time getting around: there is no shortage of charged particles ready for a collision. The average distance traveled by a photon before collisions is the mean free path, $\lambda_\gamma = (n \sigma)^{-1}$, determined by the number density of the charge-carriers, $n$, and the scattering cross-section, $\sigma$. To get a feel for just how wadded up everything was in the early universe, let’s find the mean free path of photons when the universe was one second old, at a torrid $T=10^{10}\, {\rm K}$. This is the epoch of nucleosynthesis when protons and neutrons fuse to form the light nuclei. Though we are technically in the realm of Compton scattering, $T > m_e$, the Thomson scattering cross-section will suffice for an order-of-magnitude estimate: $\sigma_T = 6.65\times 10^{-25}\, {\rm cm}^2$, where $\sigma_T$ is proportional to the classical electron radius, $e^4/m_e^2$. As for the charge-carrier density, we can safely ignore everything except the electrons: though charge neutrality of the universe requires that the densities of free electrons and protons balance, $n_e = n_p$, scattering off the much heavier protons is suppressed by a factor of a million relative to electrons (owing to the factor $1/m^2$ in $\sigma_T$)¹.

It’s safe to treat the electrons as radiation (they are still quite hot, about the temperature of a supernova). To get the number density, we need to integrate the Fermi-Dirac distribution function,
$$f(E) = \frac{1}{\exp\left[\left(E – \mu\right)/T\right]+1},$$
over the full phase space in the relativistic limit, $(T \gg \mu, m)$,
$$n_e = \frac{g}{2\pi^2}\int_0^\infty \frac{E^2}{\exp E/T+1}\, {\rm d}E,$$
where $\mu$ is the chemical potential (the energy associated with a change of particle number), $g = 2$ is the fermion degeneracy, and $E=p^2/2m_e$ is the particle kinetic energy. In a feat akin to bending a spoon with ones mind,²
$$\label{n} n_e = \frac{3\zeta(3)}{2\pi^2}T^3,$$
where $\zeta(3) \approx 1.2$ is the Riemann zeta function (of 3). The mean free path is found to be $\lambda_\gamma \approx 7 \times 10^{-8}\, {\rm m}$, or about the size of a typical virus. So, yeah: tiny. The universe is effectively opaque.

As the universe continues to cool we eventually expect neutral hydrogen to form and the free charge-carrier density to decline rapidly in a process confusingly called recombination (there’s nothing “re-” about it.) Naively, we might expect this transition to occur as the universe cools through $T = 10^5\, {\rm K}$, which corresponds dimensionally to the ionization energy of hydrogen, $E = 13.6 \, {\rm eV}$. At this energy, the reaction $p\, +\, e^{-}\longleftrightarrow H\, +\, \gamma$ should begin to fall out of chemical equilibrium because background photons won’t be able to re-ionize the newly formed hydrogen. With enough electrons locked away in hydrogen atoms, photons will propagate relatively unimpeded across the cosmos. This event, the corollary of recombination called decoupling, is important because these are the very photons that make up today’s cosmic microwave background. Let’s see if this is indeed what happens by finding the photon mean free path when the universe has cooled down below $13.6\, {\rm eV}$ to a tepid $1\, {\rm eV}$, corresponding to a temperature of roughly $T = 10^4\, {\rm K}$ (around the temperature of a white dwarf, or really hot habanero). At these temperatures, the electrons are no longer relativistic (since $T \ll m_e$) and so the number density assumes the classical Boltzmann form,
$$\label{boltz} n_e = 2\left(\frac{m_eT}{2\pi}\right)^{3/2}\exp\left(\frac{\mu_e-m_e}{T}\right).$$
After a little work³, the electron density at $T=10^4\, {\rm K}$ is found to be $n_e \approx 10^4\, {\rm cm}^{-3}$. The mean free path is found to be $\lambda_\gamma \approx 1.5 \times 10^{20}\, {\rm cm}$, or around $1000$ parsecs. This is about the distance to the star Deneb in the constellation Cygnus, which is, incidentally, the most distant star visible to the naked eye under urban skies. That’s quite a distance—significantly longer than the mean free path during nucleosynthesis—but still only a sliver of the observable universe at that time. Hardly “across the cosmos”. Apparently the universe is still quite ionized even at $E=1\, {\rm eV}$. What’s going on?

For one, there are hugely many more photons than charge carriers per unit volume. The hugeness of this disparity is the result of the tiny and mysterious matter-antimatter asymmetry in the early universe: most of the matter and all of the antimatter annihilated to create a glut of photons, leaving only a tiny residual matter density. All told, there are roughly 10 billion photons for every electron in the universe. With so many more photons than electrons, even at $T = 10^4\, {\rm K}$ there were enough photons all the way out in the ultraviolet tail of the blackbody spectrum with energies greater than $13.6\, {\rm eV}$ to keep hydrogen relatively well-ionized. To find out just how many, we need to consult the Planck spectrum,
$$\label{planck} n_\gamma = \frac{1}{\pi^2}\int_{13.6}^\infty \frac{E^2}{\exp(E/T) – 1}\, {\rm d}E,$$
which gives $n_\gamma \approx 3 \times 10^9\, {\rm cm}^{-3}$ at $T = 10^4\, {\rm K}$. In comparison with $n_e \approx 10^4\, {\rm cm}^{-3}$ determined above, there are around $3\times 10^5$ photons with an energy greater than $E = 13.6\, {\rm eV}$ for every electron! This means that for every neutral hydrogen atom that forms, there are schools of high-energy photons available to re-ionize it.

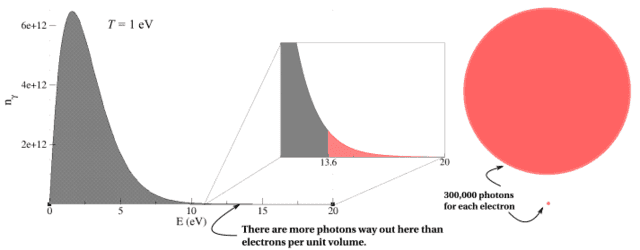
Fig 1. Blackbody spectrum of photons at $T=1\, {\rm eV}$. At this temperature, there are roughly $10^5$ photons with an energy greater than $13.6\, {\rm eV}$ for every electron

So then when does recombination happen? To find out, we’ll need to do a more careful accounting of the electron and baryon number densities. In particular, we’re going to track the ionization fraction, $X_e = n_e/n_B$, where the baryon density includes the protons and neutral hydrogen, $n_B = n_p + n_H$. We assume that the electrons, baryons, and photons are all in thermal equilibrium, which is true so long as the reaction $p\, +\, e^{-}\longleftrightarrow H\, +\, \gamma$ proceeds rapidly relative to the expansion rate of the universe. In equilibrium, the chemical potentials balance⁴, $\mu_e + \mu_p = \mu_H$: this condition is our starting point. We know from our earlier results that we are well inside the nonrelativistic regime when recombination finally does happen, so all equilibrium abundances have the Boltzmann form, Eq. (4). Writing the condition $\mu_e + \mu_p = \mu_H$ in terms of $n_e$, $n_p$, and $n_H$ gives
$$\frac{n_H}{n_e n_p} = \left(\frac{m_eT}{2\pi}\right)^{-3/2}\exp\left(\frac{m_e + m_p – m_H}{T}\right),$$
To get this expression we’ve taken $m_p = m_H$ in the coefficient of the exponential (but not in the exponent of the exponential because, though tiny, it’s exponentiated!) Shuffling around the number densities gives
$$\frac{n_B^2}{n_e^2} – \frac{n_B}{n_e} = n_B\left(\frac{m_eT}{2\pi}\right)^{-3/2}\exp\left(B/T\right),$$
where charge neutrality of the universe permits us to replace $n_p$ with $n_e$ and $B = m_e + m_p – m_H = 13.6\, {\rm eV}$ is the binding energy of hydrogen. In terms of $X_e$ this becomes
$$\frac{1-X_e}{X_e^2} = \eta n_\gamma \left(\frac{m_eT}{2\pi}\right)^{-3/2} \exp\left(B/T\right),$$
where the baryon-photon ratio, $\eta = n_B/n_\gamma$, has been introduced to trade the leftover $n_B$ for the more workable $n_\gamma$. With $n_\gamma = 2(\zeta(3)/\pi^2)T^3$ (gotten by performing the integral in Eq. (5) from $0$ instead of $13.6\, {\rm eV}$), we find
$$\frac{1-X_e}{X_e^2} = 4\eta\zeta(3)\sqrt{\frac{2}{\pi}}\left(\frac{T}{m_e}\right)^{-3/2} \exp\left(B/T\right).$$
This is the famed Saha Equation giving the equilibrium ionization fraction. It tells us how, at a given temperature, the energy is distributed among the reactants (the various particle species and ionization states) in equilibrium. With $\eta = 6 \times 10^{-10}$, the universe is $90\%$-ionized when $T \simeq 3600\, {\rm K} \simeq 0.3\, {\rm eV}$, a full two orders of magnitude below the hydrogen binding energy.

It might strike you as curious that recombination happens at all. Think about it: even without hordes of hot, ionizing photons flying around, a single recombination directly to the ground state releases a photon with an energy $E > 13.6\, {\rm eV}$. Unless the plasma density is sufficiently low (it isn’t), these photons will readily re-ionize any neutral hydrogen atoms they happen upon. Any recombination—even those too-excited states of hydrogen—releases photons capable of reionizing other neutral hydrogen atoms in the same excited state. Recombination must proceed via very particular transitions through the excited states of hydrogen; for example, the decay from 2s to 1s proceeds via the emission of two photons, neither of which has sufficient energy to excite hydrogen out of the ground state. The other pathway is through Lyman $\alpha$ decay (a $10.2\, {\rm eV}$ photon from 2p to 1s): while absorption of a Lyman $\alpha$ photon will excite neutral hydrogen to a readily-ionizable state (a mere $3.5\, {\rm eV}$ away), as the hydrogen density decreases with the expansion the Lyman $\alpha$ photon redshifts just enough to render it harmless. These transitions work; the problem is that they are slow, and they get slower as the ionization fraction falls. Recall that thermal equilibrium is maintained when reactions proceed at a faster rate than the expansion, $\Gamma \gg H$. Once $\Gamma$ drops below $H$, abundances are locally conserved because the reaction effectively shuts off: the universe doubles in size before the reaction completes. As recombination proceeds and the ionization fraction falls, these key transitions to the hydrogen ground state are too slow to maintain equilibrium and are therefore no longer adequately described by the Saha Equation. As a result, the Saha Equation predicts a more rapid recombination process than actually occurs through these slow, out-of-equilibrium decays. It is possible to do a proper job of this, taking into account out-of-equilibrium interactions involving the excited states of hydrogen, but this is a poor man’s CMB primer⁵. The Saha Equation is sufficient for our purposes—it illustrates the physics and gives an order-of-magnitude estimate of the temperature of recombination.

Putting this all together, we revisit the photon mean free path. With $n_e = X_e n_B = X_e \eta n_\gamma$ giving $\lambda_\gamma = (X_e \eta n_\gamma \sigma)^{-1}$, we can watch the mean free path of the photon grow as the formation of neutral hydrogen really gets going,

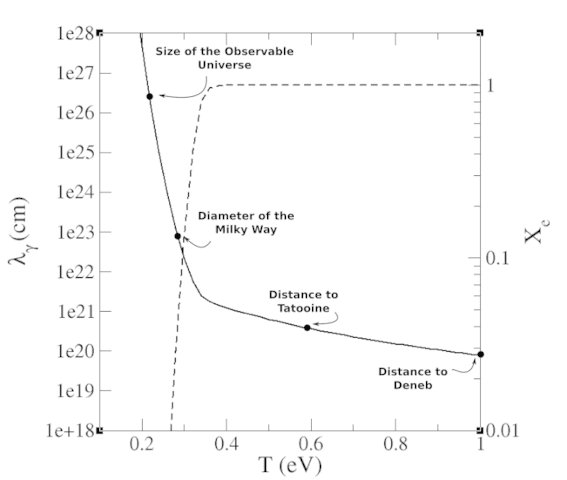
Fig 2. Mean free path, $\lambda_\gamma$ (solid), and ionization fraction, $X_e$ (dashed), vs. temperature.  Photons traverse the whole of the visible universe unimpeded only when the ionization fraction has dropped below $X_e < 0.01$.  These are the CMB photons.

Only when the universe is mostly hydrogen—99 hydrogen atoms for every electron—does it become effectively transparent to photons. These photons, ladies, and gentlemen, are the cosmic background radiation: the CMB is born!

Next time we’ll learn about how these photons, now cooled into the microwave band of the spectrum, look to us on Earth today. We’ll spend some time dissecting the marvelous CMB maps created by WMAP and Planck, two of the many contemporary CMB observatories.

References and Footnotes

[1] Steven Weinberg, Cosmology, Oxford (2008). Section 2.3 in particular.

¹And the even heavier and more dilute deuterium, helium, and lithium nuclei can be likewise ignored. back
²The integral $\int_0^\infty dE\,\frac{E^2}{e^{E/T}+1}$ can be solved via geometric series expansion and some low-level shenanigans. First, substitute $x=E/T$ giving
$$I = T^3\int_0^\infty dx\, \frac{x^2}{e^x+1}.$$
The idea is to perform a binomial expansion involving the exponential:
$$I = T^3\int_0^\infty dx\, e^{-x}(1+e^{-x})^{-1}x^2 = T^3\int_0^\infty dx\, \left[\sum_{k=0}^\infty(-1)^ke^{-(k+1)x}\right]x^2.$$
After another change of variables, $y = (k+1)x$,
$$I = T^3\sum_{k=0}^\infty(-1)^k \frac{1}{(k+1)^3}\int_0^\infty dy\,y^2e^{-y}.$$
The keen eye might recognize $\int_0^\infty dy\,y^2e^{-y}$ as the gamma function, $\Gamma(3)$. This, together with a slight nudge to the bottom limit of the summation we can rewrite things as
$$I = -T^3\Gamma(3)\sum_{k=1}^\infty \frac{(-1)^k}{k^3}.$$
To get rid of the annoying $(-1)^k$ term, we break the summation up into an even and an odd part, and then rework things to get two full summations back,
$$I = -T^3\Gamma(3)\left(\sum_k^{\rm even} \frac{1}{k^3} – \sum_k^{\rm odd}\frac{1}{k^3}\right) = -T^3\Gamma(3)\left(\frac{2}{2^3}\sum_{l=1}^{\infty} \frac{1}{l^3} – \sum_{k=1}^{\infty}\frac{1}{k^3}\right).$$
After the dust settles, we see that two copies of the Riemann zeta function have emerged, $\zeta(3) = \sum_k 1/k^3$, allowing us to write
$$I = T^3\zeta(3)\Gamma(3)\left(1 – \frac{1}{2^2}\right) = \frac{3}{2}\zeta(3)T^3.$$back
³This is actually a little tricky: we can’t solve $n_e$ directly because we don’t know $\mu_e(T)$ in general. Instead, we need to round up expressions for $n_p$ and $n_H$, the densities of protons and hydrogen, together with the constraints $n_e = n_p$, $n_B = n_H + n_p = \eta n_\gamma$, and $\mu_H = \mu_e + \mu_p$, put them in a mixer, and shake vigorously. The result is a quadratic in $n_e$ without any of the $\mu_i$. If some of these constraints look unfamiliar, it’s because we haven’t discussed them yet. We’ll get to them: they are central to later developments and I don’t want to jump the gun. back
⁴$\mu_\gamma = 0$ because photons are freely created and destroyed in recombination reactions. back
⁵Weinberg’s cosmology text [1] has a thorough discussion of this calculation. back

Click For Forum Comments