The Electric Field Seen by an Observer: A Relativistic Calculation with Tensors

This Insight was inspired by the discussion in “electric field seen by an observer in motion“, which tries to understand the relation between two expressions:

• the definition of the electric field as seen by an observer (expressed as an observer-dependent 4-vector, as decomposed from the Maxwell field tensor $E_{a}=F_{ab}v^b$, as found in Wald’s General Relativity [p. 64, Eq (4.2.21)] )
• the Lorentz Transformation of the Electric Field, in 3-vector form

I was going to reply to a comment on something I said (here) but then realized that my post was getting too large. So, here it is in the Insight.

$\def\MACROS{} \def\hv{\hat v} \def\hw{\hat w} \def\hvx{\hat {\bar x}} \def\hwx{\hat {\tilde x}} \def\hvy{\hat {\bar y}} \def\hwy{\hat {\tilde y}} \def\hvz{\hat {\bar z}} \def\hwz{\hat {\tilde z}} \def\vE{\bar E} \def\vB{\bar B} \def\vBs{{}^*\bar B} \def\wE{\tilde E} \def\wB{\tilde B} \def\wBs{{}^*\tilde B} \def\vW{\bar W} \def\wW{\tilde W}$

(I am only going to work on the electric field… the magnetic field is handled similarly.)

We begin with a definition seen in Wald’s General Relativity text.
However, we use the $(+,-,-,-)$ signature convention. We use the abstract-index notation,

(On another website I visit, there seem to be some misconceptions concerning this spacetime formulation.
At the end of this document, I have included some quotes from some relativity textbooks that use this spacetime formulation.)

Definitions

Given an antisymmetric tensor $F_{ab}$, a metric $g_{ab}$ with signature $(+,-,-,-)$, and a unit timelike vector $\hv^a$ ($g_{ab} \hv^a \hv^b=+1$),
define
$E_a=F_{ab} \hv^b$ (note: $E_a \hv^a=0$)
and
$B_a={}^*F_{ab} \hv^b=\frac{1}{2} \epsilon_{abcd}F^{cd}\hv^b$ (note: $B_a \hv^a=0$).
Thus, the vectors $E^a=g^{ab}E_b$ and $B^a=g^{ab}B_b$ are orthogonal to $\hv^a$.
For convenience, we define two antisymmetric tensors $E_{ab}=2E_{[a}\hv_{b]}$ and $B_{ab}=2B_{[a}\hv_{b]}.$
Then ${}^* B_{ab}=\epsilon_{abcd} B^c \hv^d$ and ${}^* E_{ab}=\epsilon_{abcd} E^c \hv^d$.
Note:
$$E_{ab}\hv^b
=E_a \hv_b \hv^b – E_b \hv_a \hv^b
= E_a (\hv_b \hv^b) – 0 =
E_a \quad ({\rm since\ } \hv_b \hv^b =1)$$
(so, $B_{ab}\hv^b=B_a$, $E_{ab}\hv^b=E_a$, ${}^* B_{ab}\hv^b=0$, ${}^*E_{ab}\hv^b=0$).

Thus, $$F_{ab}=E_{ab}-{}^*B_{ab}$$
since $E_a=(E_{ab}-{}^*B_{ab})\hv^b$ and $B_a=({}^*E_{ab}-{}^{**}B_{ab})\hv^b=({}^*E_{ab}+B_{ab})\hv^b$

---

The Electric Field according to Vic and according to Will

If $F_{ab}$ is a Maxwell field, then $E_a$ and $B_a$ are the electric and magnetic parts of the field according to an observer Vic with four-velocity $\hv^a$ (a future-directed unit timelike vector).

What are the electric and magnetic parts according to another observer Will with four-velocity $\hw^a$?

To distinguish the observer’s electric and magnetic parts
and to emphasize the observer-dependence of this decomposition of the Maxwell field tensor,
we refer to

• Vic‘s parts with a bar as $\vE_a$ , $\vB_a$, $\vE_{ab}$ and $\vB_{ab}$
  and
• Will‘s parts with a tilde as $\wE_a$ , $\wB_a$, $\wE_{ab}$ and $\wB_{ab}$.
• [To emphasize the observer-dependent decomposition,
  we could have used $’$ and $”$… but not “primed” and “unprimed”.]

According to Vic (with 4-velocity $\hv^a$),
Will’s 4-velocity can be written in these suggestive forms
\begin{align*}
\hw^a
&= (w_{||v})^a+(w_{\bot v})^a \\
&=\quad \alpha \hv^a\ +\ \ \beta^a \\
&=\quad \gamma \hv^a\ +(\gamma \vW^a) \\
&=\quad \gamma \hv^a\ +(\gamma \vW \hv_{\bot}^a)\\
&=\cosh\theta \ \hv^a\ +\sinh\theta\ \hv_{\bot}^a
\end{align*}
where $\alpha>0$ (since $\hv^a$ and $\hw^a$ are both future-directed) and $0=\hv^a \beta_a$, $0=\hv^a \vW_a$ and $0=\hv^a \hv_{\bot a}$.
(That is, $\beta^a$, $\vW^a$, and $\hv_{\bot}^a$ are spacelike—in fact, “purely-spatial vectors according to $\hv^a$”.)
[Technically, we should “bar” all of the quantities on the right-side. But we’ll just keep the “bars” on the vectors we will use.]

---

Some formulae

To motivate these expressions,
\begin{align*}
\hv_a \hw^a = \hv_a(\quad \alpha \hv^a\ +\ \ \beta^a)=\alpha.
\end{align*}

The square norm of the spacelike vector $\beta^a$ is
\begin{align*}
\beta^a \beta_a
&= (\hw^a -\alpha \hv^a)(\hw_a -\alpha \hv_a)\\
&= (\hw^a \hw_a -2\alpha \hv^a \hw_a + \alpha^2 \hv^a\hv_a)=1-2\alpha^2+\alpha^2=1-\alpha^2.
\end{align*}
Since, for nonzero $\beta^a$, we have $\beta^a \beta_a <0$ (in the $(+,-,-,-)$-convention),
we have $(1-\alpha^2)<0$ — that is, $\alpha^2 >1$.
Since we established $\alpha>0$ (due to the future-timelike relation), we have $\alpha >1$ (for nonzero $\beta^a$).
For zero $\beta^a$, we would have $\alpha=1$.
Thus, generally $\alpha \geq 1$.

Since $\hw^a$ is a spacetime-displacement of Will according to Vic, the ratio of the magnitude of the spatial-displacement to the temporal-displacement is the “speed $|W|$ of Will according to Vic”:
\begin{align*}
|W|&=\frac{\sqrt{\beta^a\beta_a}}{\alpha}
=\frac{\sqrt{\alpha^2-1}}{\alpha},
\end{align*}
which is always less than $1$ (in our units) since $\alpha^2\geq 1$.

Let us define the spatial-velocity vector of
Will according to Vic
$$\vW^a=W \hv_{\bot}^a =\alpha \beta^a$$
Thus $$ \vW^2= -\vW^a \vW_a=\frac{\alpha^2-1}{\alpha^2}$$
By solving for $\alpha$, we have
$$\alpha =\frac{1}{\sqrt{1-W^2}},$$
which suggests that we can identify
$$\alpha=\hv_a \hw^a =\gamma=\cosh\theta \qquad
\mbox{ and }\qquad
\sqrt{\alpha^2-1}=\sinh\theta$$
and
$$W=\frac{\sinh\theta}{\cosh\theta}=\tanh\theta.$$
Thus, we write:
$$\hw^a= \gamma \hv^a + \gamma \vW^a.$$

---

The Electric Field

Starting with the electric part $\wE_a$ according to Will ($\hw^a= \gamma \hv^a + \gamma \vW^a$),
we have
\begin{eqnarray*}
\wE_a
&=& F_{ab}\hw^b\\
&=& F_{ab}(\gamma \hv^b + \gamma \vW^b)\\
&=& \gamma (\vE_{a} + F_{ab}\vW^b )\\
&=& \gamma (\vE_{a} + (\vE_{ab}-\vBs_{ab})\vW^b )\\
&=& \gamma (\vE_{a} + (\vE_a \hv_b \vW^b – \vE_b \hv_a \vW^b) –
\epsilon_{abcd} \vB^c \hv^d \vW^b )\\
&=& \gamma (\vE_{a} + (\quad 0\quad ) + (-\vE_b \vW^b) \hat v_a –
\epsilon_{abcd} \vB^c \hv^d \vW^b )\\
&=& \gamma (\vE_{a} – (\vE_b \vW^b) \hv_a –
(-1)^3 \hv^d\epsilon_{dabc} \vW^b \vB^c )\\
&=& \gamma (\vE_{a} – (\vE_b \vW^b) \hv_a \ \ + \
\hv^d\epsilon_{dabc} \vW^b \vB^c )\\
&=& -\gamma (\vE_b \vW^b) \hv_a + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\end{eqnarray*}
where we have isolated the component of Will’s electric part $\wE_a$ that is parallel to Vic’s 4-velocity; the second term is purely-spatial according to Vic.

---

Special case: $W^a=W\hvx^a$

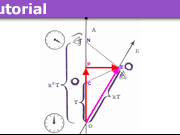
Take the special case where Will’s relative velocity is along Vic’s $x$-axis, which we write as$W^a=W\hvx^a$.

Recalling the form of the Lorentz Transformation for relative motion along the $x$-axis,
\begin{eqnarray*}
\hat t’^a &=& \gamma(\hat t^a+V_{rel}\ \hat x^a) \\
\hat x’^a &=& \gamma(V_{rel}\ \hat t^a+ \hat x^a)\\
\hat y’^a &=& \hat y^a\\
\hat z’^a &=& \hat z^a
\end{eqnarray*} we express this transformation with our notation encoding the observer 4-velocities
\begin{eqnarray*}
\hw^a &=& \gamma(\hv^a+W\hvx^a)\\
\hwx^a &=& \gamma(W\hv^a+\hvx^a)\\
\hwy^a &=& \hvy^a\\
\hwz^a &=& \hvz^a.
\end{eqnarray*}

Due to our signature $(+,-,-,-)$ convention,
define (with the over-tilde) $\wE_{(\tilde x)}=-\hwx^a \wE_a = \hwx \cdot \vec \wE$ to be Will’s $\tilde x$-component of the electric field $\wE_a$ that Will measures.
Similarly,
define (with the over-bar) $\vE_{(\bar x)}=-\hvx^a \vE_a = \hvx \cdot \vec \vE$ to be Vic’s $\bar x$-component of the electric field $\vE_a$ that Vic measures.

We calculate Will’s-$\hwx$-component of the Will’s-Electric Field in terms of Vic’s–$\hvx$-component of the Vic’s Electric Field
\begin{eqnarray*}
\hwx^a \wE_a
&=&
\gamma(W\hv^a+\hvx^a)
\left(
-\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\right)
\\
&=&
\gamma(W\hv^a+\hvx^a)
\left( -\gamma W(\vE_b \hvx^b) \hv_a + \gamma (\vE_{a} +
W\hv^d\epsilon_{dabc} \hvx^b \vB^c ) )\right)
\\
&=&
\gamma^2 \left[ -W^2 (\vE_b \hvx^b) \hv^a\hv_a\ + 0 + 0 +
\hvx^a \vE_a + \hvx^a W\hv^d\epsilon_{dabc} \hvx^b \vB^c \right]\\
\wE_{(\tilde x)}
&\stackrel{*}{=}& \gamma^2 \left[ -W^2 \vE_{(\bar x)} \qquad \quad\ + 0 + 0 +\ \vE_{(\bar x)} \ + \qquad 0 \qquad\right]
\\
&=& \gamma^2 \left[ -W^2 +1 \right] \vE_{(\bar x)}
\\
\wE_{(\tilde x)}
&=& \vE_{(\bar x)}
\end{eqnarray*}
(The * indicates that we have canceled the common factor $(-1)$ that arose in our definition of components in this $(+,-,-,-)$ convention.)

For the $y$-  and $z$-components of the Electric Field, we have
\begin{eqnarray*}
\hwy^a \wE_a
&=& \hvy^a
\left(
-\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\right)
\\
&=& \gamma \left[ \qquad\quad 0 \qquad\quad\ + \hvy^a \vE_a
+ \hvy^a \hv^d\epsilon_{dabc} \vW^b B^c \right]
\\
\wE_{(\tilde y)}
&\stackrel{*}{=}& \gamma \left[ \phantom{ \qquad\quad 0 \qquad\quad\ +\ } \vE_{(\bar y)} + ( \vW_{(\bar z)} \vB_{(\bar x)} – \vW_{(\bar x)} \vB_{(\bar z)}) \right]\\
\wE_{(\tilde y)}
&=& \gamma (\vE_{(\bar y)}- W \vB_{(\bar z)})
\end{eqnarray*}

 

\begin{eqnarray*}
\hwz^a \wE_a
&=& \hvz^a
\left(
-\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\right)
\\
&=& \gamma \left[ \qquad\quad 0 \qquad\quad\ + \hvz^a \vE_a
+ \hvz^a \hv^d\epsilon_{dabc} \vW^b B^c \right]
\\
\wE_{(\tilde z)}
&\stackrel{*}{=}& \gamma \left[ \phantom{\qquad\quad 0 \qquad\quad\ +\ \ }
\vE_{(\bar z)} + ( \vW_{(\bar x)} \vB_{(\bar y)} – \vW_{(\bar y)} \vB_{(\bar x)})
\right]\\
\wE_{(\tilde z)}
&=& \gamma (\vE_{(\bar z)}+ W \vB_{(\bar y)})\\
\end{eqnarray*}

For completeness, let’s evaluate Will’s temporal component.
\begin{eqnarray*}
\hw^a \wE_a
&=& \gamma(\hv^a+\vW^a)
\left(
-\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\right)
\\
0
&=& \gamma^2 \left[
-(\vE_b \vW^b) \hv^a \hv_a \quad + 0
\quad +0 \quad + \vW^a \vE_a \quad
+ 0
\right]
\\
0
&=& \gamma^2 \left[ -(\vE_b \vW^b)+ \phantom{\qquad\quad 0 \qquad\quad + }
+ \vW^a \vE_a \quad + 0\right]\\
0
&=& \gamma^2 \left[ \qquad \qquad 0\qquad\qquad \right]\\
\end{eqnarray*}

---

The Lorentz transformation of the Electric Field in 3-vector form

 

Let’s collect the nontrivial relations
\begin{eqnarray*}
\wE_{(\tilde x)}
&=& \vE_{(\bar x)}
\\
\wE_{(\tilde y)}
&=& \gamma (\vE_{(\bar y)}- W \vB_{(\bar z)})
\\
\wE_{(\tilde z)}
&=& \gamma (\vE_{(\bar z)}+ W \vB_{(\bar y)})
\end{eqnarray*}

Let’s rewrite this in a way to suggest a more general form,
resurrecting some terms that evaluated to zero.
\begin{eqnarray*}
\wE_{(\tilde x)}
&=& \gamma \vE_{(\bar x)} – ( \gamma -1) \vE_{(\bar x)}
\\
\wE_{(\tilde y)}
&=& \gamma \left(\vE_{(\bar y)} +
( \vW_{(\bar z)} \vB_{(\bar x)} – \vW_{(\bar x)} \vB_{(\bar z)})
\right)
\\
\wE_{(\tilde z)}
&=& \gamma \left(\vE_{(\bar z)} +
( \vW_{(\bar x)} \vB_{(\bar y)} – \vW_{(\bar y)} \vB_{(\bar x)})
\right)
\end{eqnarray*}
…and a little more suggestive rewriting (without proof)…
\begin{eqnarray*}
\wE_{(\tilde x)}
&=& \gamma \vE_{(\bar x)} – ( \gamma -1) \vE_{(\bar x)}
\\
\wE_{(\tilde y)}
&=& \gamma \left(\vE_{(\bar y)} +
( \vec \vW \times \vec \vB )_{(\bar y)}
\right)
\\
\wE_{(\tilde z)}
&=& \gamma \left(\wE_{(\bar z)} +
( \vec \vW \times \vec \vB )_{(\bar z)}
\right)
\end{eqnarray*}

Recall that the relative-velocity was along the $\bar x$-direction: $\vW^a = W\hvx^a$.
(More precisely, Vic’s $\hv\hvx$-plane coincides with Will’s $\hw\hwx$-plane.)
So, by symmetry, we expect
\begin{eqnarray*}
\wE_{(\tilde x)}
&=& \gamma \left(\wE_{(\bar x)} + ( \vec \vW \times \vec \vB )_{(\bar x)}\right) – ( \gamma -1) \vE_{(\bar x)}
\\
\wE_{(\tilde y)}
&=& \gamma \left(\wE_{(\bar y)} +
( \vec \vW \times \vec \vB )_{(\bar y)}
\right)
\\
\wE_{(\tilde z)}
&=& \gamma \left(\wE_{(\bar z)} +
( \vec \vW \times \vec \vB )_{(\bar z)}
\right)
\end{eqnarray*}
or, more compactly,
\begin{eqnarray*}
\vec \wE=\gamma(\vec {\vE} + (\vec\vW \times \vec\vB) ) -(\gamma-1)\vE_{(\hvx)}\hvx
\end{eqnarray*}

Note that in the derivation of $\wE_{(\tilde x)}$ the quantity $\vE_{(\bar x)}$ on the right-hand-side is really the component of $\vE^a$ along the $\vW^a$-direction.
Since we can write
$$\vE_{(\bar x)}
=\vE_{(|| \hvx )}
= \hvx \cdot \vec \vE $$
and
$$\vE_{(\bar x)}\hvx
=\vE_{(|| \hvx )} \hvx
= \left( \hvx \cdot \vec \vE\right) \hvx, $$
we can write this more generally for an arbitrarily-directed $\vec\vW$ as
$$\vE_{(|| \vW)} = – \hat \vW^a \vE_a = \hat\vW \cdot \vec \vE $$
so that
$$\vE_{(|| \vW)}\hat \vW = \left(\hat \vW \cdot \vec \vE\right) \hat\vW $$

---

Thus, we obtain the more general expression
\begin{eqnarray*}
\vec \wE &=&\gamma(\vec {\vE} + (\vec \vW \times \vec\vB) ) -(\gamma-1)\left(\hat \vW \cdot \vec \vE\right) \hat\vW
\end{eqnarray*}
which agrees with the last set of equations for the Electric Field on https://www.physicsforums.com/threads/electric-field-seen-by-an-observer-in-motion.1000677/ .

The magnetic field is handled in a similar way.

 

Update:

Using an identity involving the definition of the time-dilation factor $$\gamma = \frac{1}{\sqrt{1-W^2}},$$
the above equation can be written in an alternative form, as follows.

First, the identity.
Let $T=(\gamma-1)$.
Then,

\begin{eqnarray*}
T(\gamma+1)
&=&(\gamma-1)(\gamma+1)\\
&=&\gamma^2-1\\
&=&\frac{1}{1-W^2}-1\\
&=&\frac{1-(1-W^2)}{1-W^2}\\
&=&\frac{W^2}{1-W^2}\\
&=&\gamma^2 W^2
\end{eqnarray*}

Thus,
\begin{eqnarray*}
T=(\gamma-1)
&=&\frac{1}{\gamma+1}\gamma^2 W^2=\frac{\gamma^2W^2}{\gamma+1}
\end{eqnarray*}

(In terms of rapidity, $T=(\cosh\theta -1)$. Then  $T(\cosh\theta+1)=(\cosh^2\theta -1)=\sinh^2\theta$.

Thus,

$$T=(\cosh\theta-1)=\frac{\sinh^2\theta}{\cosh\theta+1}.)$$

 

So, the expression for the electric field can be written
\begin{eqnarray*}
\vec \wE
&=&\gamma(\vec {\vE} + (\vec \vW \times \vec\vB) ) -(\gamma-1)\left(\hat \vW \cdot \vec \vE\right) \hat\vW\\
&=&\gamma(\vec {\vE} + (\vec \vW \times \vec\vB) ) -\frac{\gamma^2 W^2}{\gamma+1}\left(\hat \vW \cdot \vec \vE\right) \hat\vW\\
&=&\gamma(\vec {\vE} + (\vec \vW \times \vec\vB) ) -\frac{\gamma^2 }{\gamma+1}\left(\vec \vW \cdot \vec \vE\right) \vec\vW\\
\end{eqnarray*}

 

 

 

---

Update:

In hindsight, we can see the final result more directly.
Begin with

\begin{eqnarray*}
\wE_a
&=& F_{ab}\hw^b\\
&=& F_{ab}(\gamma \hv^b + \gamma \vW^b)\\
&\vdots&\\
&=& -\gamma (\vE_b \vW^b) \hv_a + \gamma (\vE_{a} +
\hv^d\epsilon_{dabc} \vW^b \vB^c )
\end{eqnarray*} where we have isolated the component of Will’s electric part $\wE_a$ that is parallel to Vic’s 4-velocity; the second term is purely-spatial according to Vic.

For arbitrary $\vW^a$,
we write the Lorentz transformations as
\begin{eqnarray*}
\hw^a &=& \gamma(\hv^a+W\hat\vW^a)\\
\hat\wW^a &=& \gamma(W\hv^a+\hat\vW^a)\\
\hat\wW_{\bot}^a &=& \hat\vW_{\bot}^a.
\end{eqnarray*}

So, the component parallel to $\vec\vW$ is

\begin{eqnarray*}
\hat\wW^a \wE_a
&=& \gamma(W\hv^a+\hat\vW^a) \left( -\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} + \hv^d\epsilon_{dabc} \vW^b \vB^c ) \right) \\
&=& \gamma(W\hv^a+\hat\vW^a) \left( -\gamma W(\vE_b \hat\vW^b) \hv_a + \gamma (\vE_{a} + \hv^d\epsilon_{dabc} \vW^b \vB^c ) )\right) \\
&=& \gamma^2 \left[ -W^2 (\vE_b \hat\vW^b) \hv^a\hv_a\ + 0 + 0 + \hat\vW^a \vE_a + \hat\vW^a\hv^d\epsilon_{dabc} \vW^b \vB^c \right]\\
\wE_{(||\wW)}
&\stackrel{*}{=}& \gamma^2 \left[ -W^2 \vE_{(|| \vW)} \qquad \quad\ + 0 + 0 +\ \vE_{(|| \vW)} \ +(\vec \vW \times \vec \vB)_{(|| \vW)} \right] \\
&\stackrel{*}{=}& \gamma^2 \left[ -W^2 \vE_{(|| \vW)} \qquad \quad\ + 0 + 0 +\ \vE_{(|| \vW)} \ + \qquad 0 \qquad\right] \\
&=& \gamma^2 \left[ -W^2 +1 \right] \vE_{(|| \vW)}
\\ \wE_{(||\wW)} &=& \vE_{(|| \vW)}
\end{eqnarray*}

 

and the component perpendicular to $\vec\vW$ is

\begin{eqnarray*}
\hat\wW_{\bot}^a\wE_a &=& \hat\vW_{\bot}^a\left( -\gamma (\vE_b \vW^b) \hv_a \ \ + \gamma (\vE_{a} + \hv^d\epsilon_{dabc} \vW^b \vB^c ) \right) \\
&=& \gamma \left[ \qquad\quad 0 \qquad\quad\ + \hat\vW_{\bot}^a\vE_a + \hat\vW_{\bot}^a \hv^d\epsilon_{dabc} \vW^b \vB^c \right] \\
\wE_{{(\bot\wW)}}
&\stackrel{*}{=}& \gamma \left[ \phantom{\qquad\quad 0 \qquad\quad\ +\ \ } \vE_{(\bot\vW)}+ (\vec \vW \times \vec\vB)_{(\bot\vW)} \right]\\
\wE_{{(\bot\wW)}}
&=& \gamma (\vec\vE+ (\vec \vW\times  \vec\vB) )_{(\bot\vW)}\\
\end{eqnarray*}

---

References and Quotes for this Spacetime Approach to Electromagnetism

There seem to be some misconceptions on this spacetime formulation.
Here, I have included some quotes from some relativity textbooks that use this spacetime formulation.

 

Robert Geroch’s General Relativity 1972 Lecture Notes (p. 53-54):
An electromagnetic field is a (smooth) antisymmetric tensor field,
$F_{ab} (= F_{[ab]})$, on space-time $M$. An individual observer resolves this
single object – the electromagnetic field – into the separate electric and
magnetic fields seen by him. Our first task is to see how this resolution
comes about. Let our observer have four-velocity $\xi^a$. Recall that
$h_{ab}= g_{ab} + \xi_a\xi_b$ is the projection operator orthogonal to $\xi^a$.
..
To resolve $F_{ab}$ into “spatial tensors” for our observer,
we project the indices of $F_{ab}$ parallel and orthogonal to $\xi^a$.
Thus, we obtain four tensors:
$F_{mn}\xi^m\xi^n$, $F_{mn}\xi^m h^n{}_a$, $F_{mn} h^m{}_a\xi^n$, and $F_{mn} h^m{}_a h^n{}_b$.
Since $F_{ab}$ is antisymmetric, the first vanishes and the second equals minus the third.
The third is called the electric field
$$E^a=F_{ab}\xi^b.\qquad(20)$$
(Note that $F_{am}\xi^m =F_{mn}\xi^m h^n{}_a$.)
Note that the determination of an electric field from the electromagnetic field involves a choice of the observer (i.e., of his four-velocity).
The remaining piece of $F_{ab}$ is $F_{mn}h^m{}_a h^n{}_b$, a spatial, antisymmetric tensor.
…
The spatial vector which gives $F_{mn}h^m{}_a h^n{}_b$
is thus
$$B_a =\frac{1}{2} \epsilon_{abcd} \xi^b F^{cd}.\qquad (21)$$
This $B_a$ is called the magnetic field.
To summarize, the whole story is described by a single tensor field, the electromagnetic field.
An observer resolves this $F_{ab}$ using his four-velocity, into a pair of vectors, $E_a$ and $B_a$, orthogonal to his four-velocity.
The $E_a$ and $B_a$ depend on the observer.
(In terms of components,
the six independent components of $F_{ab}$ become three in $E_a$ and three in $B_a$.)

 

Robert Wald’s General Relativity, (p. 64):
“For an observer moving with 4-velocity $v^a$, the quantity $E_a=F_{ab}v^b$ (4.2.21) is interpreted as the electric field measured by that observer, while
$B_a = -\frac{1}{2} \epsilon_{ab}{}^{cd}F_{cd}v^b$ (4.2.22)
is interpreted as the magnetic field, where $\epsilon_{abcd}$ is the totally antisymmetric tensor of positive orientation with norm
$\epsilon_{abcd}\epsilon^{ abcd}=-24$ (see appendix B) so that in a right-handed orthonormal basis we have $\epsilon_{0123}=1$. “

 

Sachs & Wu General Relativity for Mathematicians (p. 75):
“Let $F$ be an electromagnetic field on $M$, and $(z, Z)$ be an instantaneous observer;
…
Since $F$ is antisymmetric, $\tilde FZ \in Z^\bot$. $E = \tilde FZ$ is defined as the *electric vector* $(z, Z)$ *measures for* $F$. It is kosher to imagine $(z, Z)$ measuring the
electric vector in $Z^\bot$ by essentially Newtonian methods–for example, using
a “test charge” (Alonso-Finn [2]). Now by exterior algebra there is a unique
vector $B\in Z^\bot$ such that
$4! \Omega(X, Y, B, Z) = F(X, Y), \ \forall X,Y \in Z^\bot$,
where $\Omega$ is the metric volume element (cf. the discussion of Hodge duality in Bishop-Goldberg). $B$ is defined as the *magnetic vector* $(z, Z)$ *measures for* $F$.”

 

DeFelice Relativity on Curved Manifolds Eq 9.9.4 (p.299):
Let us now define (Misner et al., 1973):
$E^j = F^j{}_r u^r$; (9.9.4)
from the skew-symmetry of $F^i{}_j$ it follows that:
$E^j u_j=0 \qquad h^i{}_j E^j = E^i$ . (9.9.5)

 

See also (implicitly) Misner, Thorne,& Wheeler Box 3.1(p.72) and Ex 3.6 (p.78).

These are spacetime-formulations (as opposed to 3-vector formulations in a spatial slice) of the “observer-dependent electric and magnetic parts” of the electromagnetic field.

 

---

Comment Thread