The Extended Riemann Hypothesis and Ramanujan’s Sum
Table of Contents
Riemann Hypothesis and Ramanujan’s Sum Explanation
- RH: All non-trivial zeros of the Riemannian zeta-function lie on the critical line.
- ERH: All zeros of L-functions to complex Dirichlet characters of finite cyclic groups within the critical strip lie on the critical line.
- Related Article: The History and Importance of the Riemann Hypothesis
The goal of this article is to provide the definitions and theorems that are necessary to understand these two Riemann hypotheses, i.e. why is a strip in the complex plane called critical, what are trivial and non-trivial zeros, and what is a group character, etc. We will gather a couple of facts around the functions involved, in particular several functional equations.
The extended Riemann hypothesis is a generalization of the Riemann hypothesis that became important when modern computer science began to use number-theoretical results for encryption mechanisms. We will elaborate on these relations to applied mathematics in another article. The main subject of this article will be the Riemann zeta function which is sort of a special case of an L-function and what we and most other people mean if we briefly say zeta-function or ##\zeta##-function.
Zeta Functions
List
- Airy zeta function, related to the zeros of the Airy function
- Arakawa–Kaneko zeta function
- Arithmetic zeta function
- Artin–Mazur zeta function of a dynamical system
- Barnes zeta function or double zeta function
- Beurling zeta function of Beurling generalized primes
- Dedekind zeta function of a number field
- Duursma zeta function of error-correcting codes
- Epstein zeta function of a quadratic form
- Goss zeta function of a function field
- Hasse–Weil zeta function of a variety
- Height zeta function of a variety
- Hurwitz zeta function, a generalization of the Riemann zeta function
- Igusa zeta function
- Ihara zeta function of a graph
- Jacobi zeta function
- L-function, a “twisted” zeta function
- Lefschetz zeta function of a morphism
- Lerch zeta function, a generalization of the Riemann zeta function
- Local zeta function of a characteristic-p variety
- Matsumoto zeta function
- Minakshisundaram–Pleijel zeta function of a Laplacian
- Motivic zeta function of a motive
- Multiple zeta function, or Mordell–Tornheim zeta function of several variables
- p-adic zeta function of a p-adic number
- Prime zeta function, like the Riemann zeta function, but only summed over primes
- Riemann zeta function, the archetypal example
- Ruelle zeta function
- Selberg zeta function of a Riemann surface
- Shimizu L-function
- Shintani zeta function
- Subgroup zeta function
- Weierstraß zeta function
- Witten zeta function of a Lie group
- Zeta function of an incidence algebra, a function that maps every interval of a poset to the constant value 1. Despite not resembling a holomorphic function, the special case for the poset of integer divisibility is related as a formal Dirichlet series to the Riemann zeta function.
- Zeta function of an operator or spectral zeta function
Definitions
\begin{align*}
\zeta(s)&:=\sum_{n=1}^\infty \dfrac{1}{n^s}=1+\dfrac{1}{2^s}+\dfrac{1}{3^s}+\dfrac{1}{4^s}+\dfrac{1}{5^s}+\cdots = \prod_{p\text{ prime}}\dfrac{1}{1-p^{-s}}\quad (s\in \mathbb{C})\\
\Gamma(z)&:=\lim_{n \to \infty}\dfrac{n!n^z}{z\cdot (z+1)\cdot \ldots\cdot (z+n)}=\int_0^{\infty }t^{z-1}e^{-t}\,dt\\[6pt]
\Gamma(0)&=1\, , \,\Gamma(1)=1\, , \,\Gamma(n)=(n-1)!\, , \,\Gamma(1/2)=\sqrt{\pi}\, , \,\Gamma(z+1)=z\Gamma(z)
\end{align*}
Proof
Let ##\mathbb{P}## be the set of all primes and ##p\in \mathbb{P}.## Then
\begin{align*}
\left(1-\dfrac{1}{p^s}\right)\zeta(s)&=\sum_{n=1}^\infty \dfrac{1}{n^s}-\sum_{n=1}^\infty \dfrac{1}{(pn)^s} =\sum_{\stackrel{n=1}{n\not\in p\mathbb{Z}}}^\infty \dfrac{1}{n^s}
=\sum_{\stackrel{n=1}{p\nmid n}}^\infty \dfrac{1}{n^s}\\
\prod_{p\in\mathbb{P}}\left(1-\dfrac{1}{p^s}\right)\zeta(s)&=\sum_{\stackrel{n=1}{n\not\equiv 0\mod p\,\forall \,p\in \mathbb{P}}}^\infty \dfrac{1}{n^s}=\sum_{n\in \{1\}}\dfrac{1}{n^s}=1\\
\zeta(s)&=1/\prod_{p\in\mathbb{P}}\left(1-\dfrac{1}{p^s}\right)=
\prod_{p\in\mathbb{P}}\left(1/\left(1-\dfrac{1}{p^s}\right)\right)=\prod_{p\in\mathbb{P}}\dfrac{1}{1-p^{-s}}
\end{align*}
From ##e^{-t}=\displaystyle{\lim_{n \to \infty}}\left(1-\dfrac{t}{n}\right)^n## we get
\begin{align*}
\int_0^\infty e^{-t}\,t^{z-1}\,dt&=\lim_{n \to \infty}\int_0^n\left(1-\dfrac{t}{n}\right)^nt^{z-1}\,dt\\
&\stackrel{t=ns}{=}\lim_{n \to \infty}\int_0^1 (1-s)^nn^{z}s^{z-1}\,ds\\
&=\lim_{n \to \infty}n^z \left(\left[\dfrac{s^z}{z}(1-s)^{n}\right]_0^1 +\dfrac{n}{z}\int_0^1 (1-s)^{n-1}s^z\,ds\right)\\
&\quad \vdots \\
&=\lim_{n \to \infty}n^z\left(\dfrac{n}{z}\cdot \dfrac{n-1}{z+1}\cdot\ldots\cdot \dfrac{1}{z+n-1}\int_0^1s^{z+n-1}\,ds\right)\\
&=\Gamma(z)
\end{align*}
\begin{align*}
\Gamma(z+1)&=\int_0^{\infty }t^{z}e^{-t}\,dt=-\left[t^ze^{-t}\right]_0^\infty + z\int_0^{\infty }t^{z-1}e^{-t}\,dt=z\Gamma(z)
\end{align*}
The particular values of the ##\Gamma##-function are obvious except for ##\Gamma(1/2).##
\begin{align*}
\left(\int_\mathbb{R}e^{-t^2}\,dt\right)^2&=\left(\int_\mathbb{R}e^{-x^2}\,dx \right)\cdot \left(\int_\mathbb{R}e^{-y^2}\,dy \right)=\int_\mathbb{R}\int_\mathbb{R}e^{-x^2-y^2}\,dx\,dy\\
&=\int_0^{\infty}\int_0^{2\pi}re^{-r^2}\,d\varphi \,dr=2\pi \int_0^\infty re^{-r^2}\,dr=-\pi\left[e^{-r^2}\right]_0^\infty =\pi
\end{align*}
Hence
$$
\Gamma(1/2)=\int_0^\infty t^{-1/2}e^{-t}\,dt=2\int_0^\infty e^{-r^2}\,dr=\int_\mathbb{R} e^{-r^2}\,dr= \sqrt{\pi}.
$$
We will need the important functional equation for the zeta-function (Riemann, 1859) which we will prove next. The proof requires some preparations.
Poisson Summation Formula
Let ##f\, : \,\mathbb{R}\longrightarrow \mathbb{C}## be a continuously differentiable function with ##f(x)=O(|x|^{-2})## for ##|x|\to \infty .## Let ##\hat{f}\, : \,\mathbb{R}\longrightarrow \mathbb{C}## be the Fourier transform of ##f,## i.e.
$$
\hat{f}(t)=\int_{-\infty }^{\infty }f(x)e^{-2\pi i x t}\,dx.
$$
Then $$
\sum_{n\in \mathbb{Z}} f(n)=\sum_{n\in \mathbb{Z}}\hat{f}(n).
$$
Furthermore, we get for ##\lambda >0## and ##f_\lambda (x):=f(\lambda x)##
$$
\hat{f_\lambda }(t)=\dfrac{1}{\lambda }\hat{f}\left(\dfrac{t}{\lambda }\right).
$$
Proof
##F(x):=\sum_{n\in \mathbb{Z}} f(x+n) \, : \,\mathbb{R}\longrightarrow \mathbb{C}## is a continuously differentiable function with period ##1## and can as such be developed into a Fourier-series
$$
F(x)=\sum_{n\in \mathbb{Z}} c_ne^{2\pi i n x}.
$$
This series converges uniformly since ##F## is continuously differentiable. Therefore
\begin{align*}
c_n &=\int_0^1 F(x)e^{-2\pi i n x}\,dx=\sum_{k=-\infty }^\infty \int_0^1 f(x+k)e^{-2\pi i n x}\,dx \\
&=\sum_{k=-\infty }^\infty \int_{k}^{k+1}f(x)e^{-2\pi i n x}\,dx=\int_{-\infty }^\infty f(x)e^{-2\pi i n x}\,dx=\hat{f}(n)
\end{align*}
Thus
$$
\sum_{n\in \mathbb{Z}}f(x+n)=\sum_{n\in \mathbb{Z}}\hat{f}(n)e^{-2\pi i n x}
$$
and the statement follows for ##x=0.## The formula for ##\hat{f_\lambda }## follows from
\begin{align*}
\hat{f_\lambda }(t)&=\int_{-\infty }^\infty f(\lambda x)e^{-2\pi i x t}\,dx=\frac{1}{\lambda }\int_{-\infty }^\infty f(\lambda x)e^{-2\pi i (\lambda x) \frac{t}{\lambda }}\,d(\lambda x)=\frac{1}{\lambda }\hat{f}\left(\frac{t}{\lambda }\right)
\end{align*}
Example
The Fourier transform of ##f(x):=e^{-\pi x^2}## is ##\hat{f}(t)=\displaystyle{\int_{-\infty }^\infty e^{-\pi x^2}e^{-2\pi i x t}\,dx}=f(t).##
Proof
\begin{align*}
\hat{f}(0)&= \int_{-\infty }^\infty e^{-\pi x^2}\,dx=\dfrac{1}{\sqrt{\pi}} \int_\mathbb{R}e^{-y^2}\,dy\stackrel{(s.a.)}{=}\dfrac{\Gamma(1/2)}{\sqrt{\pi}}=1\\
\hat{f}(t)&=e^{-\pi t^2}\int_\mathbb{R}e^{\pi t^2 -2\pi i x t -\pi x^2}\,dx=e^{-\pi t^2}\int_\mathbb{R}e^{-\pi(x+it)^2}\,dx\\
&\stackrel{(s=x+it)}{=}e^{-\pi t^2}\int_\mathbb{R}e^{-\pi s^2}\,ds=e^{-\pi t^2}\hat{f}(0)=e^{-\pi t^2}=f(t)
\end{align*}
In order to see why the substitution works, we apply Cauchy’s integral theorem on ##z\longmapsto e^{-\pi z^2}## along the closed integration path

\begin{align*}
\int_{-R}^{R}e^{-\pi(x+ i t)^2}\,dx&=\int_{-R+it}^{R+it}e^{-\pi z^2}\,dz= \underbrace{\int_{-R+it}^{-R}e^{-\pi z^2}\,dz}_{\to 0 \text{ for }R\to \infty} + \int_{-R}^{R}e^{-\pi s^2}\,ds + \underbrace{\int_{R}^{R+it}e^{-\pi z^2}\,dz}_{\to 0 \text{ for }R\to \infty}
\end{align*}
The classical Jacobian theta-function is defined as ##\vartheta (z,\tau ):=\sum_{n= -\infty }^{\infty }e^{\pi i n^{2}\tau +2\pi inz}.## The series is convergent in ##\mathbb {C} \times \mathbb {H} ## where ##\mathbb {H} =\{x\in \mathbb{C} \mid \mathfrak{I(\tau)}>0\}.## We are interested in the theta-function with a fixed value ##z## such that ##\vartheta(z,\cdot)## is a holomorphic function on ##\mathbb{H}.## Let ##z= 0## and ##\theta\, : \,\mathbb{R}^+\longrightarrow \mathbb{C}##
$$
\theta(x):=\sum_{n= -\infty }^{\infty }e^{-\pi n^{2}x}
$$
Functional Equation of the Theta-Series
\begin{align*}
\theta(x)&=\dfrac{1}{\sqrt{x}}\;\theta\left(\dfrac{1}{x}\right)\; \text{ for all } x>0\;\text{ and }\; \theta(x)=O(1/\sqrt{x}\,)\,\text{ for }x \searrow 0
\end{align*}
Proof
We have ##\hat{f_1}(x)=f_1(x)## for ##f_1\, : \,\mathbb{R}\longrightarrow \mathbb{C}\, , \,f_1(x):=e^{-\pi x^2}## by the example above. For ##\lambda >0## we get with ##f_\lambda (x):=e^{-\pi x^2\lambda }##
$$
\hat{f_\lambda }(x)=\dfrac{1}{\sqrt{\lambda }}\hat{f}\left(\dfrac{x}{\lambda }\right)= \dfrac{1}{\sqrt{\lambda }}e^{-\pi x^2/\lambda }
$$
such that the Poisson summation with ##f=f_1## yields
$$
\theta(\lambda )=\sum_{n\in \mathbb{Z}}e^{-\pi n^2\lambda }=\sum_{n\in \mathbb{Z}}f_\lambda (n)=\sum_{n\in \mathbb{Z}}\hat{f_\lambda}(n)=\dfrac{1}{\sqrt{\lambda }}\sum_{n\in \mathbb{Z}}e^{-\pi n^2/\lambda }=\dfrac{1}{\sqrt{\lambda }}\;\theta\left(\dfrac{1}{\lambda }\right)
$$
Finally, ##\lim_{\lambda \to \infty}\theta(\lambda )=1,## i.e. with ##x=1/\lambda ##
$$
\lim_{x\searrow 0}\theta(x)=\lim_{\lambda\to\infty}\theta(1/\lambda )=\lim_{\lambda\to\infty}\sqrt{\lambda }\;\theta(\lambda )
=\lim_{\lambda\to\infty}\sqrt{\lambda }=\lim_{x \searrow 0}1/\sqrt{x}.
$$
Supplementary Theorem of the Gamma Function, Euler 1749
$$
\Gamma(z)\Gamma(1-z) = \dfrac{\pi}{\sin \pi z}\;\text{ for all }z\in \mathbb{C}-\mathbb{Z}
$$
Proof
$$
\phi(z):=\Gamma(z)\Gamma(1-z)=\dfrac{\Gamma(1+z)}{z}\cdot \dfrac{\Gamma(2-z)}{1-z}
$$
is meromorphic on ##\mathbb{C}## with first order singularities in ##z=n\in \mathbb{Z},## and bounded on ##S_1:=\{z\in \mathbb{C}\,|\,0\leq \mathfrak{R}(z)\leq 1\text{ and }|\mathfrak{I}(z)|\geq 1\}.## Furthermore, let
$$
\psi(z):=\sin(\pi z)\phi(z)=\sin(\pi z)\Gamma(z)\Gamma(1-z)
$$
that is holomorphic everywhere because ##\sin(\pi n)=0## with first order zeros.
\begin{align*}
\phi(z+1)&=\phi(-z)=-\dfrac{\Gamma(1-z)}{z}\cdot \dfrac{\Gamma(2+z)}{1+z}=-\dfrac{\Gamma(1-z)}{z}\cdot \dfrac{z\Gamma(z)(z+1)}{1+z}\\
&=-\Gamma(1-z)\Gamma(z)=-\phi(z)\\[12pt]
\psi(z+1)&=\sin(\pi(z+1))\phi(z+1)=\sin(\pi z)\phi(z)=\psi(z)
\end{align*}
Thus ##|\psi(z)|\leq |\phi(z)|\leq Ce^{\pi|z|}\;(*)## for all ##z\in \mathbb{C}## since ##\phi(z)## is bounded on ##S_1.## However, ##\psi(z)\neq 0## everywhere, hence ##\psi(z)=e^{g(z)}## for some on ##\mathbb{C}## holomorphic function ##g(z).## This means, given ##(*),## that ##g(z)=a+bz## for some constants ##a,b\in \mathbb{C}.## It follows that ##b=0## because ##\psi(-z)=\sin(-\pi z)\phi(-z)=\sin(\pi z)\phi(z)=\psi(z)## and ##\psi(z)## is constant. From
$$
\psi(z)=\dfrac{\sin(\pi z)}{z} \Gamma(z+1)\Gamma(1-z)
$$
we get ##\psi(0)=\pi## which proves the statement, and provides a second proof for ##\Gamma(1/2) = \sqrt{\pi}.##
Legendre’s Doubling Formula, 1809
$$
\Gamma\left(\dfrac{z}{2}\right)\cdot\Gamma\left(\dfrac{z+1}{2}\right)=\dfrac{\sqrt{\pi}}{2^{z-1}}\cdot \Gamma(z)\;\text{ for all }z\in \mathbb{C}-\{0,-1,-2,\ldots\}
$$
Proof
Set ##G(z):=2^z\Gamma\left(\dfrac{z}{2}\right)\Gamma\left(\dfrac{z+1}{2}\right).## Then
\begin{align*}
G(z+1)&=2^{z+1}\Gamma\left(\dfrac{z+1}{2}\right)\Gamma\left(\dfrac{z}{2}+1\right)=2^{z+1}\Gamma\left(\dfrac{z+1}{2}\right)\dfrac{z}{2}\Gamma\left(\dfrac{z}{2}\right)=zG(z)
\end{align*}
By the theorem of Bohr-Mollerup, we get ##G(z) = G(1) \cdot \Gamma(z) = 2\sqrt{\pi}\;\Gamma(z).##
Cp. first problem in September 2021.
Alternatively, consider with ##x=z/2## (in order to avoid too many powers of ##z/2##)
\begin{align*}
2^z\Gamma\left(\dfrac{z}{2}\right)\Gamma\left(\dfrac{z+1}{2}\right)&=
\lim_{n \to \infty}\dfrac{2^{2x} n^xn!n^{x+1/2}n!}{x(x+1)\cdots(x+n)(x+1/2)(x+3/2)\cdots(x+n+1/2)}\\[6pt]
&=\lim_{n \to \infty} \dfrac{2^{z+2n+2}(n!)^2n^{z+1/2}}{z(z+2)\cdots(z+2n)(z+1)(z+3)\cdots(z+2n+1)}\\[6pt]
&=\lim_{n \to \infty}\dfrac{2^{2(n+1)}(n!)^2n^{1/2}}{(2n+1)!}\cdot\dfrac{(2n+1)^z(2n+1)!}{z(z+1)\cdots(z+2n+1)}\cdot\left(\dfrac{2n}{2n+1}\right)^z\\[6pt]
&=\lim_{n \to \infty}\dfrac{2^{2(n+1)}(n!)^2n^{1/2}}{(2n)!}\cdot \Gamma(z)
\end{align*}
From ##z=1## we get ##2\Gamma(1/2)\Gamma(1)=2\sqrt{\pi}=\lim_{n \to \infty}\dfrac{2^{2(n+1)}(n!)^2n^{1/2}}{(2n)!}\cdot\Gamma(1)## and
$$
2^{z-1}\Gamma\left(\dfrac{z}{2}\right)\Gamma\left(\dfrac{z+1}{2}\right)=\sqrt{\pi}\cdot\Gamma(z)
$$
The Legendre doubling formula is a special case of (without proof)
Gauß’s Multiplication Formula, 1812
\begin{align*}
\Gamma\left(\dfrac{z}{n}\right)\cdot\Gamma\left(\dfrac{z+1}{n}\right)\cdots\Gamma\left(\dfrac{z+n-1}{n}\right)&=\dfrac{(2\pi)^{(n-1)/2}}{n^{(z-1/2)}}\cdot \Gamma(z)\\[6pt]
\text{for }n\in \mathbb{N},z\in \mathbb{C}-\{0,-1,-2\ldots\}&
\end{align*}
Gregory Chudnovsky has shown in 1975, that every number ##\Gamma (1/6)##, ##\Gamma (1/4)##, ##\Gamma (1/3)##, ##\Gamma (2/3)##, ##\Gamma (3/4)## and ## \Gamma (5/6)## is transcendent and algebraically independent of ##\pi.## On the other hand, it is unknown whether ##\Gamma(1/5)## is even irrational.
##\rho(t):=\displaystyle{\sum_{n=1}^\infty e^{-\pi n^2 t}= \dfrac{1}{2}(\theta(t)-1) \stackrel{t\searrow 0}{\longrightarrow }O\left(1/\sqrt{t}\right)}.## Thus the following integral exists, and for ##s\in \mathbb{C}## with ##\mathfrak{R}(s)>1,## we get (##t=\pi n^2 u##)
\begin{align*}
\Gamma\left(\dfrac{s}{2}\right)\zeta(s)&= \sum_{n=1}^\infty \Gamma\left(\dfrac{s}{2}\right) \dfrac{1}{n^s}
=\sum_{n=1}^\infty \dfrac{1}{n^s}\int_0^\infty t^{s/2}e^{-t}\dfrac{dt}{t}= \pi^{s/2} \int_0^\infty u^{s/2}\sum_{n=1}^\infty\left(e^{-\pi n^2 u}\right)\dfrac{du}{u}.
\end{align*}
Finally, we can prove the
Functional Equation of the ##\zeta##-Function
Set ##\xi(s):=\pi^{-s/2}\Gamma(s/2)\zeta(s).## This at prior on the half plane ##\{\mathfrak{R}(s)>1\}## holomorphic function can be extended to a function that is meromorphic on the entire complex plane. The extended function has first order singularities at ##s\in \{0,1\},## and is holomorphic elsewhere. Moreover,
$$
\xi(s)=\xi(1-s).
$$
The ##\zeta##-function can therefore be meromorphic extended on ##\mathbb{C}## with a single pole at ##s=1.## Moreover,
$$
\zeta(1-s)=2(2\pi)^{-s}\Gamma(s)\cos\left(\dfrac{\pi s}{2}\right)\zeta(s).
$$
Proof (Riemann)
Assuming the first part, we get
\begin{align*}
\zeta(s-1)&=\pi^{(1-s)/2}\Gamma\left(\dfrac{1-s}{2}\right)^{-1}\xi(1-s)=\pi^{(1-s)/2}\Gamma\left(\dfrac{1-s}{2}\right)^{-1}\pi^{-s/2}\Gamma\left(\dfrac{s}{2}\right)\zeta(s)\\
&=\pi^{-s}\sqrt{\pi}\;\Gamma\left(\dfrac{1-s}{2}\right)^{-1}\Gamma\left(\dfrac{s}{2}\right)\zeta(s)\\
&=\pi^{-s}\sqrt{\pi}\;\dfrac{\sin\left(\dfrac{\pi(1-s)}{2}\right)}{\pi}\Gamma\left(\dfrac{1+s}{2}\right)\Gamma\left(\dfrac{s}{2}\right)\zeta(s)\\
&=\pi^{-s}\cos\left(\dfrac{\pi s}{2}\right)\dfrac{1}{2^{s-1}}\Gamma(s)\zeta(s)
\end{align*}
We get from the previous remark that
$$
\xi(s) = \pi^{-s/2}\Gamma(s/2)\zeta(s)=\int_0^\infty t^{s/2}\underbrace{\left(\sum_{n=1}^\infty e^{-\pi n^2t}\right)}_{=\rho(t)=(1/2)(\theta(t)-1)}\dfrac{dt}{t}\quad (\mathfrak{R}(s)>1)
$$
Observe that
$$
\rho\left(\dfrac{1}{t}\right)=\dfrac{1}{2}\left(\theta\left(\dfrac{1}{t}\right)-1\right)=\dfrac{1}{2}\left(\sqrt{t}\;\theta(t)-1\right)=\sqrt{t}\;\rho(t)-\dfrac{1}{2}+\dfrac{1}{2}\sqrt{t}
$$
Then
\begin{align*}
\int_0^1 t^{s/2}\rho(t)\dfrac{dt}{t}&=\int_0^1 t^{s/2}\left(\dfrac{1}{\sqrt{t}}\;\rho\left(\dfrac{1}{t}\right)+\dfrac{1}{2\sqrt{t}}-\dfrac{1}{2}\right)\dfrac{dt}{t}\\
&=\int_0^1 t^{(s-1)/2}\rho\left(\dfrac{1}{t}\right)\dfrac{dt}{t}+\dfrac{1}{2}\int_0^1 (t^{(s-1)/2}-t^{s/2})\dfrac{dt}{t}\\
&=\int_1^\infty u^{(1-s)/2}\rho(u)\dfrac{du}{u}+\dfrac{1}{s-1}-\dfrac{1}{s}\\
\xi(s)&=\int_0^1t^{s/2}\rho(t)\dfrac{dt}{t}+\int_1^\infty t^{s/2}\rho(t)\dfrac{dt}{t}\\
&= \dfrac{1}{s-1}-\dfrac{1}{s}+\int_1^\infty \left(t^{s/2}+t^{(1-s)/2}\right) \rho(t) \dfrac{dt}{t}
\end{align*}
##\rho(t)## tends exponentially toward ##0## with increasing ##t,## i.e. the integral exists for all ##s\in \mathbb{C}.## It is a holomorphic function in ##s.## Thus, ##\xi(s)## is meromorphic extended. The last representation of ##\xi(s)## is invariant under ##s \mapsto 1-s,## which proves the statement.
Zeros and Poles of the ##\zeta##-Function
We have just proven that the only pole of the ##\zeta##-Function is at ##x=1.##
To consider the zeros, we will distinguish between three cases.
- ##\mathfrak{R}(s)>1.##
In this case, we conclude by the product formula that
$$
\zeta(s)=\prod_{p \text{ prime}}\dfrac{1}{1-p^{-s}} \neq 0
$$ - ##\mathfrak{R}(s)<0.##
In this case, we have ##\mathfrak{R}(1-s)>1##, and the functional equation
$$
\zeta(s)=2(2\pi)^{s-1}\Gamma(1-s)\cos\left(\dfrac{\pi (1-s)}{2}\right)\zeta(1-s)=0
$$
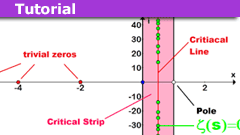
if and only if the cosine becomes zero because ##\mathfrak{R}(1-s)>1.## However, the complex cosine has only real zeros at ##(2k+1)\pi/2.## Therefore ##1-s=2k+1## or ##\zeta(s)=\zeta(-2k)=0## if and only if ##k\in \{1,2,3,\ldots\}.##The zeros ##-2,-4,-6,\ldots## are called trivial zeros of the ##\zeta##-function. - ##0\leq \mathfrak{R}(s)\leq 1.##
This area of the complex number plane is called the critical strip. All remaining zeros have to be within the critical strip. We know that if ##\zeta(a+ib)=0## then ##\zeta(1-a-ib)=0,## i.e. possible zeros occur mirrored at ##\mathfrak{R}(s)=1/2,## which we call the critical line, and mirrored at the real axis. All ##10^{13}## zeros that have been found so far lie on the critical line.
The Riemann hypothesis says, that all non-trivial zeros of the Riemann zeta function lie on the critical line.

The Extended Riemann Hypothesis
A complex Dirichlet character of a finite cyclic group is a group homomorphism ##\chi: \left(\mathbb{Z}_n\right)^\times \rightarrow \mathbb{C}^\times## into the multiplicative group of the complex numbers
\begin{align*}
\chi\, : \,\{a \mod n\,|\,(a,n)=1\}&\longrightarrow \mathbb{C}-\{0\}\\
\chi(a)\cdot \chi(b)=\chi(a\cdot b)
\end{align*}
where ##(a,n)## denotes the greatest common divisor of ##a## and ##n.## We associate with it a periodic function modulo ##n## by
\begin{align*}
\chi\, &: \,\mathbb{Z} \longrightarrow \mathbb{C}\\
\chi(a)=&\begin{cases}
\chi(a) &\text{ if }(a,n)=1\\
0 &\text{ if }(a,n)>1 \end{cases}
\end{align*}
which we also call Dirichlet character modulo n. They play a key role in Dirichlet’s theorem on arithmetic progressions:
For any two positive coprime integers ##a## and ##d## there are infinitely many primes of the form ##a + nd.##
Examples are the trivial character ##\chi_0(a)=1## for all ##(a,n)=1,## and the Jacobi-character
$$
\chi(a)=\left(\dfrac{a}{p}\right)=
\begin{cases}
1 &\text{ if } a\not\equiv 0 \mod p \text{ and there is an integer x such that}a\equiv x^2 (p)\\
-1 &\text{ if }a\not\equiv 0 \mod p \text{ and there is no such x}\\
0 &\text{ if }a\equiv 0 \mod p
\end{cases}
$$
extended to a homomorphism for ##n=\prod p## (cp. Jacobi symbol). Every Dirichlet character has a (Dirichlet) ##L##-function, or ##L##-series defined by
$$
L_\chi(z):=\sum_{a=1}^\infty \dfrac{\chi(a)}{a^z}
$$
Dirichlet series converge absolutely and locally uniform in the half-plane ##\{\mathfrak{R}(z)>1\}## since
$$
\left|\dfrac{\chi(a)}{a^z}\right|=\left|\dfrac{\chi(a)}{a^{\mathfrak{R}(z)} \cdot e^{ i \log(a) \mathfrak{I}(z) }} \right|=\dfrac{1}{a^{\mathfrak{R}(z)}} \text{ or } 0
$$
and the homomorphism property forces ##\chi(a)## to be a root of unity (or ##0##). ##L##-series can be holomorphic extended on ##\{\mathfrak{R}(z)>0\},## except for the trivial character, which has a first order pole at ##z=1.## The Dirichlet function ##L_\chi(z)## has the Riemann property, if all zeros within the critical strip are already on the critical line ##\{\mathfrak{R}(z)=\frac{1}{2}\}##.
The extended Riemann hypothesis says that all zeros of all Dirichlet L-functions to complex Dirichlet characters of finite cyclic groups within the critical strip lie already on the critical line.
We get for the trivial character ##\chi_0## modulo ##n##
$$
L_{\chi_0}(z)=\sum_{(a,n)=1}\dfrac{1}{a^z}=\zeta(z)\cdot \prod_{p|n\text{ prime}}\left(1-\dfrac{1}{p^z}\right)
$$
which has exactly the same zeros in ##\{\mathfrak{R}(z)>0\}## as the ##\zeta##-function. The extended Riemann hypothesis thus implies the ordinary Riemann hypothesis.
Srinivasa Ramanujan, 1887-1920

“The divergent series are the invention of the devil, and it is a shame to base on them any demonstration whatsoever.” (Niels Henrik Abel, 1832)
$$
\dfrac{1}{1^2}+\dfrac{1}{2^2}+\dfrac{1}{3^2}+\dfrac{1}{4^2}+\ldots=\dfrac{\pi^2}{6}=\zeta(2)\quad \text{(Euler)}
$$
Hence
$$
1+2+3+\ldots=\zeta(-1)=\zeta(1-2)=2(2\pi)^{-2}\Gamma(2)\cos\left(\dfrac{2\pi}{2}\right)\zeta(2)=-\dfrac{1}{12}
$$
Sources
[1] Die erweiterte Riemannsche Vermutung (ERH)
https://www.staff.uni-mainz.de/pommeren/Kryptologie/Asymmetrisch/3_Prim/erh.pdf
[2] Analytische Zahlentheorie, Sommersemester 2007, Prof. Dr. Helmut Maier, Dipl.-Math. Dipl.-Inform. Daniel Haase
https://www.uni-ulm.de/fileadmin/website_uni_ulm/mawi.inst.zawa/lehre/12sem-pz/Analytische_Zahlentheorie_SS_2007.pdf
[3] On Riemann’s Paper, “On the Number of Primes Less Than a Given Magnitude”, W. Dittrich, Institut für Theoretische Physik, Universität Tübingen, August 3, 2017
https://arxiv.org/pdf/1609.02301.pdf
[4] On the Number of Prime Numbers less than a Given Quantity.
(Ueber die Anzahl der Primzahlen unter einer gegebenen Grösse.), Bernhard Riemann, Translated by David R. Wilkins, Preliminary Version: December 1998, [Monatsberichte der Berliner Akademie, November 1859.]
https://www.claymath.org/sites/default/files/ezeta.pdf
[5] B Candelpergher. Ramanujan summation of divergent series. Lectures notes in mathematics, 2185, 2017. ffhal-01150208v2f
https://hal.univ-cotedazur.fr/hal-01150208v2/document
[6] Frank Thorne, “1+2+3+4+…”, University of South Carolina, Dartmouth College, May 9, 2013
https://people.math.sc.edu/thornef/dartmouth.pdf
[7] Die Riemannsche Zetafunktion, Bakkalaureatsarbeit, Technische Universität Wien, 28. September 2012, Armand Nabavi
https://www.asc.tuwien.ac.at/~herfort/BAKK/Nabavi_Riemann_Zeta_Funktion.pdf
[8]Otto Forster, München, 6. Die Gamma-Funktion
https://www.mathematik.uni-muenchen.de/~forster/v/zeta/RZF_chap06.pdf
[9] Otto Forster, München, 7. Funktionalgleichung der Zeta-Funktion
https://www.mathematik.uni-muenchen.de/~forster/v/zeta/RZF_chap07.pdf
[10] Wikipedia
https://en.wikipedia.org/wiki/Main_Page
https://de.wikipedia.org/wiki/Wikipedia:Hauptseite









Best of luck to all those trying to solve it!
"
"
If I were to awaken after having slept for a thousand years, my first question would be: Has the Riemann hypothesis been proven?
"
"Mathematical Mysteries: The Beauty and Magic of Numbers". Book by Calvin C. Clawson, p. 258, 1999.