- #1
PRODOS
- 7
- 0
In the double slit experiment, Bohmian Mechanics http://plato.stanford.edu/entries/qm-bohm/#2s" the paths of real particles traveling from the two slits to the detector to look like something like this:
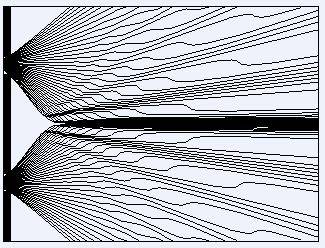
The above image shows particles traveling in non-straight paths.
The diagram below represents the double slit experiment.
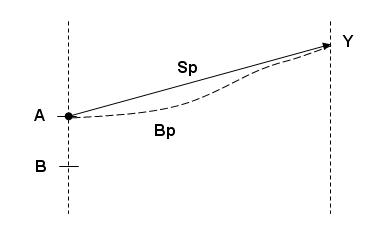
The two slits are at points A and B.
A hypothetical real photon goes through the slit at A and is detected at point Y on the detector screen.
Sp is a straight line from A to Y.
Represent its length as “s”.
Bp is a non-straight line from A to Y.
Represent its length as “b”.
b > s
If the time it takes a photon to leave A and be detected at Y could be measured, and ...
If this was found to be:
s/c
(c is the speed of light)
Then would it be reasonable to conclude the following about the hypothetical real photon?
Either:
Or:
I would appreciate comments and corrections regarding this hypothetical real photon scenario.
Thanks.
The above image shows particles traveling in non-straight paths.
The diagram below represents the double slit experiment.
The two slits are at points A and B.
A hypothetical real photon goes through the slit at A and is detected at point Y on the detector screen.
Sp is a straight line from A to Y.
Represent its length as “s”.
Bp is a non-straight line from A to Y.
Represent its length as “b”.
b > s
If the time it takes a photon to leave A and be detected at Y could be measured, and ...
If this was found to be:
s/c
(c is the speed of light)
Then would it be reasonable to conclude the following about the hypothetical real photon?
Either:
- The photon has in fact traveled in a straight line from A to Y - rather than in a non-straight line as depicted by Bohminan Mechanics
Or:
- The photon has at some point along its trajectory traveled faster than the speed of light
I would appreciate comments and corrections regarding this hypothetical real photon scenario.
Thanks.
Last edited by a moderator: