- #1
mattmannmf
- 172
- 0
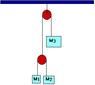
3 blocks are hanging on pulleys:
O
| \
| \
M3 \
\
O
| \
| \
M1 M2
o= pulleysthe masses of 3 blocks are M1 = 12 kg, M2 = 18 kg, and M3 = 30 kg. The pulleys and strings are massless, there is no friction, and gravity points downward. The whole system is held fixed, then released at rest. What is the acceleration of the 30 kg block? Enter a positive answer if it goes up, a negative answer if it goes down, or 0 if the acceleration is zero.
so pretty much the 30 kg mass is attatched to a pulley which is attached to another pulley which is held by the the 2 masses of 12 kg and 18 kg.
As far as doing this problems I know that I need to use the formula:
T-mg=ma
and to figure out the acceleration of the M1 and M2 I used this:
a= (m2 −m1)g/ (m1 + m2)
which i then got a= 1.96 m/s^2
which (plugging into the above equation using M1) i got tension to be 141.12 N
Also noted by this equation:
T= 2(M1M2)g / (m1 +m2)
Now this is where i get stuck. I don't know where to go from there. I got the tension for the 2 pulleys and also the acceleration but how do I apply that to the next pulley which is attatched to M3 (30 kg) which will get my acceleration of block M3.
O
| \
| \
M3 \
\
O
| \
| \
M1 M2
o= pulleysthe masses of 3 blocks are M1 = 12 kg, M2 = 18 kg, and M3 = 30 kg. The pulleys and strings are massless, there is no friction, and gravity points downward. The whole system is held fixed, then released at rest. What is the acceleration of the 30 kg block? Enter a positive answer if it goes up, a negative answer if it goes down, or 0 if the acceleration is zero.
so pretty much the 30 kg mass is attatched to a pulley which is attached to another pulley which is held by the the 2 masses of 12 kg and 18 kg.
As far as doing this problems I know that I need to use the formula:
T-mg=ma
and to figure out the acceleration of the M1 and M2 I used this:
a= (m2 −m1)g/ (m1 + m2)
which i then got a= 1.96 m/s^2
which (plugging into the above equation using M1) i got tension to be 141.12 N
Also noted by this equation:
T= 2(M1M2)g / (m1 +m2)
Now this is where i get stuck. I don't know where to go from there. I got the tension for the 2 pulleys and also the acceleration but how do I apply that to the next pulley which is attatched to M3 (30 kg) which will get my acceleration of block M3.