Addition and Subtraction of Vectors

[MuchJokes]

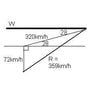
A plane, traveling with a velocity relative to the air of 320km/h [28 S of W], passes over Winnipeg. The wind velocity is 72km/h . Determine the displacement of the plane from Winnipeg 2.0 h later.

Cosine Law -> c^2 = b^2 + a^2 - 2*b*a*Cos<C
Sine Law- (Sin<A)/a = (Sin<B)/b = (Sin<C)/c
Addition and Subtraction of Vectors
West is left, South is down.
1. Drew the thing. It was pretty.
2. Drew a line parallel to West and found part of the really big angle. Then I added 90 to it. = 118 degrees
3. Used Cosine law to get resultant Velocity
4. Used Sine law to get the top little angle. Added it to the existing 28 degrees to get my resultant angle S of W
5. Burst into tears because the answer in the book brutally murdered my already low self-esteem.

 

[lavalamp]

It would help if you show your working out, so that it's possible to determine at what point you made a mistake.

It certainly sounds like you know what you're doing since you're going about it in the right way.

 

[MuchJokes]

Here's what it looks like.

I used to Cosine law to find the resultant Velocity, and then used the sine law. Unfortunately, my answer for the top angle was 10 degrees. Which apparently is wrong.

 

[lavalamp]

I can't look at that attachment until it has been approved by a moderator. Can you put it online somewhere? Any random image host would do.

Edit: I also get 10.19 degrees for the small angle in the triangle. But remember that needs to be added on to the 28 degrees to get the angle south of west.

 

[MuchJokes]

http://img227.imageshack.us/img227/4299/physicswa9.jpg

I know this is against the rules but the book says the answer is 30 degrees S of W

Is this just wrong or something? Or does something change from the transition from velocity to displacement?

 

[lavalamp]

It seems as though the book may just be wrong. However go back and check, double check, triple check the question to make sure there's no information you missed or read incorrectly.

If you can't find anything that changes the answer, you might want to mention this to the teacher. And if it turns out that the answer is actually 30 degrees south of west, make sure you find out what you did wrong, then come back and tell me too.

 

[MuchJokes]

The bok was wrong. Ironically, the answer book which our teacher has in his possesion had a different answer. It had the RIGHT answer.

There went my monday. Thanks for the help.

 

[lavalamp]

Heh, I can sympathise, but at least you got it right. It's better than spending lots of time on it and still getting it wrong.