Bernoulli equation (self made diagram)

[Kaxa2000]

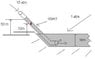
An object is accidentally dropped into a water pipe. Pressure applied to pipe is 10 atm. The depth of lake is 10m. What will be speed of object when it exits the pipe and first enters the lake? Assume that the object is carried along with the surrounding water and does not affect flow of water in any way. Neglect friction.

Diagram is in attachment

I know you have to use Bernoullis equation for this problem. The examples I've looked at usually have a different area at each end. This problem has apparently the same area at each end. Also I don't understand how to factor in the height included in the problem.

 

[Kaxa2000]

.5*density *(0)² + 0 + 10atm(convert) = constant

(.5)(density)(vf)² + density(g)(y height) + 2atm

Would I set these equal and solve for vf??

What about the height of the lake and the height of the pipe?? Do those add more pressure??