- #1
Beamsbox
- 61
- 0
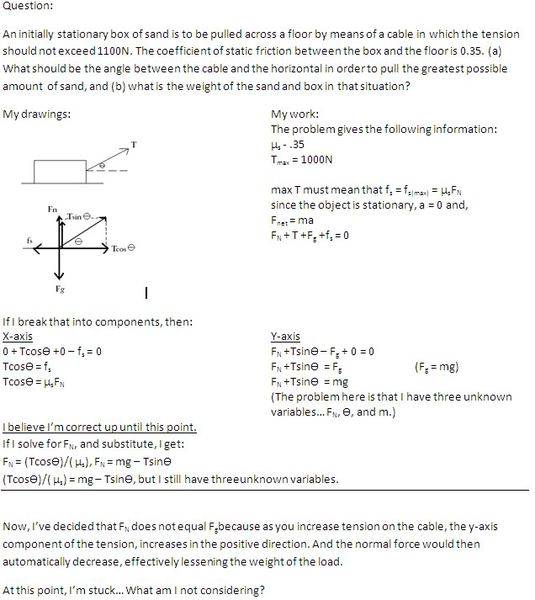
Having difficulties with this, I assume that something's supposed to cancel out at some point, but I must be missing something important. If someone could point em in the right direction... Thanks for all your help, once again!
(http://i51.photobucket.com/albums/f362/BeamsBox/Math_Physics.jpg)
Any ideas?
(http://i51.photobucket.com/albums/f362/BeamsBox/Math_Physics.jpg)
Any ideas?