How to Prove the Lagrange Inversion Theorem?

AdrianZ · Dec 8, 2011

I encountered this beautiful theorem and then I tried hard to prove it using ordinary algebraic methods and my understanding of calculus without involving real analysis in it but I didn't succeed. The theorem states that if f is an analytical function at some point x=a then f_-1 has the following Taylor series:

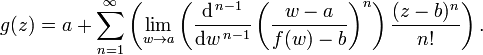
How can I prove this formula?

AdrianZ · Dec 9, 2011

No one knows?

How to Prove the Lagrange Inversion Theorem?

Discussion

Undergrad Why ##a^0=1##?

Undergrad Finding the minimum distance between two curves

High School Arc Length for Hyperbolic Sin

Undergrad Best way to numerically solve this system of equations?

Undergrad Why is ##x=e^y## the inverse of ##y=\int_1^x \frac{1}{t} dt##?

Undergrad Correct Upper/Lower limits for continuation of solutions for ODE

Graduate About ellipticity and a proof that a system of PDEs is elliptic

Graduate Leibniz Rule Videos on Digital-University

Insights Revisiting the Velocity-Time Function

Insights Remote Operated Gate Control System

Insights AI Enriched Problem Solving

Insights Thinking Outside The Box Versus Knowing What’s In The Box

Insights Why Entangled Photon-Polarization Qubits Violate Bell’s Inequality

Insights Quantum Entanglement is a Kinematic Fact, not a Dynamical Effect

How to Prove the Lagrange Inversion Theorem?

Discussion

Similar threads

Graduate Proof of Lagrange inversion theorem

Graduate The error caused by the lagrange inversion

How to Prove the Lagrange Interpolation Formula?

Programs How good is this applied math program?