- #1
Jhenrique
- 685
- 4
I was verifying that:
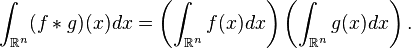
http://en.wikipedia.org/wiki/Convolution#Integration
But, I'd like to know if this equality is true:
[tex]\int f(x)g(x)dx \overset{?}{=} \int f(x)dx \ast \int g(x)dx[/tex]
Subquestion: this theorem above can be applied in summation too?
[tex]\sum_{x}\left (f(x)\ast f(x) \right )\overset{?}{=}\left(\sum_{x} f(x)\right)\left (\sum_{x} g(x) \right )[/tex]
[tex]\sum_{x}f(x)g(x) \overset{?}{=} \sum_{x}f(x) \ast \sum_{x}g(x)[/tex]
Thanks!
http://en.wikipedia.org/wiki/Convolution#Integration
But, I'd like to know if this equality is true:
[tex]\int f(x)g(x)dx \overset{?}{=} \int f(x)dx \ast \int g(x)dx[/tex]
Subquestion: this theorem above can be applied in summation too?
[tex]\sum_{x}\left (f(x)\ast f(x) \right )\overset{?}{=}\left(\sum_{x} f(x)\right)\left (\sum_{x} g(x) \right )[/tex]
[tex]\sum_{x}f(x)g(x) \overset{?}{=} \sum_{x}f(x) \ast \sum_{x}g(x)[/tex]
Thanks!