- #1
Novark
- 15
- 0
I understand everything up to the point which I've marked in red...
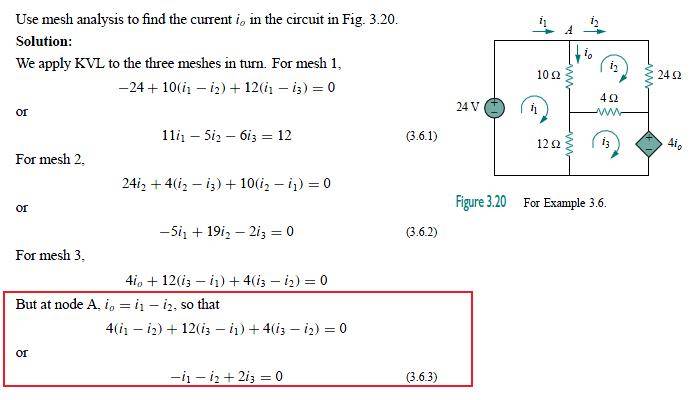
Can anyone explain those few lines?
I would greatly appreciate it!
Thanks.
Can anyone explain those few lines?
I would greatly appreciate it!
Thanks.