- #1
pbody
- 41
- 0
1. Nellie Newton hangs at rest from the ends of the rope as shown. How does the reading on the scale compare with her weight?
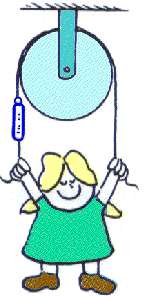 2. ANSWER FROM BOOK.
2. ANSWER FROM BOOK.
The scale will read half her weight. In this way, the net force (upward pull of left rope + upward pull of right rope rope weight = 0
3. Can somebody explain to me what the answer means, and how I can understand this better?
How would I know it would read half her weight?
The scale will read half her weight. In this way, the net force (upward pull of left rope + upward pull of right rope rope weight = 0
3. Can somebody explain to me what the answer means, and how I can understand this better?
How would I know it would read half her weight?