- #1
GreyGus
- 23
- 0
Homework Statement
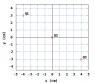
Three positive charges are located in the x-y plane (see plot below), with Q1=3.10 μC, Q2=5.20 μC and Q3=6.30 μC. Note that the charges are located at grid intersections, and that the x and y coordinates are in cm.
dynamically generated plot
A. Calculate the magnitude of the electric force on Q1 due to Q2.
Homework Equations
F=kq1q2/r^2
The Attempt at a Solution
Fx=k(3.1*10^-6)(5.2*10^-6)/((4/100)^2)
Fy=k(3.1*10^-6)(5.2*10^-6)/((3/100)^2)
Then for the magnitude: sqrt((160.977)^2+(90.54)^2))
Thank you in advance because I really cannot figure out what I'm doing wrong.