10^(1/10) is VERY close to 2^(1/3)

[Juanda]

Is there a subjacent reason that explains why these two numbers are so close?
$$10^{1/10} \approx 2^{1/3}$$

For context, this is where I found out about this.
Source: https://www.instarengineering.com/p...ration_Testing_of_Small_Satellites_Part_5.pdf

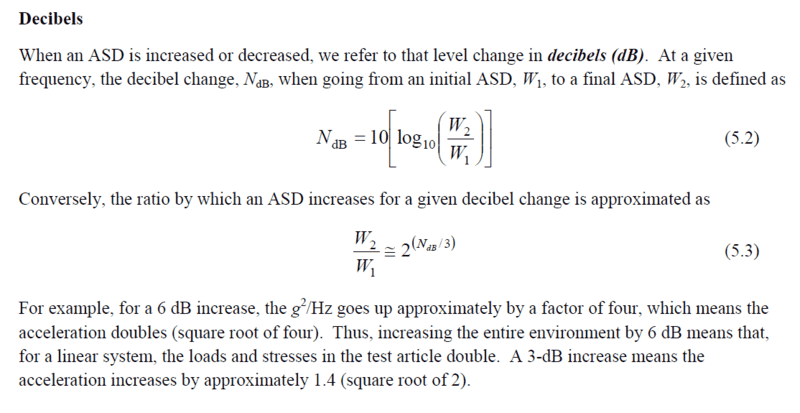
Is it just a coincidence? I tried factoring the numbers but it doesn't provide any additional information. From the infinite number of possibilities, how did they realize that $10^{1/10} \approx 2^{1/3}$ are so close together that it is acceptable to use the $2^{1/3}$ without losing too much accuracy?

 

[Hill]

$2^{10}=1024, \sqrt[3] {1024} \approx 10$

 

[Juanda]

How did I not see it?
Thank you. It's clear now.

 

[Mark44]

Is it just a coincidence?

Pretty much -- $10^{1/10}$ is not all that close to $2^{1/3}$.
The first is ~1.2599, and the second is ~1.2589. Rounding to 3 decimal places gives 1.260 vs. 1.259. I guess these are close if you consider $\sqrt 2 \approx 1.4$ as they did in the quoted article.