karush
Gold Member
MHB
- 3,240
- 5
Find Parametric eq for line segment from (-2,18,31) to (11,-4,48)
ok not sure how to start on this the book example is in the spoiler
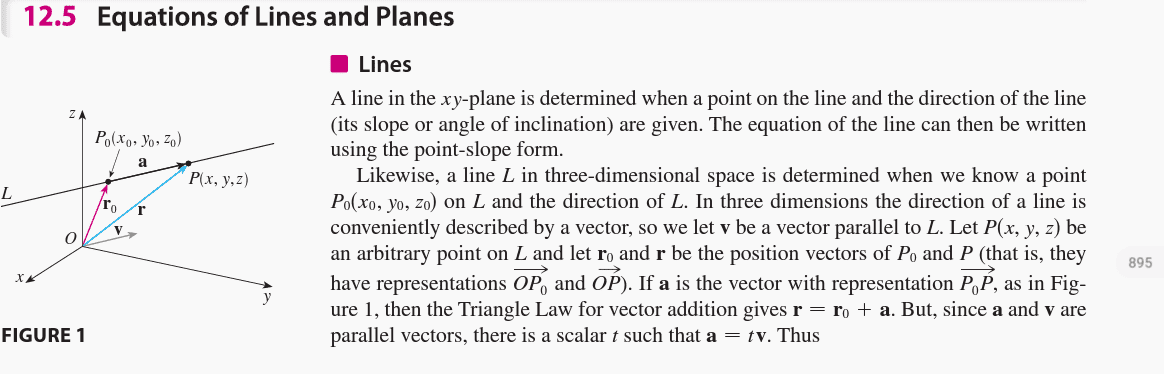
ok not sure how to start on this the book example is in the spoiler