- #1
Sebi123
- 14
- 1
I'm reading Thermodynamics: Foundations and Applications by Gyftoploulos and Beretta, because the authors claim to give a presentation of classical thermodynamics without "... the lack of logical consistency and completeness in the many presentations of the foundations of thermodynamics" [from the preface], a problem I have also experienced in my education by reading some of the "standard" textbooks. Although I was looking forward to "understand" the subject once and for all I have to admit that this type of presentation raises more questions than it should solve. I obviously do not really understand their formulations of the 1st and 2nd law, because I can think of very simple counterexamples where they seem to fail:
1st law
Their 1st law is [section 3.2]:
"Any two states of a system may always be the end states of a weight process, that is, the initial and final states of a change of state that involves no net effects external to the system except the change in elevation between z1 and z2 of a weight. Moreover, for a given weight, the value of the quantity Mg(z1 — z2) is fixed by the end states of the system, and independent of the details of the weight process, where M is the mass of the weight and g the gravitational acceleration."
From this statement they directly derive a property called "energy", which can be defined as
E = E0 - Mg(z - z0) where E0 is an arbitrary reference energy and z0 the reference height of the weight.
In my opinion this statement can only be true for closed systems, because I can think of no weight process that is equivalent to a process in which the system receives or loses some amount of matter. However, they don't explicitly confine their 1st law to closed systems. And even if they rephrased the first sentence to "Any two states of a closed system ...", what about the following example:
A closed composite system consists of two subsystems, A and B, which are connected by a movable piston allowing the exchange of particles [section 6.1]:
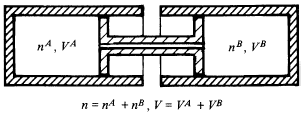
The only parameter is volume V = VA + VB (that's not the point here) and the number of particles is restricted by the equation n = nA + nB.
Assuming open systems are excluded by the 1st law, I see no justification to assign a property called "energy" to each of the subsystems, because both are open. I also have a problem saying "the composite (A+B) has a property called 'energy', so each subsystem also has such a property". I mean I also can't say: "(A+B) is divisible by 2, so A and B also have the property beeing divisible 2". Not beeing able to assign "energy" to open systems seems very weird to me, so what do I misunderstand here, using only the information given in the book and no previous knowledge from other sources?
2st law
Their 2nd law is [section 4.4]:
"Among all the states of a system that have a given value E of the energy and are compatible with a given set of values n of the amounts of constituents and β of the parameters, there exists one and only one stable equilibrium state. Moreover, starting from any state of a system it is always possible to reach a stable equilibrium state with arbitrarily specified values of amounts of constituents and parameters by means of a reversible weight process."
Consider the following mechanical system of a point mass in a constant gravitational field. According to my understanding of the definition of a well defined system (chapter 1 in the book) this system can also be regarded as a valid thermodynamic system. (Indeed, the authors use a point mass system in example 3.9, p. 37 to make clearer the concept of energy.) According to the authors' formulation of the 2nd law there must a stable equilibrium state according to the energy E = mgh (where I set the reference energy E0 to zero) this system currently has.
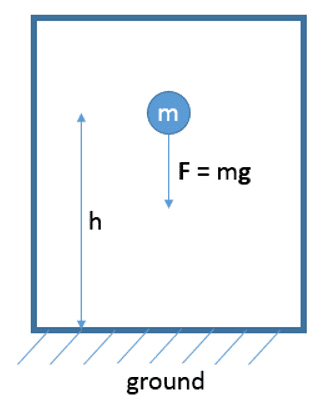
However, as the authors explain in section 4.3, in a mechanical system there is only one stable equilibrium state: the state of minimum energy (point mass on the ground with speed= 0). Doesn't this contradict their formulation of the 2nd law, because the system shown with E = mgh is obviously not in a stable equilibrium state: One can easily extract energy (mgh) from the point mass while the mass is falling.
Entropy
The authors introduce entropy by the definition S = S0 + 1/cR[(E - E0) - (ΩR - Ω0R))]. ΩR is the "available energy", that is "the largest amount of energy that can be transferred to a weight out of the composite of a system A and a given reservoir R in a weight process" [section 6.6.2]. So, the definition of entropy relies on the definition of available energy which in turn relies on the definition of reservoir. In section 6.3 the term "reservoir" is defined, but the authors admit that the the statements defining a reservoir "... are so restrictive that they must be regarded as limits that a system obeying the laws of physics can approach but cannot actually reach" [section 6.3]. How can an exact property like entropy be defined in terms of an unphysical system like a reservoir?
1st law
Their 1st law is [section 3.2]:
"Any two states of a system may always be the end states of a weight process, that is, the initial and final states of a change of state that involves no net effects external to the system except the change in elevation between z1 and z2 of a weight. Moreover, for a given weight, the value of the quantity Mg(z1 — z2) is fixed by the end states of the system, and independent of the details of the weight process, where M is the mass of the weight and g the gravitational acceleration."
From this statement they directly derive a property called "energy", which can be defined as
E = E0 - Mg(z - z0) where E0 is an arbitrary reference energy and z0 the reference height of the weight.
In my opinion this statement can only be true for closed systems, because I can think of no weight process that is equivalent to a process in which the system receives or loses some amount of matter. However, they don't explicitly confine their 1st law to closed systems. And even if they rephrased the first sentence to "Any two states of a closed system ...", what about the following example:
A closed composite system consists of two subsystems, A and B, which are connected by a movable piston allowing the exchange of particles [section 6.1]:
The only parameter is volume V = VA + VB (that's not the point here) and the number of particles is restricted by the equation n = nA + nB.
Assuming open systems are excluded by the 1st law, I see no justification to assign a property called "energy" to each of the subsystems, because both are open. I also have a problem saying "the composite (A+B) has a property called 'energy', so each subsystem also has such a property". I mean I also can't say: "(A+B) is divisible by 2, so A and B also have the property beeing divisible 2". Not beeing able to assign "energy" to open systems seems very weird to me, so what do I misunderstand here, using only the information given in the book and no previous knowledge from other sources?
2st law
Their 2nd law is [section 4.4]:
"Among all the states of a system that have a given value E of the energy and are compatible with a given set of values n of the amounts of constituents and β of the parameters, there exists one and only one stable equilibrium state. Moreover, starting from any state of a system it is always possible to reach a stable equilibrium state with arbitrarily specified values of amounts of constituents and parameters by means of a reversible weight process."
Consider the following mechanical system of a point mass in a constant gravitational field. According to my understanding of the definition of a well defined system (chapter 1 in the book) this system can also be regarded as a valid thermodynamic system. (Indeed, the authors use a point mass system in example 3.9, p. 37 to make clearer the concept of energy.) According to the authors' formulation of the 2nd law there must a stable equilibrium state according to the energy E = mgh (where I set the reference energy E0 to zero) this system currently has.
However, as the authors explain in section 4.3, in a mechanical system there is only one stable equilibrium state: the state of minimum energy (point mass on the ground with speed= 0). Doesn't this contradict their formulation of the 2nd law, because the system shown with E = mgh is obviously not in a stable equilibrium state: One can easily extract energy (mgh) from the point mass while the mass is falling.
Entropy
The authors introduce entropy by the definition S = S0 + 1/cR[(E - E0) - (ΩR - Ω0R))]. ΩR is the "available energy", that is "the largest amount of energy that can be transferred to a weight out of the composite of a system A and a given reservoir R in a weight process" [section 6.6.2]. So, the definition of entropy relies on the definition of available energy which in turn relies on the definition of reservoir. In section 6.3 the term "reservoir" is defined, but the authors admit that the the statements defining a reservoir "... are so restrictive that they must be regarded as limits that a system obeying the laws of physics can approach but cannot actually reach" [section 6.3]. How can an exact property like entropy be defined in terms of an unphysical system like a reservoir?
Last edited: