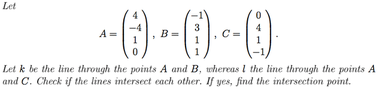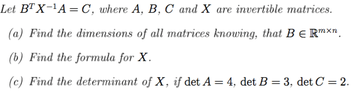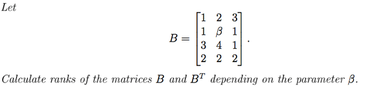3 Algebra problems that I with.

[namerequired]

Hello everybody, I am Francesco and I would be very grateful if someone could show me the solution for those 3 problems. I have many of them and these are just an example for type, so if I understand I can do the rest of my own. I don't find on google an exact answer to my problems, but all the time something similar but never the same :S. Thank you in advance if you can help the fellow here. (Angry)

1)

View attachment 1919

This one I don't understand if it is too easy or I am doing it wrong. Because the logical answer is: Coordinate of the point A am I wrong?

2)

View attachment 1920

3)

View attachment 1921

 

[Fantini]

Hello Francesco! Welcome to MHB. :) We'll be happy to guide you to a greater understanding of these concepts, but we don't do that by giving you the solution: we'll walk the path with you, instead. Therefore, what did you attempt on questions 2 and 3? Namely, to arrive at their solutions you must answer positively to these questions:

1) Do you know what it means for a matrix to be invertible?

2) Are you familar with determinant properties?

3) Do you know how to find the rank of a matrix?

4) How do you write a line passing through two points?

Hope to hear your answers and further help you. :)

Best wishes,

Fantini.

 

[MarkFL]

Hello and welcome to MHB, Francesco! (Wave)

In addition to showing what you have tried as Fantini has stated, we also ask that no more than two questions be posted per thread. This just helps keep a thread from becoming convoluted and hard to follow, particularly if more than one helper is assisting you with different problems at the same time.

 

[namerequired]

1) Do you know what it means for a matrix to be invertible?

It should be a square matrix and it is the opposite of the original one and has to be determined, so it can't have Det = 0.

2) Are you familar with determinant properties?

det A = Det A^T
The position matter
det(AB) = det(A) and det(B)
for invertible matrices det(A) cannot be 0

3) Do you know how to find the rank of a matrix?

Row operations to find the diagonal, or check if the det of a sub matrix 2x2, 3x3 is not 0

4) How do you write a line passing through two points?

I look for the coordinate of the 2 points.I hope it is more or less right )) - Sorry to post more than 2, actually the first question was more a bonus, because I don't really understand if the problem is so easy or so tricky )

 

[Fantini]

It should be a square matrix and it is the opposite of the original one and has to be determined, so it can't have Det = 0.

Exactly. The information that it is an square matrix is important. Using "opposite" as terminology is not adequate here in my opinion, as I usually associate that word for addition. So consider this: if $B \in \mathbb{R}^{m \times n}$ and $B$ is invertible, then what can you say about $m$ and $n$? This answers more than just $B$: the dimensions of all other matrices are automatically determined.

det A = Det A^T
The position matter
det(AB) = det(A) and det(B)
for invertible matrices det(A) cannot be 0

You are correct. In order to apply that information you need to isolate $X$ in the equation $B^{T} X^{-1} A = C$. Looking at it, we can see some possible orders to do this. I would do as the following:

1) Eliminate $A$ (how?), transpose what the rest (to eliminate $B$), invert and transpose back.

Any other order is essentially an rearrangement of the above.

Row operations to find the diagonal, or check if the det of a sub matrix 2x2, 3x3 is not 0

These are correct, but rather tedious. In particular there are many determinants to be computed, so it's not really a good option. What else can we do? This information is important: the rank of the matrix is always less than or equal to the minimum between the number of rows and columns. Let's apply in this case: we have 4 rows and 3 columns, therefore whatever the rank of the matrix is it has to be less than or equal to 3.

How can we check that? Consider the three columns as vectors and make a linear combination of them, equating to zero. From here you should find the condition on $\beta$ that answers the problem.

I look for the coordinate of the 2 points.

This isn't correct. You already have two points and you want a line between them. The most common way (but definitely not unique) to define such line is to write it as

$$r = \{ P_1 + t(P_2 - P_1), \, t \in \mathbb{R} \}.$$

The parameter $t$ controls where you are in the line. At $t=0$ you start at point $P_1$, while at $t=1$ you are at $P_2$. It is usual to think of it as "time" (hence the letter $t$) and this parametrization of the line as the unit time taken to walk the line leaving one point and arriving at another.

Try using it this time: first write the equations of lines $AB$ and then $AC$. What will be the condition for them to intersect? :)