- #1
Sunny Sun
- 1
- 0
Not homework, but I hope this is the right forum to post in:
1. Homework Statement
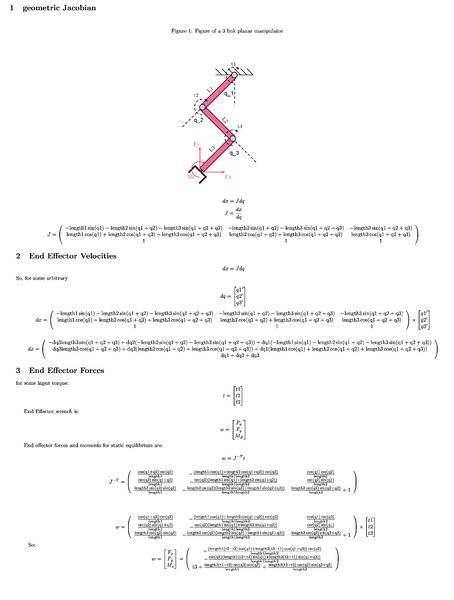
So we have a 3-link planar manipulator, with lengths L1,L2,L3 and angles q1,q2,q3.
About each of the joints, we may apply a torque, t1,t2,t3.
At the end effector, there will be a force and moment that will fulfill static equilibrium with the input joint torques.
So we can go through the rigmarole and compute the geometric jacobian, which is a map from your angular velocies to your linear endpoint velocities. dx = J dq.
From my image, you can see that the linear velocities care about the orientation of the 3rd limb.
Now, using the principles of virtual work, we can compute the end effector moment and forces that we need in order to balance the input torques.
W = J^-T t, where W is the end effector wrench and t is a joint torques vector and J^-T is the inverse transpose of J.
You can see from the analytical solution, that the Fx and Fy do not depend on the orientation q3 of the 3rd limb!
if that image is too blurry:
https://i.redd.it/nmpd2ekfv3f11.jpg
A puzzling observation. Can someone explain why conceptually that the orientation of the 3rd link has no effect on the Fx and Fy, but does change the end effector moment Mz?
1. Homework Statement
So we have a 3-link planar manipulator, with lengths L1,L2,L3 and angles q1,q2,q3.
About each of the joints, we may apply a torque, t1,t2,t3.
At the end effector, there will be a force and moment that will fulfill static equilibrium with the input joint torques.
Homework Equations
So we can go through the rigmarole and compute the geometric jacobian, which is a map from your angular velocies to your linear endpoint velocities. dx = J dq.
From my image, you can see that the linear velocities care about the orientation of the 3rd limb.
Now, using the principles of virtual work, we can compute the end effector moment and forces that we need in order to balance the input torques.
W = J^-T t, where W is the end effector wrench and t is a joint torques vector and J^-T is the inverse transpose of J.
You can see from the analytical solution, that the Fx and Fy do not depend on the orientation q3 of the 3rd limb!
The Attempt at a Solution
if that image is too blurry:
https://i.redd.it/nmpd2ekfv3f11.jpg
A puzzling observation. Can someone explain why conceptually that the orientation of the 3rd link has no effect on the Fx and Fy, but does change the end effector moment Mz?
