3 mass coupled oscillators

[Lambda96]

Homework Statement

See Screenshot

Relevant Equations

none

Hi,

I am not sure if I have derived the matrix correctly, because of my results in task b

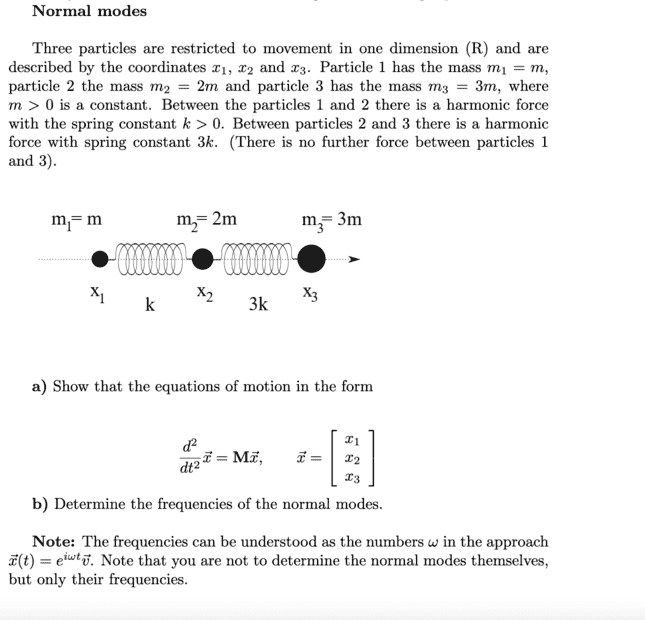
I solved task 1 as follows, I assumed that all three particles move to the right

$$m \dot{x_1}=-k(x_1 - x_2)$$
$$2m \dot{x_2}=-k(x_2-x_2)-3k(x_2-x_3)$$
$$3m \dot{x_3}=-3k(x_3-x_2)$$

Then I simply divided all three equations by the masses and got the following form

$$ \dot{x_1}=-\frac{k}{m}(x_1 - x_2)$$
$$ \dot{x_2}=-\frac{k}{2m}(x_2-x_2)-\frac{3k}{2m}(x_2-x_3)$$
$$ \dot{x_3}=-\frac{k}{m}(x_3-x_2)$$

Then I set up the 3 equations in the required matrix form:$$\frac{d}{dt^2} \vec{x}=\left( \begin{array}{rrr}
-\frac{k}{m} & \frac{k}{m} & 0 \\
\frac{k}{2m} & -(\frac{k}{2m}+\frac{3k}{2m}) & \frac{3k}{2m} \\
0 & \frac{k}{m} & -\frac{k}{m} \\
\end{array}\right) \left( \begin{array}{rrr}
x_1 \\
x_2 \\
x_3 \\
\end{array}\right)$$

For task part b, I simply put $\vec{x}(t)=e^{i \omega t} \vec{v}$ into the matrix above and then divided out the exponential term on both sides.$$ -\omega^2 \vec{v}= \left( \begin{array}{rrr}
-\frac{k}{m} & \frac{k}{m} & 0 \\
\frac{k}{2m} & -(\frac{k}{2m}+\frac{3k}{2m}) & \frac{3k}{2m} \\
0 & \frac{k}{m} & -\frac{k}{m} \\
\end{array}\right) \vec{v}$$

After that I just got everything on one site and used that $\frac{k}{m}=\omega_0$ is

$$ \left( \begin{array}{rrr}
0 \\
0 \\
0 \\
\end{array}\right)= \left( \begin{array}{rrr}
-\frac{k}{m}+\omega^2 & \frac{k}{m} & 0 \\
\frac{k}{2m} & -(\frac{k}{2m}+\frac{3k}{2m})+\omega^2 & \frac{3k}{2m} \\
0 & \frac{k}{m} & -\frac{k}{m}+\omega^2 \\
\end{array}\right) \vec{v}$$

Now, to determine $\omega$, I simply formed the determinant of the matrix and got the following:

$$\frac{3 \omega_0^6}{2}+\frac{3 \omega_0^4 \omega^2}{2}-2 \omega_0^2 \omega^4- \omega^6=0$$

If I now solve the equation for $\omega$, I get the following values

$$\omega_1=\pm \omega_0$$
$$\omega_2=\pm \sqrt{\frac{1}{2}(3+ \sqrt{3})}\sqrt{-\omega_0^2}$$
$$\omega_3=\pm \frac{\sqrt{(\sqrt{3}-3) \omega_0^2}}{\sqrt{2}}$$

 

[pasmith]

You can simplify your calculations by writing the system as $$\ddot{\mathbf{x}} + \omega_0^2 \begin{pmatrix} 1 & -1 & 0 \\ -\tfrac12 & 2 & -\tfrac32 \\ 0 & -1 & 1 \end{pmatrix}\mathbf{x} = 0$$ where $\omega_0^2 = k/m$. Then after substituting $\mathbf{x} = \mathbf{v}e^{i\omega t}$ you can set $\omega^2 = \lambda \omega_0^2$ to obtain $$\omega_0^2\begin{pmatrix} 1 - \lambda & -1 & 0 \\ -\tfrac12 & 2 - \lambda & -\tfrac 32 \\ 0 & -1 & 1 - \lambda \end{pmatrix}\mathbf{v} = 0.$$ The determinant is then (EDIT: This is incorrect; please see below) $$(1 - \lambda)((2 - \lambda)(1 - \lambda) + \tfrac32) - \tfrac12 (1 - \lambda) = (1- \lambda)(\lambda^2 - 3\lambda + 3).$$ Thus we have $\lambda = 1, \sqrt{3}e^{\pm i\pi/6}$. Therefore $$\frac{\omega}{\omega_0} = \pm 1, 3^{1/4}e^{\pm i\pi/12}, 3^{1/4}e^{\pm i 5 \pi /12}.$$ We can immediately see that the roots occur in complex conjugate pairs, as expected.

 

[vanhees71]

I've not explicitly checked the calculation, but how can you get complex eigenvalues for a non-dissipative system of harmonic oscillators?

 

[pasmith]

I've not explicitly checked the calculation, but how can you get complex eigenvalues for a non-dissipative system of harmonic oscillators?

Yes, I see I have made a sign error; the determinant should be $$(1 - \lambda)((2-\lambda)(1 - \lambda) - \tfrac32 ) - \tfrac12(1 - \lambda) = (1 - \lambda)(\lambda^2 - 3\lambda).$$

 

[vanhees71]

That looks good!

 

[pasmith]

I should also note that we expect a zero eigenvalue: The centre of mass does not accelerate, so $$m_i \ddot x_i = m_i M_{ij} x_j = 0$$ for every $x_j$. But this requires that $$m_i M_{ij} = 0$$ so $m_i \neq 0$ is a left eigenvector with eigenvalue zero.

 

[Lambda96]

Thank you pasmith and vanhees71 for your help , sorry I'm only getting back to you now, the last few weeks have been a bit stressful

Now I have understood how I can solve the task much easier, thank you very much

One quick question, does the sign of the eigenvalues actually tell me in which direction the masses are moving?

 

[pasmith]

One quick question, does the sign of the eigenvalues actually tell me in which direction the masses are moving?

No. If $\lambda < 0$ then $\mathbf{x} = \mathbf{v}e^{\pm \omega_0\sqrt{|\lambda|}t}$ and we have exponential growth or decay. Thus we must have $\lambda \geq 0$. The zero eigenvalue corresponds to a constant drift of the centre of mass; the other eigenvalues give the frequencies of oscillation about the centre of mass. The corresponding eigenvectors will show you the relative motion of the masses.