311.1.5.19 parametric equation of the line through a parallel to b.

[karush]

$\tiny{311.1.5.19}$
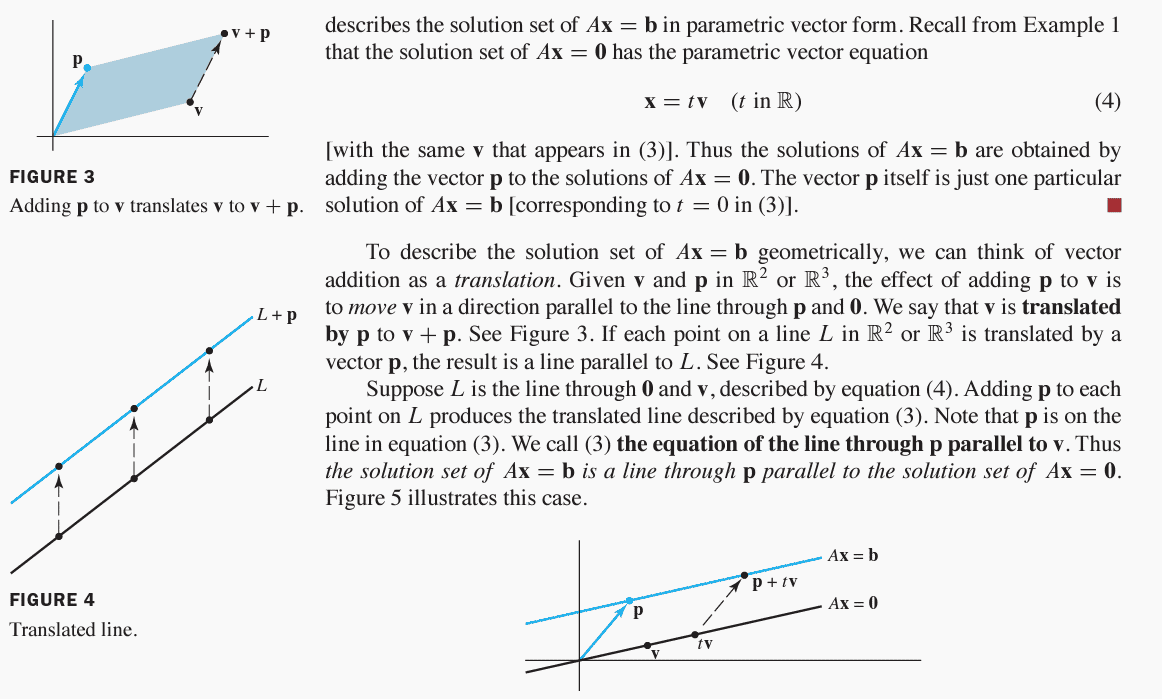
find the parametric equation of the line through a parallel to b.
$a=\left[\begin{array}{rr}
-2\\0
\end{array}\right],
\, b=\left[\begin{array}{rr}
-5\\3
\end{array}\right]$

ok I know this like a line from 0,0 to -5,3 and $m=dfrac{-5}{3}$
so we could get line eq with point slope formula

but this is be done by parametric eq

this was book section I tried to follow..

anyway...

$x=a+tb=
\left[\begin{array}{rr}
-2\\0
\end{array}\right]+t
\left[\begin{array}{rr}
-5\\3
\end{array}\right]$

maybe

 

[HOI]

Didn't you check this yourself?

When t= 0, [math]\begin{bmatrix}-2 \\0 \end{bmatrix}+ 0\begin{bmatrix}-5 \\ 3\end{bmatrix}=\begin{bmatrix}-2 \\0 \end{bmatrix}[/math] so it goes through the right point, a= (-2, 0).

When t= 1, [math]\begin{bmatrix}-2 \\0 \end{bmatrix}+ 1\begin{bmatrix}-5 \\ 3\end{bmatrix}=\begin{bmatrix}-7 \\3 \end{bmatrix}[/math] so it also goes through (-7, 3).

And the vector from (-2, 0) to (-7, 3) is [math]\begin{bmatrix}-7- (-2) \\ 3- 0\end{bmatrix}=\begin{bmatrix}-5 \\ 3 \end{bmatrix}[/math] as desired.