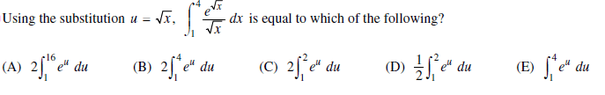karush
Gold Member
MHB
- 3,240
- 5
View attachment 9321
calculator returned this but know sure why
$\displaystyle2 \int _1^2e^udu$
note there might be a duplicat of this post ?
calculator returned this but know sure why
$\displaystyle2 \int _1^2e^udu$
note there might be a duplicat of this post ?