- #1
Kulkarni Sourabh
- 2
- 1
- Homework Statement
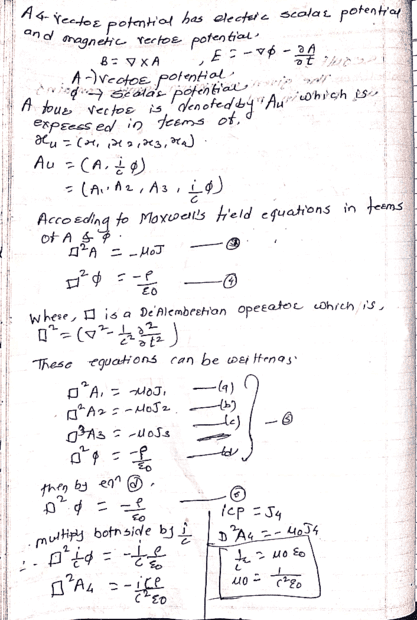
- 4- vector potential transformation under Gauge fixing.
- Relevant Equations
- .
What is 4- vector potential transformation under Gauge fixing ?
A 4-vector potential in electromagnetism is a four-dimensional vector that combines the electric potential (scalar potential) and the magnetic vector potential into a single entity. It is denoted as \( A^\mu = ( \phi, \mathbf{A} ) \), where \( \phi \) is the electric potential and \( \mathbf{A} \) is the magnetic vector potential. This formulation is particularly useful in the context of special relativity.
Under a Lorentz transformation, the components of the 4-vector potential transform according to the Lorentz transformation matrix. If \( \Lambda \) represents the Lorentz transformation matrix and \( A^\mu \) is the 4-vector potential, then the transformed 4-vector potential \( A'^\mu \) is given by \( A'^\mu = \Lambda^\mu_{\ \nu} A^\nu \). This ensures that the physical laws remain invariant under changes in inertial reference frames.
The 4-vector potential is important in the theory of relativity because it provides a covariant formulation of electromagnetism, meaning it respects the principles of special relativity. By combining the electric and magnetic potentials into a single 4-vector, the equations of electromagnetism can be written in a form that is invariant under Lorentz transformations, making them consistent with the theory of relativity.
The electromagnetic field tensor \( F^{\mu\nu} \) is derived from the 4-vector potential \( A^\mu \). The components of the field tensor are given by \( F^{\mu\nu} = \partial^\mu A^\nu - \partial^\nu A^\mu \). This tensor encapsulates both the electric and magnetic fields in a covariant form, and it is antisymmetric, meaning \( F^{\mu\nu} = -F^{\nu\mu} \).
Gauge transformations change the 4-vector potential without altering the physical electromagnetic fields. A gauge transformation adds the gradient of a scalar function \( \Lambda \) to the 4-vector potential: \( A^\mu \rightarrow A^\mu + \partial^\mu \Lambda \). Despite this change in the potential, the electromagnetic field tensor \( F^{\mu\nu} \) remains unchanged, ensuring that observable quantities like the electric and magnetic fields are unaffected.