- #1
JessicaHelena
- 188
- 3
- Homework Statement
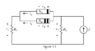
- Two non-linear devices are placed in parallel in the circuit show in Figure 3-1. The resistors in the circuit have the following values: ##R_1 = 2## Ohms and ##R_2 = 1## Ohms.
The non-linear devices have different current-voltage (I-V) characteristics shown in Figure 3-2, where ##I_S = 1A## and ##V_S = 1V##.
1. Given that the current source, I = 3A, calculate the numerical value for v1 and v2.
2. Now assume that the current source, I = 1A, and calculate the numerical value for v1 and v2.
- Relevant Equations
- KVL, KCL
I honestly don't know how to quite even begin this problem.
Looking at Fig 3-2, the slopes of the graphs are 1/R, and hence where the slopes are 0, we have infinite resistance, in which case current wouldn't flow through that resistor and hence simplify the circuit. So I was trying to find ways to use that, only I don't really know what the conditions are for ##i_A, i_B, v_A, v_B##, and I don't think I could just randomly choose values I want to work with, could I?
Looking at Fig 3-2, the slopes of the graphs are 1/R, and hence where the slopes are 0, we have infinite resistance, in which case current wouldn't flow through that resistor and hence simplify the circuit. So I was trying to find ways to use that, only I don't really know what the conditions are for ##i_A, i_B, v_A, v_B##, and I don't think I could just randomly choose values I want to work with, could I?