A curvature problem (differentiation)

[DreamWeaver]

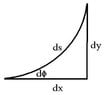
In the Euclidean plane, assume a differentiable function $$y=f(x)$$ exists. At any given point, say $$(x_0,y_0)$$, the line tangential to $$y=f(x)$$ at this point intersects the x-axis at an angle $$\phi$$.

The curvature of this curve, $$\kappa$$, is the rate of change of $$\phi$$ with respect to arc length, $$s$$:

$$\kappa = \frac{d\phi}{ds} $$Problem:Prove that

$$\kappa = \frac{ \left[ 1+ \left( \frac{dy}{dx} \right)^2 \right]^{3/2} }{ \frac{d^2y}{dx^2} }$$Or equivalently

$$\kappa = \frac{\left[ 1+\left( f'(x) \right)^2 \right]^{3/2}}{f''(x)}$$

 

[DreamWeaver]

Here's an optional, visual aid, for a generic curve. Just in case... (Bandit)

Spoiler

View attachment 2614

 

[jacobi1]

Isn't

$$\kappa = \frac{f''(x)}{ \left [ 1+ (f'(x))^2 \right ]^{3/2}}$$?

The solution follows.

First, $$\frac{d \varphi}{ds}= \frac{\frac{ d \varphi}{dx}}{ \frac{ds}{dx}}$$.

If the tangent line to f(x) at $$x=x_0$$ intersects the x-axis at an angle $$\varphi$$, then $$f'(x_0) = \tan \varphi$$. Differentiating both sides with respect to $$x_0$$ and assuming $$\varphi$$ is a function of $$x_0$$ gives $$f''(x_0)=\varphi' \sec^2 \varphi $$. Solving and remembering that $$1+\tan^2 (x)=\sec^2 (x)$$, we have

$$\frac{f''(x_0)}{1+f'(x_0)^2}= \varphi'=\frac{d \varphi}{dx}$$.

Now, remembering the definition of arc length,

[math]\frac{ds}{dx}= \sqrt{1+f'(x)^2}[/math],

substituting into the first equation, and dropping subscripts gives our result,

$$\kappa= \frac{d \varphi}{ds}= \frac{f''(x)}{\left [1+f'(x)^2 \right]^{3/2}}$$.