A-level Mechanics Q3: Need Help w/Calculations

[Shah 72]

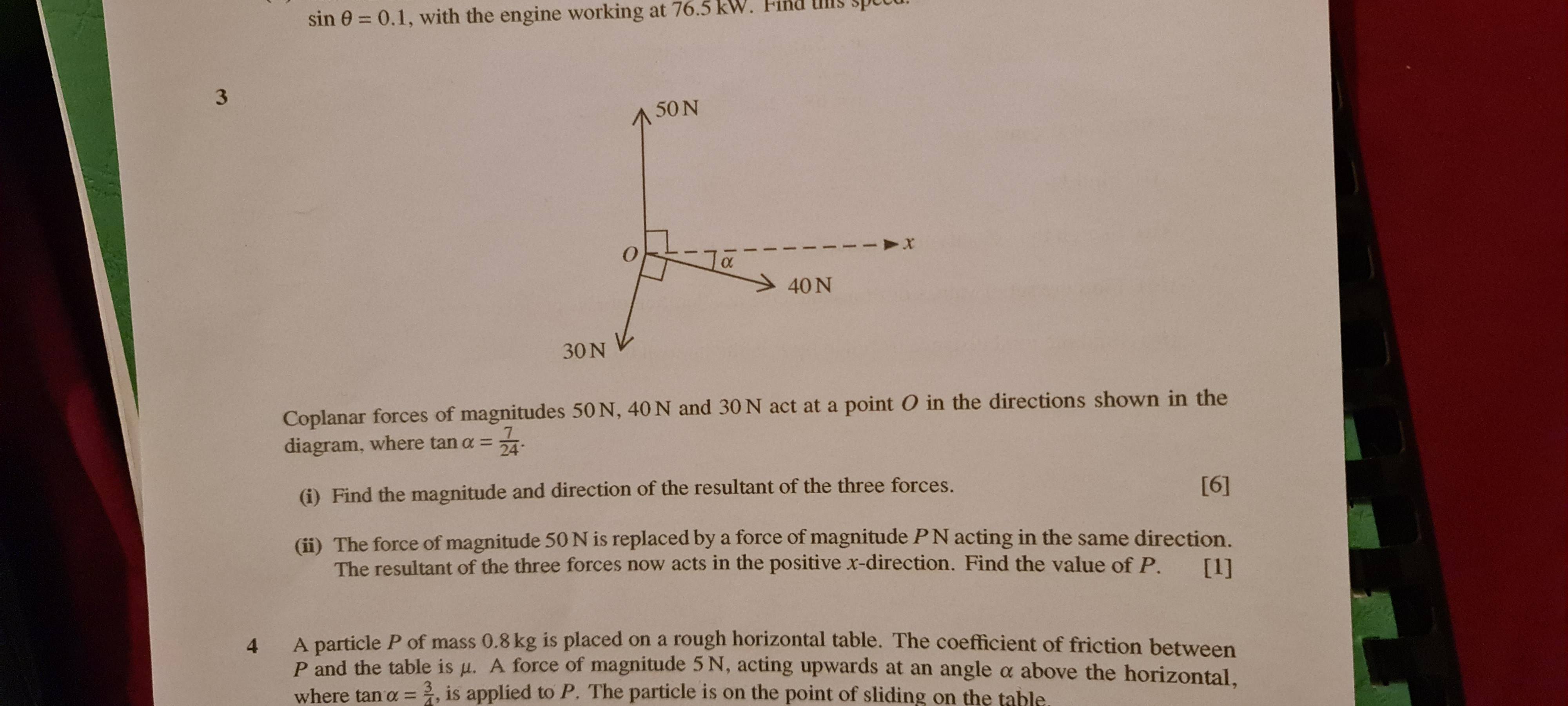
It's question 3. Iam getting a bit confused with the calculations. Pls help

 

[topsquark]

On which part are you stuck? In each case you add the vectors by components.

-Dan

 

[Shah 72]

On which part are you stuck? In each case you add the vectors by components.

-Dan

Iam getting confused with 30 N.
So f(x) = 40cos alpha - 30 cos alpha
F(y) = 40 sin alpha - 30 sin alpha

 

[topsquark]

You have three forces, each having x, y, and z components. So:
[math]40 ~ cos( \alpha ) \hat{i} ~ + 40 ~ sin( \alpha ) \hat{j} ~ \text{N}[/math]

[math]30 \hat{j} ~ \text{N}[/math]

[math]50 \hat{k} ~ \text{N}[/math]

Add them and you get F.

-Dan

 

[skeeter]

the problem statement says the three given forces are coplanar, so you are dealing with x and y components …

$\sum F_x = 0 + 40\cos{\alpha} - 30\sin{\alpha}$

$\sum F_y = 50 - 40\sin{\alpha} - 30\cos{\alpha}$

$|F_{net}| = \sqrt{(\sum F_x)^2+(\sum F_y)^2}$

$\theta = \arctan\left(\dfrac{\sum F_y}{\sum F_x}\right)$

 

[topsquark]

"Co-planar forces" Hee hee. Sorry for the mistake!

-Dan

 

[Shah 72]

the problem statement says the three given forces are coplanar, so you are dealing with x and y components …

$\sum F_x = 0 + 40\cos{\alpha} - 30\sin{\alpha}$

$\sum F_y = 50 - 40\sin{\alpha} - 30\cos{\alpha}$

$|F_{net}| = \sqrt{(\sum F_x)^2+(\sum F_y)^2}$

$\theta = \arctan\left(\dfrac{\sum F_y}{\sum F_x}\right)$

Thank you so so much!