- 15,825
- 8,983
- TL;DR Summary
- How to find the vector cross product without the right-hand rule with a simple method that anyone who can read can understand intuitively.
Although it is considered unwise to judge a book by its cover, a book's cover is still useful for finding the direction of the cross product ##\mathbf{A}\times \mathbf{B}## between two given vectors. Being able to read is all that is needed. Here is a detailed procedure.
Step 1. Move one vector parallel to itself so that the vectors are tail-to tail.
Step 2. Orient the pair as a unit so that the first vector in the cross product is in the plane of a table and the second vector is pointing in a direction away from the table1.
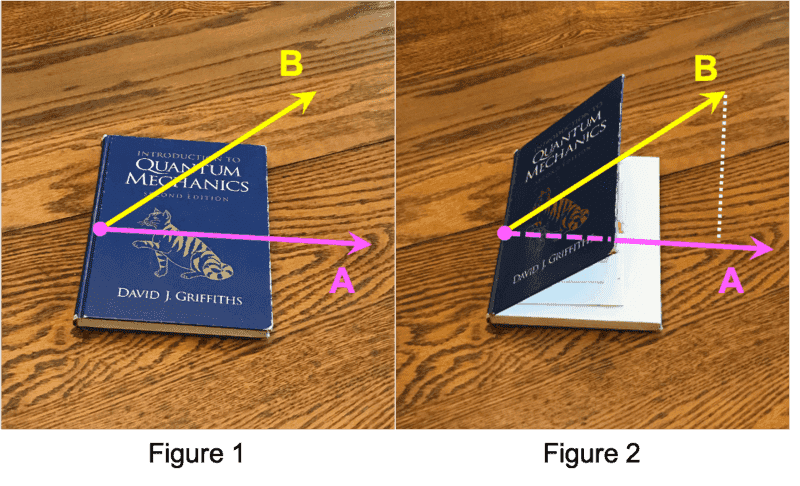
Step 3. Place a standard book flat on the table and orient it so that its spine is perpendicular to the plane defined by the two vectors and the title is read in the direction of the first vector ##\mathbf{A}## (see Figure 1 below.)
Step 4. Open the book so that the title points along the direction of the second vector ##\mathbf{B}## (see Figure 2 below)
Step 5. Read the title and author's name. The direction from one line to the next is the direction of the cross product.
Step 6. Measure the length of the vertical component of ##\mathbf{B} ## (dotted white line) and the length of the arrow representing ##\mathbf{A}##. The product of the two lengths is the magnitude of the cross product. Clearly, the cross product is zero when the book's cover after step 4 is parallel to the table.
Thus, anyone who can read a book2, including children, can immediately grasp how to apply this process and find the direction of the cross product without the use of right hands, advancing screws, three mutually perpendicular fingers, etc.
___________________________________________________________________________________
1 This can be done without loss of generality. Pedants, who insist that the vectors be written in terms of a coordinate system fixed in space, may find the appropriate Euler angle rotation matrix and rotate the table instead.
2 Books printed in languages that read right to left, e.g. Hebrew, Arabic, etc., should be oriented with the title of the book in the direction of the second vector and be opened with the cover matching the direction of the first vector.
Step 1. Move one vector parallel to itself so that the vectors are tail-to tail.
Step 2. Orient the pair as a unit so that the first vector in the cross product is in the plane of a table and the second vector is pointing in a direction away from the table1.
Step 3. Place a standard book flat on the table and orient it so that its spine is perpendicular to the plane defined by the two vectors and the title is read in the direction of the first vector ##\mathbf{A}## (see Figure 1 below.)
Step 4. Open the book so that the title points along the direction of the second vector ##\mathbf{B}## (see Figure 2 below)
Step 5. Read the title and author's name. The direction from one line to the next is the direction of the cross product.
Step 6. Measure the length of the vertical component of ##\mathbf{B} ## (dotted white line) and the length of the arrow representing ##\mathbf{A}##. The product of the two lengths is the magnitude of the cross product. Clearly, the cross product is zero when the book's cover after step 4 is parallel to the table.
Thus, anyone who can read a book2, including children, can immediately grasp how to apply this process and find the direction of the cross product without the use of right hands, advancing screws, three mutually perpendicular fingers, etc.
___________________________________________________________________________________
1 This can be done without loss of generality. Pedants, who insist that the vectors be written in terms of a coordinate system fixed in space, may find the appropriate Euler angle rotation matrix and rotate the table instead.
2 Books printed in languages that read right to left, e.g. Hebrew, Arabic, etc., should be oriented with the title of the book in the direction of the second vector and be opened with the cover matching the direction of the first vector.