A Simpler Way to Find the Shaded Area?

[Saracen Rue]

TL;DR Summary

Is there an easier way to determine what percentage of this square is bound by the shaded area of 4 line segments

Consider the following scenario:

Given that points $M$ and $N$ are the midpoints of their respective line segments, what would be the fastest way to determine what percentage of the squares total area is shaded purple?

I managed to determine that the purple shaded area is $5\text{%}$ as per my working below:

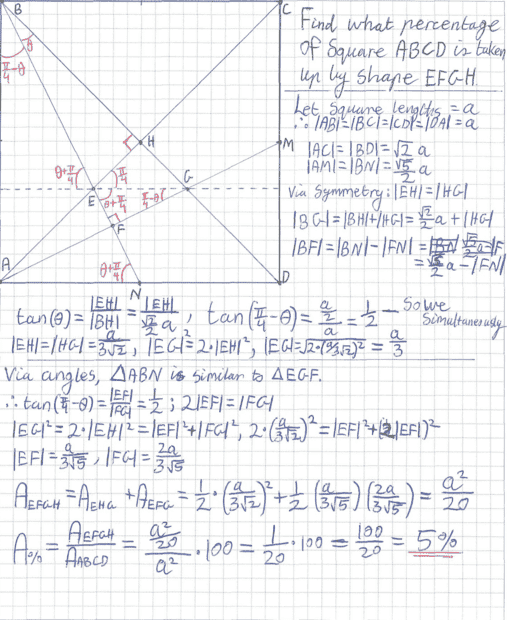
The only real problem I had with this is that it took me a genuinely long time to figure out. After I drew it up by hand, I suspected that line segment $EG$ was roughly equal to $\frac{a}{3}$ from visual inspection alone, and I did use a ruler to confirm this. However, it took me quite a while to prove it was mathematically.

I feel as though there must be a simpler way to go about solving the question using just geometry/trigonometry (I do realise that it'd probably be easy enough to solve if you put this on a Cartesian Plane with $A$ at the origin, but I wanted to try avoiding that method if possible), and if so, can anyone point me in the right direction?

 

[anuttarasammyak]

Say square ABCD=1
quadrilateral EFGH = $\triangle$ ACM - $\triangle$ AEF - quadrilateral HCMG
where
$\triangle$ACM=1/4,
$\triangle$ AEF = $\triangle$ AEN - $\triangle$ AFN = 1/12 - 1/20,
quadrilateral HCMG = $\triangle$CHD-$\triangle$ GDM = 1/4 - 1/12.