Iwanttolearnphysics
- 44
- 9
- Homework Statement
- "A ball falls vertically and bounces off the ground. Immediately before impact with the ground, the speed of the ball is u. Immediately after leaving the ground the speed is v. Which of the following expressions is the ratio of (kinetic energy lost on collision/kinetic energy immediately after a collision)?
- Relevant Equations
- KE = 1/2mv^2
Hello again. I don't believe there are rules about posting twice in a day. I'm not a student and I answer Physics questions as a hobby, but I've only just started learning, so please help me out. I'm answering IBDP Physics questions.

Here's my thinking:
I'm including a picture of my attempt to work it out, just in case no one understood what I wrote above.
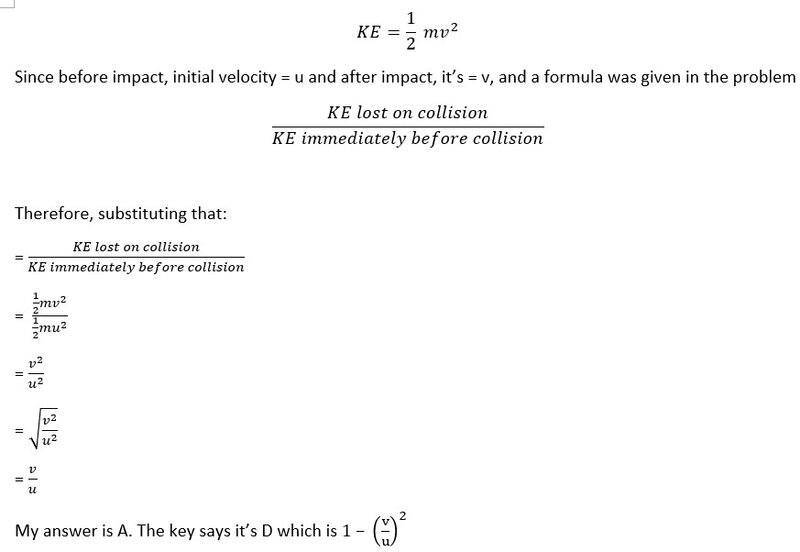
Here's my thinking:
- KE is not a vector quantity, so it's always just written as is.
- Velocity is a vector quantity, so taking into account that u moves downwards, I could show that as -u.
- However, -u^2 is still |u^2|
- Substituting from the ratio given in the problem, I could write this as (1/2mv^2)/(1/2mu^2)
- That gives me a final answer of v^2/u^2.
- I could square the whole thing and ultimately end up with the equation v/u, which is letter A.
- The answer key says the answer is D. Why? What am I failing to include?
I'm including a picture of my attempt to work it out, just in case no one understood what I wrote above.