Acute Triangle ABC: Proving Perpendicular Lines AD and EF

[anemone]

Here is this week's POTW:

-----

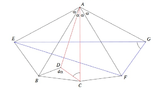
Acute triangle $ABC$ has $\angle BAC <45^\circ$. Point $D$ lies in the interior of triangle $ABC$ such that $BD=CD$ and $\angle BDC=4\angle BAC$. Point $E$ is the reflection of $C$ across line $AB$, and point $F$ is the reflection of $B$ across line $AC$. Prove that lines $AD$ and $EF$ are perpendicular.

-----

Remember to read the http://www.mathhelpboards.com/showthread.php?772-Problem-of-the-Week-%28POTW%29-Procedure-and-Guidelines to find out how to http://www.mathhelpboards.com/forms.php?do=form&fid=2!

 

[anemone]

No one answered last week's problem.(Sadface)

You can find the suggested brilliancy award-winning solution by Evan O'Dorney below:

Spoiler

We begin by reflecting $C$ over $AF$ to point $G$, as shown in the diagram:

View attachment 6596

As usual we denote $\alpha=\angle BAC,\,\beta=\angle ABC$ and $gamma=\angle ACB$.

In order to prove that $AD=\perp EF$, we will show that $\triangle ADC\sim \triangle EFG$ and that $AC\perp EG$.

To begin with the easier part, by reflection, the four angles marked $\alpha$ are congruent, and $AE=AC=AG$. Thus $AC$ is the angle bisector of isosceles triangle $AEG$ and therefore $AC \perp EG$.

Next, to take the first step towards showing $\triangle ADC\sim \triangle EFG$, we see that $BD=CD$ and $\angle BDC=4\alpha$, so triangles $AEG$ and $BDC$ are similar isosceles triangles with base angle $90^\circ-2\alpha$.

Furthermore, $\angle AGF=\angle ACF =\angle ACB=\gamma$ by reflection.

Combining these facts, we have $\angle DCB=90^\circ -2\alpha$, and thus $\angle ACD= \gamma -(90^\circ-2\alpha)=\angle EGF$.

Again, by using the similar triangles $EAG$ and $BDC$, we have

$\dfrac{AG}{EG}=\dfrac{DC}{BC}$

and because of the reflection $\triangle ABC\cong \triangle AFG$, we have

$\dfrac{FG}{AG}=\dfrac{BC}{AC}$

Multiplying these two equations gives $\dfrac{FG}{EG}=\dfrac{DC}{AC}$.

Consequently, $\triangle ADC \sim \triangle EFG$ by SAS similarity.

Since $AG\perp EG$ and both $\angle DAC$ and $\angle FEG$ are oriented in the same direction, the transformation that takes $\triangle ADC$ to $\triangle EFG$ is a $90^\circ$ rotation, combined with some dilations and/or translations. This transformation also takes $AD$ to $EF$, which implies that these lines are perpendicular.