Addition of Reluctances in Parallel [MAGNETISM]

[Ghost101]

Homework Statement

Hey guys,

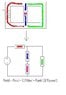
I have an incredibly broad question, just revising the topic of Magnetism,

On the topic of reluctance,
As I have learned so far, Magnetic Circuits and electric circuits have similar properties.

When the core is a "series" network, you add Reluctance together,

R(t) = R1 + R2 + ... etc

when the core is split into more than one "loop" do we still add reluctance like we do in series?

Thanks in advance.

My logic would say that the rules of parallel addition of resistors doesn't apply for a core.

 

[Zryn]

Reluctance works the same as Resistance in both series and parallel.