All about Earth's Gravity - Comments

[klotza]

klotza submitted a new PF Insights post

All about Earth's Gravity

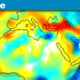
Continue reading the Original PF Insights Post.

 

[Orodruin]

Nice Insight, but I am afraid the $1-3\cos^2\theta$ might baffle anyone not familiar with Legendre polynomials and spherical harmonics. The text may be read as the gravitational field being proportional to this, which it is clearly not since it becomes negative. The next question popping up will then be if we are talking about the lattitude dependence only, why write out the constant term. For the layman, it may be more straightforward to simply say that there is a part varying proportional to $-\cos(2\theta)$.

 

[klotza]

That is a good point. I will consider a better way to describe that.

 

[phinds]

Fascinating Insight. Thanks.

 

[Borek]

"wthahat" is a funny typo.

 

[Jonathan Scott]

... differences in the passage of time due to the differing gravitational fields ...

... However, it is in a weaker gravitational field, so you might expect it to tick faster.

Both of these statements appear to be based on a common misconception. The rate of a clock does not depend on the gravitational field, but rather on the gravitational potential. Although the field is weaker at a higher potential when relating to a single central source, it is the difference in potential rather than the strength of the field which determines the time dilation. This problem seems to be limited to one paragraph, in that the following paragraphs correctly refer to the potential as determining the time dilation.

 

[nikkkom]

typo

"the gravitational field actually increases as look deeper into the Earth"

you meant "as WE look deeper..."?

 

[A.T.]

Thanks for the post.

"The geoid is the hypothetical shape that the Earth would take if its surface were completely covered in ocean, such that the gravitational and centrifugal potential is the same at every point on the surface."

Maybe this would be clearer:

"..., such that the sum of gravitational and centrifugal potential is the same at every point on the surface."

 

[jerromyjon]

Very informative and a certainly useful set of data to add to the noggin!

potential as determining the time dilation.

That exact point caught me off guard the day prior to this insight, I believe it was, regarding dilation of the ISS at a higher potential than the average surface of the Earth. (Zero on the gravity anomaly chart?) Being on the ISS you are moving at a faster relative velocity so clocks tick slower and being at a higher potential (and therefore less acceleration) clocks tick faster but of less magnitude than the velocity contribution. It may be the other way around? I'm not sure. This is where I need to clarify details and likely ask questions in a topic of a new thread...

 

[A.T.]

higher potential (and therefore less acceleration)

Note that higher potential doesn't always imply less gravitational acceleration. That's why it's important to understand that gravitational time dilation depends on the potential, not the gravitational acceleration.

 

[jerromyjon]

That's why it's important to understand that gravitational time dilation depends on the potential, not the gravitational acceleration.

That's the direction I was heading into the shell theorem where the deeper inside the Earth you travel the effects would be opposite? Less acceleration AND lower potential?

 

[Greg Bernhardt]

Great article as usual Alex!

 

[Drakkith]

Great article! Very interesting!

 

[Jonathan Scott]

After the earlier improvement, there's now a reference to a "weaker" potential which is not really meaningful; I'd say "higher" potential in this context.

 

[M Quack]

Thank for this nice article.

That is a good point. I will consider a better way to describe that.

It might be clearer to explicitly say "it deviates from its average value by (1-3 cos^2 theta)". The deviation has to average out to zero, by definition, and therefore has to be part negative, part positive.

This being a physics forum, stating the field in terms of spherical harmonics is perfectly ok, imho. Again, explicitly stating that (1-3 cos^2 theta) is the leading term in the deviation from the average might help.

 

[JorisL]

Great insight, really enjoyed it.

This being a physics forum, stating the field in terms of spherical harmonics is perfectly ok, imho. Again, explicitly stating that (1-3 cos^2 theta) is the leading term in the deviation from the average might help.

This makes it harder for high school students (for example).
So it all depends on the target of the insights (e.g. for the layperson or for the undergrad physics major)

 

[QuantumQuest]

A really good insight, thanks Alex!

 

[Wayan Dadang]

Great article! Thanks.