Alternate form of Principle of superposition

[member 731016]

Homework Statement

I am trying to reword my textbook definition of the principle of superposition in terms of propositional logic

Relevant Equations

$$L[y] = y^{''} + p(t)y^{'} + q(t)y = 0$$

The definition is,

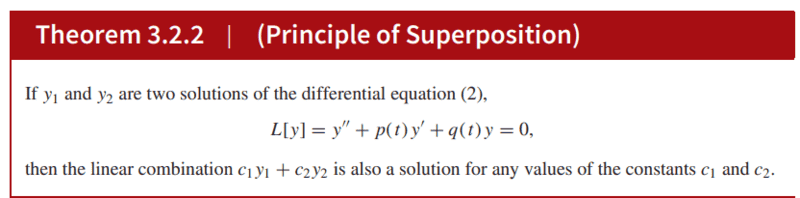
I rewrite it as $$(L[y_1] = L[y_2] = 0) \rightarrow (L[c_1y_1 + c_2y_2] = 0)$$.

However, I also wonder, whether it could also be rewritten as,

$$(L[c_1y_1 + c_2y_2] = 0) \rightarrow (L[y_1] = L[y_2] = 0) $$

And thus, combining, the two cases,

Principle of superposition. $$(L[c_1y_1 + c_2y_2] = 0) ↔ (L[y_1] = L[y_2] = 0)$$

Is my reasoning correct please?

Thanks for any help!

 

[Orodruin]

As long as you include the statement about ”any coefficients $c_1$ and $c_2$” it is obvious that if $L[c_1y_1 + c_2y_2] = 0$ then each of the ys is a solution.

 

[member 731016]

As long as you include the statement about ”any coefficients $c_1$ and $c_2$” it is obvious that if $L[c_1y_1 + c_2y_2] = 0$ then each of the ys is a solution.

Thank you for your reply @Orodruin!

Yes that is a good idea to quantify my statement with ∀ to give

$$(L[c_1y_1 + c_2y_2] = 0) ↔ (L[y_1] = L[y_2] = 0)$$ $$∀c_1, c_2 ∈ \mathbb{R}$$


Thanks!