- #1
samsterC
- 17
- 0
If wind was blowing through a tube at 15 kilometers an hour from an open end 2 meters wide to a tapered end only .3 meters wide how fast would the air be moving when it exits the pipe?
I'm using the equation Q = v * A (Q is flow, v is velocity and A is area of the tube opening) but is that the proper way to do it? Or should I be using a different equation?
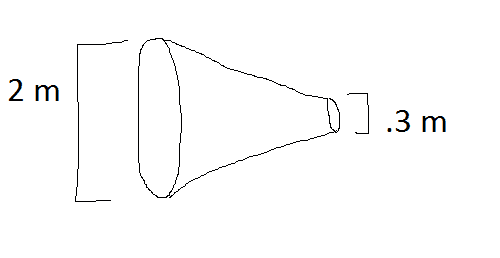
I'm using the equation Q = v * A (Q is flow, v is velocity and A is area of the tube opening) but is that the proper way to do it? Or should I be using a different equation?