An apparently tricky problem on Friction/Forces.

[cantgetaname]

Homework Statement

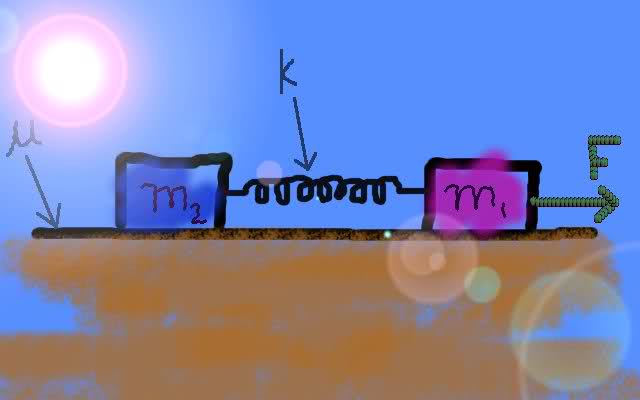
Two bodies of mass m₁ and m₂ are placed on a horizontal table with coefficient of friction $$\mu$$ and are joined by a spring. Initially, the spring has its natural length.
If F is the minimum force which, when applied on m₁, will make the other block m₂ just move, find F. (k is the spring constant)

Here is an artistic illustration of the situation.

Homework Equations

1. Limiting Frictional Force = $$\mu$$ x Normal/reaction offered by the surface.
   
2. Force due to spring = k * Elongation of spring (from its natural length)

The Attempt at a Solution

If m₂ is to move, it needs to experience a force of $$\mu m_{2}g$$ (to oppose the friction), which can be only experienced from the spring.
This means the spring's elongation at that instant is atleast $$\frac{\mu m_{2}g}{k}$$.
An equal and opposite force acts on m₁. For m₁ to move, net force on it must be greater than $$\mu m_{1}g$$.
That gives $$F=\mu m_{1}g+\mu m_{2}g$$, which is incorrect.

The answer is $$F=\mu m_{1}g+\frac{\mu m_{2}g}{2}$$, which I have no idea of where it could possibly come from.

 

[fuzzywolf]

Does the spring act only on m2?

 

[cantgetaname]

Well, it obviously acts on both.
It can't possibly act on just one object.

 

[fuzzywolf]

That is precisely correct. F = -kx assumes that one end of the spring is fixed, which is not true in this case. Try a special coordinate system where one direction is toward the center of the spring, and one direction is away from the center of the spring.

 

[Doc Al]

If m₂ is to move, it needs to experience a force of $$\mu m_{2}g$$ (to oppose the friction), which can be only experienced from the spring.
This means the spring's elongation at that instant is atleast $$\frac{\mu m_{2}g}{k}$$.

So far, so good!

An equal and opposite force acts on m₁. For m₁ to move, net force on it must be greater than $$\mu m_{1}g$$.

That's certainly true.

That gives $$F=\mu m_{1}g+\mu m_{2}g$$, which is incorrect.


The answer is $$F=\mu m_{1}g+\frac{\mu m_{2}g}{2}$$, which I have no idea of where it could possibly come from.

Hint: Realize that the applied force just has to be enough to get m1 moving and stretch the spring the right amount. Think in terms of energy.

 

[ehild]

So far, so good!

Hint: Realize that the applied force just has to be enough to get m1 moving and stretch the spring the right amount. Think in terms of energy.

No, the problems asks the force which makes m2 just move. I do not see why the minimal force is not (m1+m2)g .


ehild

 

[Doc Al]

No, the problems asks the force which makes m2 just move. I do not see why the minimal force is not (m1+m2)g .

I assume you meant μ(m1+m2)g.

Ask yourself: If you applied such a force through a distance x (the required elongation), how much energy will m1 have? If m1 has extra energy beyond that needed to stretch the spring, then you've pushed too hard. Note that the spring force is not constant.

 

[ehild]

Sorry, i meant ug(m₁+m₂). Does the problem ask the minimum constant force? If so, it is really
ug(m₁+m₂/2), and anyway, it is less than ug(m₁+m₂), so you are right...

So we apply a constant force F along a path Δx, first accelerating m₁, than letting it decelerate, stopping at the end. According to the Work-Energy Theorem, the work of all forces equals to the change of KE which is zero now. So
W=(F-μm₁g)Δx-kΔx²/2 =0, F=μm₁g+kΔx/2

ehild