- #1
Guillem_dlc
- 188
- 17
- Homework Statement
- Find the angle of the principal axes where ##x'## and ##y'## are the principal axes.
- Relevant Equations
- Last figure of the solution
Statement figure:
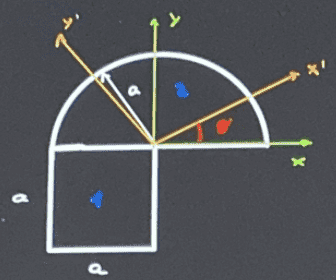
My attempt at a solution:
FIGURE 1 ##\rightarrow A=a^2##

CG ##\rightarrow \overline{x}=-a/2, y=-a/2##
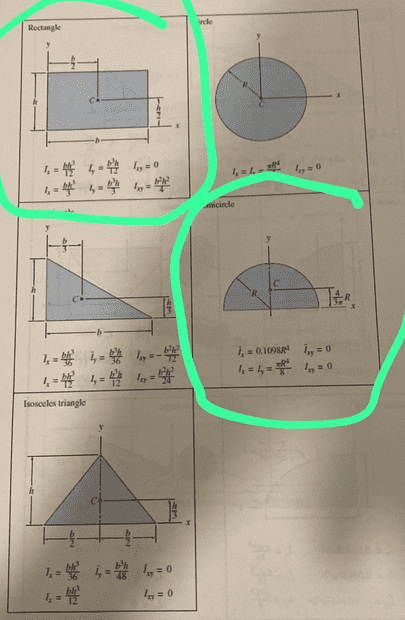
$$\overline{Ix}_1=\dfrac{bh^3}{12}=\overline{Iy}_1=\dfrac{a^4}{12}$$
$$Ix_1=\overline{Ix}_1+\overline{y}^2A=\dfrac{a^4}{12}+\dfrac{a^4}{4}=\dfrac13 a^4\, \textrm{mm}^4=Iy_1$$
$$\overline{Ixy}_1=0\, \textrm{mm}^4\rightarrow Ixy_1=\dfrac{a^4}{4}$$
FIGURE 2 ##\rightarrow A=\dfrac{\pi a^2}{2}##

CG ##\rightarrow \overline{x}=0, \overline{y}=\dfrac{4\pi}3a##
$$Ix_2=Iy_2=\dfrac{\pi}8a^4\, \textrm{mm}^4,\,\, \overline{Ixy}=0\rightarrow Ixy=0+0\cdot \overline{y}A=0\, \textrm{mm}^4$$
$$Ix=\sum Ix_i=0,76032a^4\, \textrm{mm}^4$$
$$Iy=\sum Iy_i=0,76032a^4 \, \textrm{mm}^4$$
$$Ixy=\sum Ixy_i=\dfrac 14a^2$$
$$\boxed{\sigma =\dfrac12 \arctan \left( \dfrac{-2Ixy}{Ix-Iy}\right)=\dfrac12 \arctan (-\infty)=-45^\circ}$$
If you could tell me if it's ok you would do me a big favor. thank you very much!
The moment of inertia depended on how the surface moves away from the axis, right? Because if so maybe it does make sense that I got the two equal moments (##x## and ##y##), doesn't it?
My attempt at a solution:
FIGURE 1 ##\rightarrow A=a^2##
CG ##\rightarrow \overline{x}=-a/2, y=-a/2##
$$\overline{Ix}_1=\dfrac{bh^3}{12}=\overline{Iy}_1=\dfrac{a^4}{12}$$
$$Ix_1=\overline{Ix}_1+\overline{y}^2A=\dfrac{a^4}{12}+\dfrac{a^4}{4}=\dfrac13 a^4\, \textrm{mm}^4=Iy_1$$
$$\overline{Ixy}_1=0\, \textrm{mm}^4\rightarrow Ixy_1=\dfrac{a^4}{4}$$
FIGURE 2 ##\rightarrow A=\dfrac{\pi a^2}{2}##
CG ##\rightarrow \overline{x}=0, \overline{y}=\dfrac{4\pi}3a##
$$Ix_2=Iy_2=\dfrac{\pi}8a^4\, \textrm{mm}^4,\,\, \overline{Ixy}=0\rightarrow Ixy=0+0\cdot \overline{y}A=0\, \textrm{mm}^4$$
$$Ix=\sum Ix_i=0,76032a^4\, \textrm{mm}^4$$
$$Iy=\sum Iy_i=0,76032a^4 \, \textrm{mm}^4$$
$$Ixy=\sum Ixy_i=\dfrac 14a^2$$
$$\boxed{\sigma =\dfrac12 \arctan \left( \dfrac{-2Ixy}{Ix-Iy}\right)=\dfrac12 \arctan (-\infty)=-45^\circ}$$
If you could tell me if it's ok you would do me a big favor. thank you very much!
The moment of inertia depended on how the surface moves away from the axis, right? Because if so maybe it does make sense that I got the two equal moments (##x## and ##y##), doesn't it?